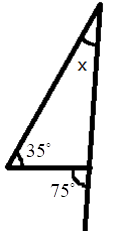
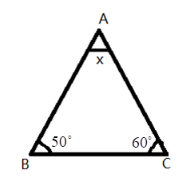
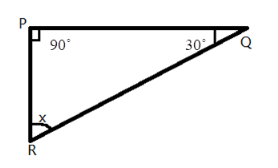
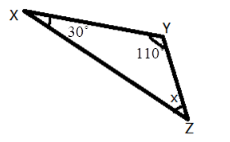
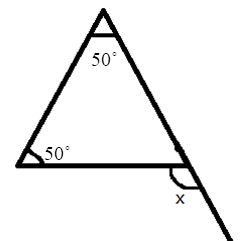NCERT Solutions for Maths Class 7 Chapter 6 The Triangle and its Properties - FREE PDF Download






FAQs on NCERT Solutions for Class 7 Maths Chapter 6 The Triangle and Its Properties
1. What will I learn in Class 7 Maths Chapter 6?
You will get to learn about the specific important properties of triangles, such as
Sum of all interior angles of a triangle.
Types of angles.
Sum of two angles lying on the same plane.
Types of angles based on degrees.
Parallel lines and transversal.
Comparison between interior and exterior angles.
Properties of angles in a triangle.
Sum of vertically opposite angles.
Concept of area and perimeter of any given two-dimensional figure.
Difference between a triangle and a quadrilateral.
Calculation of area and other regular figures and perimeter of both triangles.
Angle sum property of triangles.
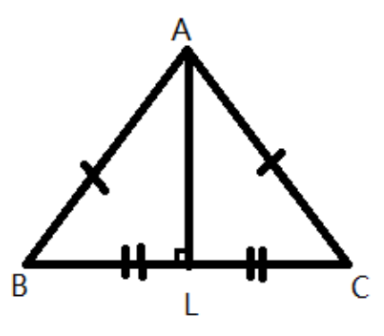
Isosceles and equilateral triangles.
Right-angle triangles.
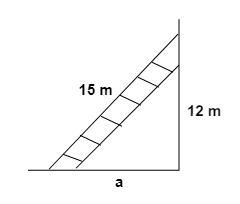
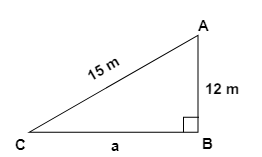
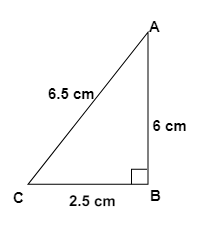
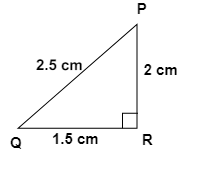
Pythagoras Theorem.
2. Explain the basic properties of the triangles.
While solving the figure-based problems, you need to know the below-given points:
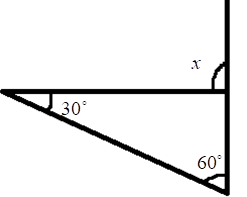
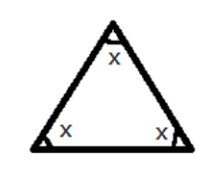
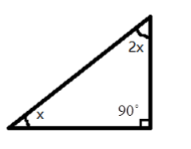
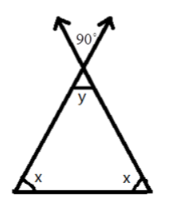
The summation of all the angles in a triangle equals 180°.
The difference between any two sides of a triangle is less than the length of its third side.
The summation of the length of any two sides of a triangle is greater than the length of its third side.
The side of a triangle that is opposite to the greater angle is the longest side of the triangle.
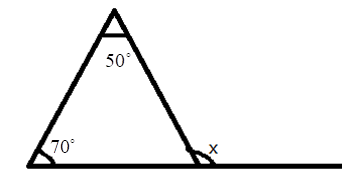
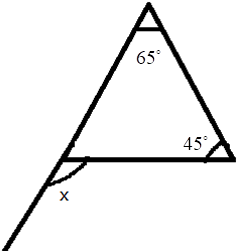
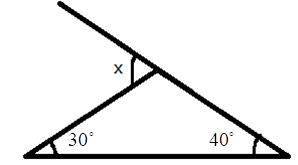
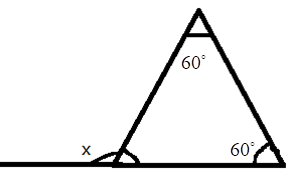
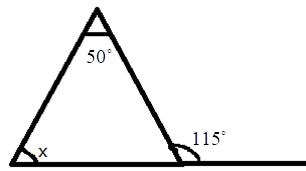
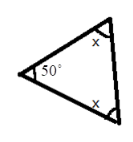
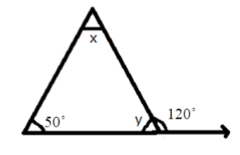
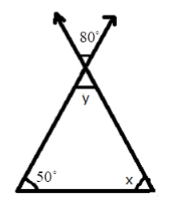
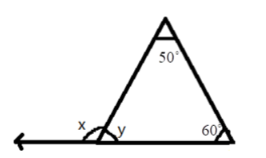
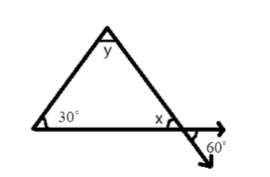
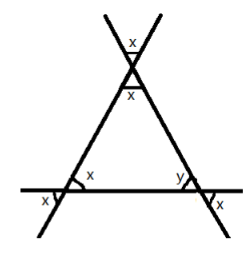
The exterior angle property – herein, the exterior angle property of a triangle is equal to the summation of the interior opposite angles.
If the corresponding angles of two triangles are congruent and the lengths of their respective sides are proportional, the two triangles are similar.
The perimeter of a triangle equals the summation of all its three sides.
The area of a triangle equals (½ x base X height).
3. How many exercises and questions are there in Chapter 6 of Class 7 Maths?
Class 7 Maths Chapter 6 consists of 6 exercises:
Exercise 6.1 consists of 3 questions. This exercise mainly deals with Isosceles and equilateral triangles.
Exercise 6.2 consists of 2 questions. This exercise focuses on interior and exterior angles, properties of angles in a triangle and the sum of all interior angles of a triangle.
Exercise 6.3 consists of 2 questions. This exercise deals with the Isosceles triangle, equilateral triangles, Right-angled triangles and Pythagoras property.
Exercise 6.4 consists of 6 questions. This exercise comprises questions related to the sum of vertically opposite angles, parallel lines and transversal.
Exercise 6.5 consists of 8 questions. These particular exercise questions mainly focus on the Pythagoras Theorem, a triangle and a quadrilateral.
4. How to reduce the fear of Maths with NCERT Solutions by Vedantu?
Maths is a subject which is always a fear for many students. It is seen as a phobia among the majority of students. This is because they have never seen this subject in the way it actually had to be seen. The best way to get rid of this phobia is to take a smart forward step.
Our NCERT Solutions for Class 7 Maths Chapter 6 are one of the most essential study materials for class 7 students. Our expert teachers have framed these solutions with the utmost care in a bit-by-bit manner to make their preparation process for the exam way easier.
5. What is the Pythagorean theorem in class 7 maths chapter 6 PDF solutions?
In class 7 maths chapter 6 PDF solutions the Pythagorean theorem is a fundamental principle in geometry. It states that in a right-angled triangle, the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. This theorem helps in calculating distances and is widely used in various mathematical applications.
6. How does the triangle inequality theorem help in understanding class 7 maths chapter triangle and its properties?
The triangle inequality theorem is a crucial concept in geometry. It states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side. In class 7 maths chapter triangle and its properties, this theorem is essential for determining the possible dimensions of a triangle and understanding the properties of triangle formation.
7. What are the criteria for the congruence of triangles in class 7 ch 6 maths?
In class 7 ch 6 maths triangles are congruent if they have the same shape and size. The criteria for congruence include Side-Side-Side (SSS), where all three sides are equal; Side-Angle-Side (SAS), where two sides and the included angle are equal; Angle-Side-Angle (ASA), where two angles and the included side are equal; and Right-angle-Hypotenuse-Side (RHS) for right-angled triangles.
8. Why is it important to practice the exercises in class 7th chapter 6?
Practising the class 7th chapter 6 is essential for mastering the concepts of triangle properties and theorems. It reinforces students' understanding and helps them apply these principles to solve problems. Regular practice ensures that students are well-prepared for exams and can tackle questions related to triangles confidently.