NCERT Solutions for Maths Chapter 10 Algebraic Expressions Class 7 - FREE PDF Download
FAQs on NCERT Solutions for Class 7 Maths Chapter 10 Algebraic Expressions
1. Choose the Correct Answer and Fill in the Blanks.
(2x – 7) is a _______________________ [ binomial / monomial ]
5x is a ____________________. [binomial/ monomial ]
3xyz is a ___________________. [ monomial/ trinomial]
A term of an expression having no literal factor is called a ______________ term.
An algebraic expression having only one term is called a _______________.
In –x, the coefficient of x is ________________.
- binomial
- monomial
- monomial
- constant
- monomial
- -1
2. Answer the Following Questions.
What do we call an expression having only one term?
What is the coefficient of x3 in –x3?
What is the power of -3x3y?
What is the value of -5x2 at x = -1?
- monomial
- – 1
- 4
- – 5
3. How are Algebraic Expressions Formed? Illustrate with an Example.
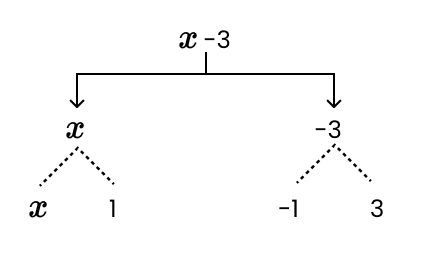
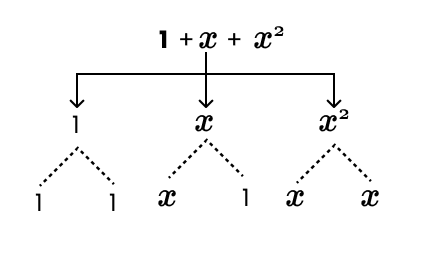
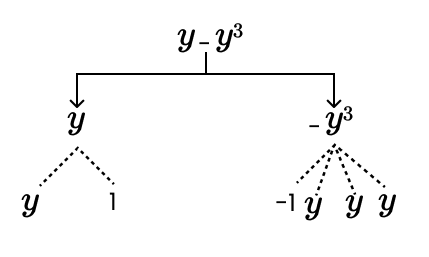
An algebraic expression is formed by a combination of constants and variables, connected by the symbols +, —, x, and ÷. We can also obtain algebraic expressions by combining variables with themselves or with other variables.
E.g.: The expression x3 is obtained by multiplying the variable x by itself.
x* x * x = x3
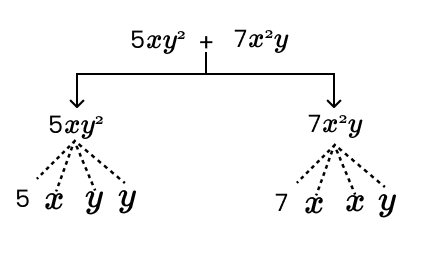
4. What are Like and Unlike Terms? Illustrate with an Example.
The terms having the same literal factors are called like terms (or similar terms) whereas the terms that do not have the same literal factors are called unlike terms (or dissimilar terms).
Example: In the expression 5x2y + 3xy2 – xy -2xy2, we have 5x2y and –2yx2 are like terms.
The terms 3xy2 and – xy are unlike terms.
5. How Does Vedantu Help Score Well in Exams?
Vedantu provides a detailed study of the concepts and theories covered in all subjects along with NCERT Solutions for every subject. You can download the PDF files of the study materials available on the Vedantu and study at your convenient time. Vedantu has a team of experts and you can always connect with them to clarify your doubts. You can also register online for NCERT Solutions for class 7 science and attend the live classes on Vedantu to score good marks in your exams.
6. How do you identify like terms in an algebraic expression in algebraic expressions class 7?
In algebraic expressions class 7 like terms in an algebraic expression are terms that have the same variables raised to the same powers, even if their coefficients are different. For example, in the expression 3x+5y−2x+7y, the like terms are 3x and −2x as well as 5y and 7y. To combine like terms, you simply add or subtract their coefficients while keeping the variable part unchanged.
7. Do I need to practice all questions provided in NCERT Solutions Class 7 Maths Algebraic Expressions?
Yes, practising all the questions provided in NCERT Solutions for Class 7 Maths in Algebraic Expressions is necessary. This will help you answer well in your exams as you will be able to understand the topics clearly. The solutions provided by Vedantu are free of cost. They are also available on the Vedantu Mobile app.
8. Why are NCERT Solutions class 7 chapter 10 important?
NCERT Solutions for Class 7 Chapter 10 is important because it provides you with all the answers to the exercises of the chapter that are given in your NCERT Maths textbook.
9. What are Algebraic Expressions for Class 7?
Algebraic Expressions are a combination of numbers, letters, and arithmetic operations. You will learn about algebra and its basics in class 6 itself. There you will get an idea as to what an algebraic expression looks like. In Class 7, you will be able to identify an algebra expression when you see them.
10. What is an Algebra formula?
Formulae in algebra are used to solve algebraic expressions and every other problem related to algebra. The formulas in algebra are the basic foundation of how you can learn algebra. To know more about formulas, refer to your textbook and reference book for class 7 NCERT Maths.