NCERT Solutions for Class 11 Maths Chapter 9 Straight Lines Exercise 9.1 - FREE PDF Download
FAQs on NCERT Solutions for Class 11 Maths Chapter 9 Straight Lines Ex 9.1
1. What is meant by the slope of a line?
The slope of a line is the measure of its steepness. In coordinate geometry, the letter m is used to denote the slope of a line. The direction of a line can be determined by its slope. Also, it is a measure of the change in the y coordinate for every unit change in the x coordinate, along the straight line. There are several formulas to find the slope of a given line. There are four types of slopes, namely, zero, positive, negative, and undefined. The basic formula for calculating the slope of a line is m = Δy/Δx, where Δy represents the change in the y coordinate and Δx represents the change in the x coordinate.
2. How to determine the slope of a straight line?
There are three forms of equations that can be used to find the slope of straight lines. These are standard form, point-slope form, and slope-intercept form.
Slope - Intercept Form: The slope m is used in the representation of the standard equation of a straight line, which is, y = mx + c. This type of equation is called the slope-intercept equation, wherein, ‘c’ is the y-intercept of the straight line.
Point - Slope Form: The general form of equation used to define the slope of a straight line is, m = Δy/Δx. This equation can be elaborated as m = [y - y1]/[x - x1]. This form of the slope equation is known as the point-slope form. In this equation, (x1,y1) represents any point on the given straight line and m is the slope of the straight line.
Standard Form: Ax + By = C This is the standard form of the equation that is used to determine the slope of a straight line. In this equation, A, B, and C are constants.
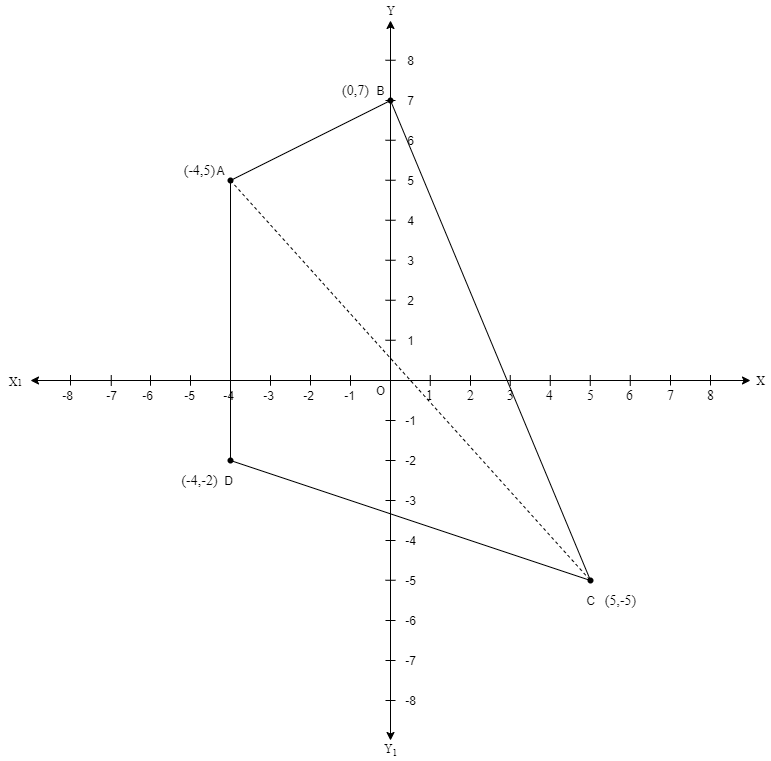
3. How will you show that a group of three given points are the vertices of a right-angled triangle, without using Pythagoras Theorem?
If any two straight lines are perpendicular to each other, then the product of their slopes is always equal to -1. For example, if (3,5), (4,4) and (-1,-1) are the vertices of a right-angled triangle, then the product of the slopes of any of the two straight lines formed with these points will be -1. Here, the straight line formed between the points (4,4) and (3,5) is -1, and the slope of the straight line formed between the points (4,4) and (-1,-1) is 1. The product of these two slopes is -1, therefore, these two lines are perpendicular to each other. The triangle formed by (3,5), (4,4), and (-1,-1) is right-angled at (4,4).
4. Why should you refer to the NCERT Solutions for Class 11 Maths Chapter 10 Straight Lines (Ex 10.1) Exercise 10.1?
Practicing all the sums from the Class 11 NCERT maths book is an important part of the preparation for your Maths exam. These NCERT solutions available on Vedantu are prepared by our subject matter experts in a stepwise manner. You can download the PDF of these NCERT solutions for free and refer to it for clearing your doubts. Chapter 10 of NCERT Class 11 Maths covers various concepts of Straight Lines. The topics included in this chapter are,
Introduction to Straight Lines
The Slope of a Line
Collinearity of three points
To find the slope of a line when the coordinates of any two points lying on the line are given
The angle between two lines
Slopes of parallel lines
Slopes of perpendicular lines
5. Find the value of x for which the points (x, – 1), (2, 1), and (4, 5) are collinear, according to Exercise 10.1 of Chapter 10 of Class 11 Maths?
The slope of AB = Slope of BC if the points (x, – 1), (2, 1), and (4, 5) are collinear.
Then (1+1)/(2-x) = (5-1)/(4-2)
2/(2-x) = 4/2
2/(2-x) = 2
2 = 2(2-x)
2 = 4 – 2x
2x = 4 – 2
2x = 2
x = 2/2
= 1
6. What is the benefit of Vedantu’s solutions for Exercise 10.1 of Chapter 10 of Class 11 Maths?
Vedantu's in-house professionals have meticulously addressed the exercise's problems/questions while adhering to all CBSE requirements. Students of Class 11 who are thoroughly familiar with all of the topics presented in the Subject Straight Lines textbook and all of the problems presented in the exercises can easily achieve the best possible score on the final exam. Students may readily grasp the pattern of questions asked in the test from this Chapter with the help of NCERT Solutions for Exercise 10.1 of Chapter 10 of Class 11 Maths and the Chapter's marks weightage for them to be prepared for the end-term exam. These solutions are available free of cost on the Vedantu website.
7. What all things are included in Exercise 10.1 of Chapter 10 of Class 11 Maths?
Students should measure geometrical shapes and compute their area or perimeter using measures like side lengths and angles formed by neighboring sides in NCERT Solutions for Exercise 10.1 of Chapter 10 of Class 11 Maths. This activity provides us with a new tool for learning and measuring geometrical forms through the questions. Coordinate geometry is this new tool. As a result, rather than having the length of sides measured, we now have the geographic coordinates of a particular point about a fixed point termed the 'Origin.'
8. How can students solve Exercise 10.1 of Chapter 10 of Class 11 Maths?
NCERT's recommendations Exercise 10.1 of Chapter 10 of Class 11 Maths Straight Lines is about navigating the coordinate geometry environment. The essential pillars of coordinate geometry are the two perpendicular axes known as the X and Y axes. To better understand the difficulties, pupils need to have a good sense of direction. There are also important considerations about determining the slope of a line in this exercise. The tasks can ask youngsters to determine if two lines are perpendicular or parallel to one another and the angle between two intersecting lines, using this method.
9. How can students find a slope in Exercise 10.1 of Chapter 10 of Class 11 Maths?
NCERT's recommendations Exercise 10.1 of Chapter 10 of Class 11 Maths encourages students to juggle several inputs of knowledge regarding a point plotted between the X and Y axes. Because this assignment involves the usage of numerous formulas, students should create a formula chart to help them understand the concepts. After completing these problems correctly, students will calculate the slope of a straight line and interpret the results graphically.
10. What is the significance of the y-intercept in the slope-intercept form of a line?
As we studied in Class 11 Ex 9.1 NCERT Solutions, The y-intercept c in the slope-intercept form y=mx+c represents the point where the line crosses the y-axis. It is the value of y when x is 0.
11. How can you determine if two lines are parallel answer it according to class 11 maths ex 9.1.
Two lines are parallel if they have the same slope. That is, if the slopes of the two lines are equal, the lines are parallel.