Question

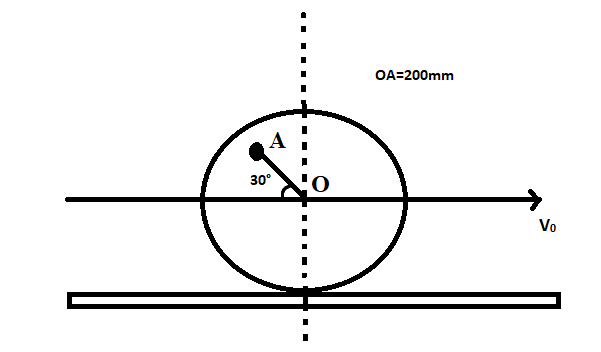
The wheel radius ${\text{ }}r = 300mm{\text{ }}$ rolls to right without slipping and has a velocity ${\text{ }}{v_0} = 3m/s{\text{ }}$ of its center ${\text{ }}O{\text{ }}$. The speed of the point ${\text{ }}A{\text{ }}$ on the wheel for the instant represented in the figure is:

A) $4.36{\text{ m/s}}$
B) $5{\text{ m/s}}$
C) $3{\text{ m/s}}$
D) $1.5{\text{ m/s}}$

Answer 1

Hint: In this question, we have to use the analytical method to find the velocity at the point${\text{ }}A{\text{ }}$. In the analytical method, we use vectors and calculate the speed at a particular instant. We use the parallelogram method to analyze the vector. Velocity is a vector quantity and can be calculated using the vector analysis method.

Formula used:
$R = \sqrt {{A^2} + {B^2} + 2AB\cos \alpha } $
Where ${\text{ }}A{\text{ }}$and ${\text{ }}B{\text{ }}$ are two vectors inclined to each other at a particular angle.
$\alpha {\text{ }}$ is the angle between the two vectors.
$R$ is the resultant vector of the two vectors ${\text{ }}A{\text{ }}$and${\text{ }}B$
$v = r\omega $ (where${\text{ v }}$ is the velocity,${\text{ r }}$ is the radius and ${\text{ }}\omega {\text{ }}$ is the angular velocity)

Complete step by step solution:

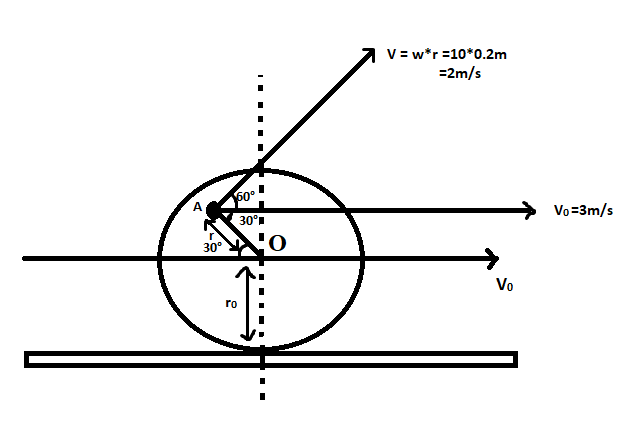
The wheel is in a uniform circular motion. It is rolling with a velocity ${\text{ }}{v_0} = 3m/s$.
We know that the linear speed of an object moving in a circular motion is ${\text{ }}{v_0} = r{\omega _0}$
Where ${\text{ }}r{\text{ }}$ is the radius of the wheel and ${\text{ }}{\omega _0}{\text{ }}$is the angular velocity
We can find the angular velocity of the from the linear velocity as,
$\omega = \dfrac{{{v_0}}}{r}$$ = \dfrac{3}{{300 \times {{10}^{ - 3}}}}$ (Given${\text{ }}{v_0} = 3m/s,r = 300 \times {10^{ - 3}}$)
$\omega = 10{\text{ }} rad$
Given ${\text{ }}OA = {\omega _A} = 200mm = 200 \times {10^{ - 3}}m$
The velocity at a point ${\text{ }}A{\text{ }}$ is
$v = r{\omega _A}$=$200 \times {10^{ - 3}} \times 10 = 2m/s$
The total velocity at the instant is can be obtained by using the formula,
$R = \sqrt {{A^2} + {B^2} + 2AB\cos \alpha } $……………………………(1)
Here, ${\text{ }}A \Rightarrow v,B \Rightarrow {v_0}{\text{ }}$ and the angle between ${\text{ }}v{\text{ }}$ and ${\text{ }}{v_0}{\text{ }}$ is${\text{ }}{60^ \circ }{\text{ }}$ that is obtained from the figure.
The net velocity ${\text{ }}{v_{net}}{\text{ }}$ can be obtained by substituting the values in the equation $(1)$
${v_{net}} = \sqrt {{v^2} + {v_0}^2 + 2v{v_0}\cos \theta } $
${v_{net}} = \sqrt {{2^2} + {3^2} + 2 \times 2 \times 3 \times \cos {{60}^ \circ }} $
Calculating the values,
${v_{net}} = \sqrt {4 + 9 + 12 \times \dfrac{1}{2}} $
${v_{net}} = \sqrt {4 + 9 + 6} = \sqrt {19} = 4.36{\text{ m/s}}$

The answer is Option (A):${\text{ }}4.36{\text{ m/s }}$.

Note: An object is in a uniform circular motion if it moves along the circumference of a circle with constant speed. The right-hand grip rule states that if the curvature of the fingers of the right hand represents the sense of rotation then the thumb represents the direction of the angular displacement vector. Angular velocity is defined as the time rate of angular displacement.