NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements - FREE PDF Download






FAQs on NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements
1. What are the topics that are covered in class 11 physics chapter 2?
The concepts that are covered in chapter 2 are:
Introduction to units and measurement.
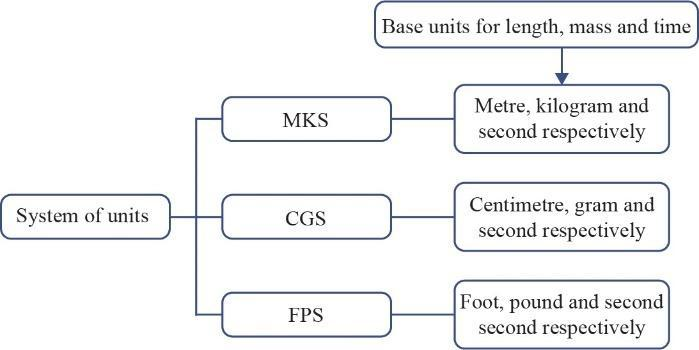
The international system of the units.
SI unit
SI Base unit
SI Derived unit
Advantages of learning SI units and CGS units
Significant figures.
Applications of significant figures
Exact Number
Dimensions of physical quantities.
Dimensional formulae and dimensional equations.
Dimensional analysis and its applications.
Unit conversion and dimensional analysis
Using dimensional analysis to check the correctness of physical equation.
Homogeneity principle of dimensional analysis.
Applications of dimensional analysis.
Limitations of dimensional analysis.
2. What is dimensional analysis?
We quantify the size and shape of things using Dimensional Analysis. It helps us study the nature of objects mathematically. It involves lengths and angles as well as geometrical properties such as flatness and straightness. The basic concept of dimension is that we can add and subtract only those quantities that have the same dimensions. Similarly, two physical quantities are equal if they have the same dimensions.
The main benefits of the dimensional analysis of a problem have reduced the number of variables in the issue by combining dimensional variables to one form of non-dimensional parameters. By far the easiest and most useful method in the analysis of any fluid problem is that of direct mathematical solution.
3. What are gross errors?
This section basically takes into account human oversight and other mistakes while reading, recording and readings. The most common errors, the human error in the measurement fall which is under this category of errors in measurement. For instance, a person taking the reading from the meter of the instrument and he may read 33 as 38. Gross errors could be avoided by using two suitable measures and they are mentioned below:
A proper care should always be taken while reading and recording the data. Also, calculation of error should be done accurately.
By increasing the total number of experimenters we can reduce the gross errors. If each experimenter takes different reading at various points, then by taking an average of more readings we can reduce the gross errors.
4. How Vedantu will help me in exam preparation?
Our NCERT solutions are prepared by our maths experts with various real-life examples. These examples will make you understand the concept quickly and memorise them for a longer time. Solutions provided to the questions are 100% accurate in the exercises which are crisp and concise to the point.
Our solutions are the best study guides, which help you in smart learning and efficient answering of questions. These solutions will also help you in improving a strong conceptual base with all the important concepts in a very easy and understandable language. You will also enjoy learning from our solutions which are really fun and interactive.
5. Are NCERT Solutions important for Class 11 Physics Chapter 2?
Referring to NCERT Solutions is as important as referring to the questions while preparing for your Class 11 Physics Chapter 2. Only reading the questions that are provided in the NCERT is not going to be helpful if students are not able to answer them correctly. NCERT Solutions for Class 11 Physics Chapter 2 available on Vedantu provide students the correct step-by-step solutions for all NCERT questions so that students do not lose any marks in their exams.
6. Do I need to practice all the questions provided in Class 11 Physics Chapter 2 NCERT Solutions?
Questions provided in Class 11 Physics NCERT Solutions for Chapter 2 are to be considered crucial when preparing for your Class 11 Physics exams. The exam question papers always include questions that have been provided in the Physics NCERT for Class 11. Practicing all the questions will only help you increase your understanding of all the concepts taught in Chapter 2 and also the possibility of scoring well in the questions framed from the chapter.
7. How can I understand the Class 11 Physics Chapter 2?
Chapter 2 in Class 11 Physics NCERT is called “Units and Measurement”. This chapter talks in detail about various units used for determining the measurement of different physical quantities, instruments used for such measurements and their accuracy, etc. Students can easily understand this chapter by indulging in regular reading of the chapter and solving the questions provided in the NCERT. For more help, students can also refer to NCERT Solutions for Class 11 Physics Chapter 2 on the official website of Vedantu or download the Vedantu app where these resources are available at free of cost.
8. What is the marks distribution for Class 11 Physics Chapter 2?
Chapter 2 - Units and Measurement in Class 11 Physics is a part of Unit - I along with Chapter 1 - Physical World. According to the marks distribution provided by CBSE for Class 11 Physics, Unit - I consisting of both the chapters, carries a total of 23 marks. Hence, preparation from both chapters should be given equal priority to avoid losing any marks in questions framed from them in the exam.
9. What are the important topics covered in NCERT Solutions for Class 11 Physics Chapter 2?
NCERT Solutions for Class 11 Physics Chapter 2 - Units and Measurements includes various topics like the International System of Units, Measurement of length, mass, and time, Application of Significant Figures, etc. Among these, the most important topics from the chapter include SI Units, Absolute Errors, Dimensional Analysis, and Significant Figures. Short-answer and numerical-based questions can also be asked from the topic of evaluating errors during the measurement of quantities.
10. What is the concept of units and measurements in class 11 exercise solutions?
In units and measurements class 11 exercise solutions in Physics Chapter 1 involves quantifying physical quantities in a standardized manner. A unit is a definite magnitude of a quantity, defined and adopted by convention or law, used as a standard for measuring the same kind of quantity. Measurement is the process of determining the size, length, or amount of something, typically using standard units.
11. Which topics are important in units and measurements class 11 NCERT solutions?
Key topics in Physics Class 11 Chapter 1 Exercise Solutions include:
Physical Quantities and Units: Base and derived quantities, SI units, and other systems of units.
Measurement Techniques: Direct and indirect measurement methods.
Significant Figures: Rules for determining significant figures in measurements.
Errors in Measurement: Types of errors (systematic and random) and methods to minimize them.
Dimensional Analysis: Checking the dimensional consistency of equations and converting units.
12. What is the direct method of measurement of length class 11 physics chapter 1 NCERT solutions?
In units and measurements class 11 exercise solutions, the direct method of measurement of length involves using instruments that directly provide the measurement of length, such as rulers, measuring tapes, vernier calipers, and micrometer screw gauges. These tools are used to measure the length of objects by comparing them directly against a standard unit of length.
13. What is the full form of SI unit?
According to physics class 11 chapter 1, the full form of SI unit is "Système International 'Unités", which translates to the International System of Units. It is the modern form of the metric system and the most widely used system of measurement.
14. What are the three main units of measurement in Class 11 Physics Ch 1 NCERT Solutions?
The three main base units and measurements class 11 ncert solutions in the SI system are:
Meter (m) for length
Kilogram (kg) for mass
Second (s) for time
15. What is a measurement of time in units and measurements class 11 solutions?
The measurement of time is the process of quantifying the duration or interval between two events. The standard unit of time in the SI system is the second (s). Time can be measured using various devices such as clocks, stopwatches, and atomic clocks.