
The tension in a string holding a solid block below the surface of a liquid (where
(A)
(B)
(C)
(D)
Answer
153.3k+ views
Hint: Firstly we have to draw a free body diagram showing all the forces in the system in both cases. First, when the system is at rest. Second when the system is moving with acceleration
Formula used:
Buoyant force,
Complete step by step solution: From the question we know that the block is below the surface of the water and assuming the volume of the block as
From the formula of buoyant force,
The equation for the first case where there is no acceleration when the system is at rest.
Free body diagram of the first case,
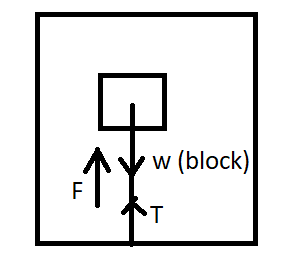
When it is at rest it means the block is at equilibrium with respect to the beaker. This tells us that the net force is zero on the block.
The equation for the second case when the system is moving with acceleration upwards, this causes an increase in buoyant force due to increased displacement of liquid.
Free body diagram in the second case,
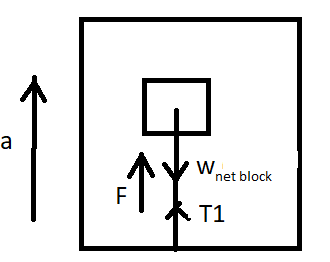
By balancing the forces we get,
Substituting the first equation,
Hence, the correct option is (B),
Note: Always remember that the buoyant force can be derived from the weight of the liquid displaced, which is why the density of the liquid is directly proportional to the buoyant force. Because the system is being accelerated upwards the buoyant force increases, on the other hand, if the system is being accelerated downwards then the buoyant force decreases.
Formula used:
Buoyant force,
Complete step by step solution: From the question we know that the block is below the surface of the water and assuming the volume of the block as
From the formula of buoyant force,
The equation for the first case where there is no acceleration when the system is at rest.
Free body diagram of the first case,

When it is at rest it means the block is at equilibrium with respect to the beaker. This tells us that the net force is zero on the block.
The equation for the second case when the system is moving with acceleration upwards, this causes an increase in buoyant force due to increased displacement of liquid.
Free body diagram in the second case,

By balancing the forces we get,
Substituting the first equation,
Hence, the correct option is (B),
Note: Always remember that the buoyant force can be derived from the weight of the liquid displaced, which is why the density of the liquid is directly proportional to the buoyant force. Because the system is being accelerated upwards the buoyant force increases, on the other hand, if the system is being accelerated downwards then the buoyant force decreases.
Recently Updated Pages
JEE Main 2022 (June 29th Shift 2) Maths Question Paper with Answer Key

JEE Main 2023 (January 25th Shift 1) Maths Question Paper with Answer Key

JEE Main 2022 (July 29th Shift 1) Maths Question Paper with Answer Key

JEE Main 2022 (July 26th Shift 2) Chemistry Question Paper with Answer Key

JEE Main 2022 (June 26th Shift 2) Maths Question Paper with Answer Key

JEE Main 2022 (June 29th Shift 1) Physics Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Degree of Dissociation and Its Formula With Solved Example for JEE

Displacement-Time Graph and Velocity-Time Graph for JEE

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line




