Question

The horizontal and vertical components of the force exerted on the beam at the wall



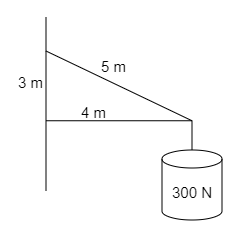
(A) horizontal component is $500\,N$ towards left and vertical component $75\,N$ downwards
(B) horizontal component is $500\,N$ towards right and vertical component $75\,N$ upwards
(C) horizontal component is $625\,N$ towards left and vertical component $150\,N$ upwards
(D) horizontal component is $625\,N$ towards right and vertical component $150\,N$ downwards

Answer 1

Hint: The horizontal and vertical components of the force exerted on the beam at the wall can be determined by tension on the rope. To find the tension of the rope, the angle should be determined, then by using the angle value the tension is determined, then the horizontal and vertical force is determined by using the tension value.

Complete step by step solution:

From the diagram given in the question,
$\sin \theta = \dfrac{3}{5}$
By dividing the terms in the above equation, then
$\sin \theta = 0.6$
By rearranging the terms in the above equation, then
$\theta = {\sin ^{ - 1}}0.6$
Then the angle value is, $\theta = {37^ \circ }$
Now, the tension equation for the given diagram is written as,
$4T \times \sin {37^ \circ } = \left( {150 \times 2} \right) + \left( {300 \times 4} \right)\,................\left( 1 \right)$
At the point of the weight hangs, the vertical tension of the rope is given as $4T\sin {37^ \circ }$
The above equation (1) is the vertical force component equation, the RHS of the equation shows the equation of weight.
By multiplying the terms in the equation (1), then the above equation is written as,
$4T \times \sin {37^ \circ } = 300 + 1200$
The value of the term $\sin {37^ \circ } = 0.6$, then the above equation is written as,
$4T \times 0.6 = 300 + 1200$
By multiplying the terms in the above equation, then the above equation is written as,
$2.4 \times T = 300 + 1200$
By adding the terms in the above equation, then the above equation is written as,
$2.4 \times T = 1500$
By rearranging the terms in the above equation, then the above equation is written as,
$T = \dfrac{{1500}}{{2.4}}$
By dividing the terms in the above equation, then the above equation is written as,
$T = 625\,N$
The horizontal force is given by,
${F_H} = T\cos {37^ \circ }$
By substituting the tension value in the above equation and also the value of the $\cos {37^ \circ } = 0.79863551$, then the above equation is written as,
${F_H} = 625 \times 0.79863551$
By multiplying the terms in the above equation, then the above equation is written as,
${F_H} = 500\,N$
Now, the vertical force is given by,
${F_V} + T\sin {37^ \circ } = 150 + 300$
By substituting the tension value in the above equation and also the value of the $\sin {37^ \circ } = 0.6$, then the above equation is written as,
${F_V} + 625 \times 0.6 = 150 + 300$
By adding the terms in the above equation, then the above equation is written as,
${F_V} + 625 \times 0.6 = 450$
By rearranging the terms in the above equation, then the above equation is written as,
${F_V} = 450 - \left( {625 \times 0.6} \right)$
By dividing the terms in the above equation, then the above equation is written as,
${F_V} = 450 - 375$
By subtracting the terms in the above equation, then the above equation is written as,
${F_V} = 75\,N$

Hence, the option (A) is the correct answer.

Note: The force of the horizontal component is acting on the left side because the tension of the rope pushes the rod towards the left side. And then the weight of the component pulls the rod downwards. The tension of the rope is used to determine the force.