
The circuit shown in the figure contains two diodes each with a forward resistance of
(A) zero
(B)
(C)
(D)
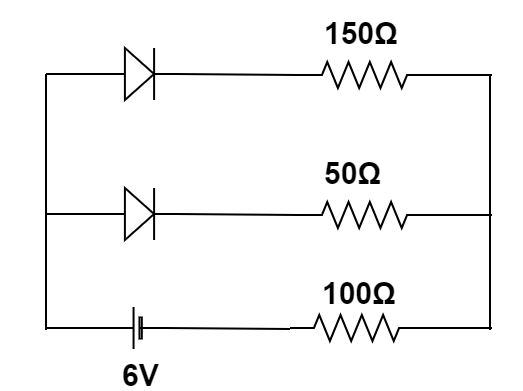
Answer
154.2k+ views
Hint: Knowledge of forward and reverse bias conditions for a PN junction diode is required. Given, the resistance of the diode will be
Formula used:
Complete Step-by-step solution: Let’s remake the circuit, by putting in the signs of the potential of the battery connected, the currents flowing through different arms of the circuit.
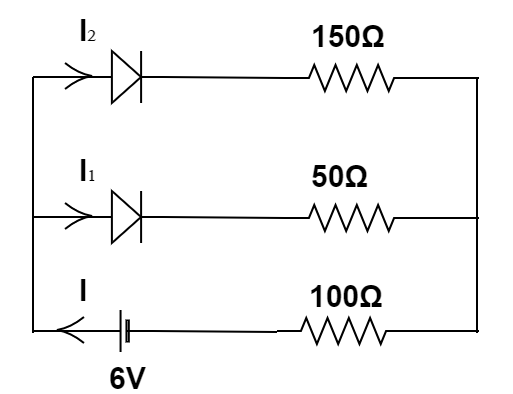
To understand the division of the current flowing through the circuit, we will use Kirchhoff's current law. Hence, considering the amount of current to be flowing through the resistance
Hence, the sum of
We will now discuss the diodes. A PN junction diode is a semiconductor containing excess electrons and holes on each end of the diode and a PN junction interface in between. A common region is formed between the P and the N side having equal amounts of holes and electrons.
In this case, both the diodes are in forward biased condition. Hence the resistance offered by the diode in a forward bias condition is
Taking this into account,
The circuit can be simplified into this form
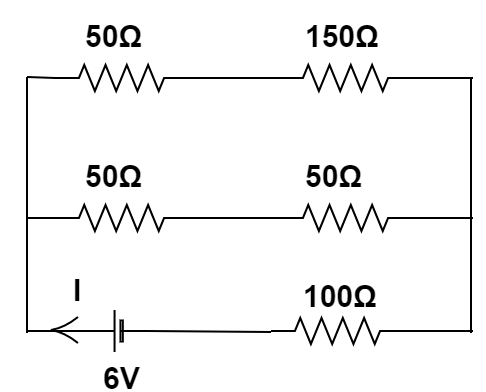
Therefore, we have to find the equivalent resistance of this circuit,
As
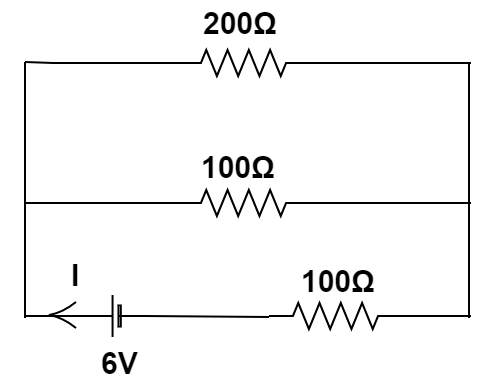
Since
Hence, the circuit can be simplified as;
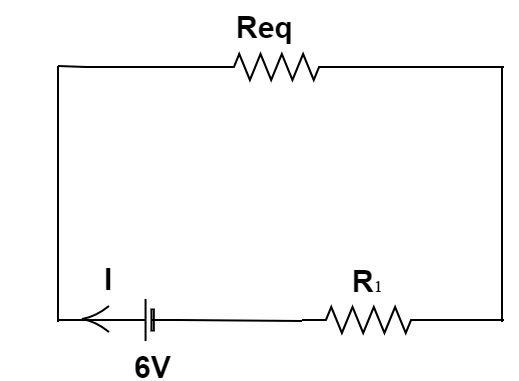
Therefore, the value of current flowing in the circuit can be found using ohm’s law:
On Substituting the value of
Solve the above equation to determine the value of
As a result, the amount of current flowing through the resistor
Hence, the correct option is (D).
Note: we consider the value of the net resistance in the above condition to be:
Formula used:
Complete Step-by-step solution: Let’s remake the circuit, by putting in the signs of the potential of the battery connected, the currents flowing through different arms of the circuit.

To understand the division of the current flowing through the circuit, we will use Kirchhoff's current law. Hence, considering the amount of current to be flowing through the resistance
Hence, the sum of
We will now discuss the diodes. A PN junction diode is a semiconductor containing excess electrons and holes on each end of the diode and a PN junction interface in between. A common region is formed between the P and the N side having equal amounts of holes and electrons.
In this case, both the diodes are in forward biased condition. Hence the resistance offered by the diode in a forward bias condition is
Taking this into account,
The circuit can be simplified into this form

Therefore, we have to find the equivalent resistance of this circuit,
As

Since
Hence, the circuit can be simplified as;

Therefore, the value of current flowing in the circuit can be found using ohm’s law:
On Substituting the value of
Solve the above equation to determine the value of
As a result, the amount of current flowing through the resistor
Hence, the correct option is (D).
Note: we consider the value of the net resistance in the above condition to be:
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Wheatstone Bridge - Working Principle, Formula, Derivation, Application

Young's Double Slit Experiment Step by Step Derivation

JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

Chemical Properties of Hydrogen - Important Concepts for JEE Exam Preparation

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Degree of Dissociation and Its Formula With Solved Example for JEE

Displacement-Time Graph and Velocity-Time Graph for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Electrical Field of Charged Spherical Shell - JEE

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Formula for number of images formed by two plane mirrors class 12 physics JEE_Main




