Question

The block is placed on a frictionless surface in gravity free space. A heavy string of a mass m is connected and force F is applied on the string, then the tension at the middle of rope is:

A.$\dfrac{\left( \dfrac{m}{2}+M \right)F}{m+M}$
B.$\dfrac{\left( \dfrac{M}{2}+m \right)F}{m+M}$
C. zero
D.$\dfrac{MF}{m+M}$

Answer 1

Hint We know that tension is nothing but the drawing force acting on the body when it is hung from objects like chain, cable, string etc. It is represented by T. The direction of tension is the pull which is given the name tension. Thus, the tension will point away from the mass in the direction of the string or rope. In case of the hanging mass, the string pulls it upwards, so the string or rope exerts an upper force on the mass and the tension will be on the upper side. The tension force is the force that is transmitted through a string, rope, cable or wire when it is pulled tight by forces acting from opposite ends. The tension force is directed along the length of the wire and pulls equally on the objects on the opposite ends of the wire. Based on this concept we have to solve this question.

Complete step by step answer
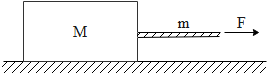
We know that the tension at any point on the rope is basically what force acts on the remaining part.
Now we can say that due to force $F$, the whole system would move with an acceleration of $\mathrm{a}=\dfrac{\mathrm{F}}{\mathrm{M}+\mathrm{m}}$
Then, at the midpoint of the rope the tension should be equal to the value of the force which would be able to pull the left part of the rope and the block with acceleration a.
Hence the conclusion can be drawn as:
$\therefore \mathrm{T}=\left(\mathrm{M}+\dfrac{\mathrm{m}}{2}\right) \dfrac{\mathrm{F}}{\mathrm{M}+\mathrm{m}}$

Hence, we can say that the correct answer is option A.

Note: We should know that the weight of an object may be thought of as acting at a single point called its centre of mass. Depending on the object's shape, its centre of mass can be inside or outside it. The weight of an object and its mass are directly proportional. The centre of mass of an object is the point at which the object can be balanced. The centre of mass is useful because problems can often be simplified by treating a collection of masses as one mass at their common centre of mass. The weight of the object then acts through this point. In the human form the centre of mass has no fixed point but is dependent on the position of the performer at any given time. Due to the body composition the centre of mass of all athletes varies, as bone, fat, tissue and muscle all contribute to total mass and are in different proportions in each performer.