
Derive an expression for maximum speed of a car on a banked road in circular motion.
Answer
468.2k+ views
1 likes
Hint:In this question, we need to find the maximum speed of the car at which car doesn’t skid while moving in a circular banked road. We basically balance all the factors of the forces exerted on a car in x-axis direction and y-axis direction.
To solve any balancing problems, whether motion is translational or rotational, we always balance forces acting along x-axis and y-axis.
In case of rotational motion, we balance torques acting on the body.
Complete step-by-step answer:
Several formulas used in our calculations are-
Gravitational attraction force – mg
Frictional force (f) – acting between car’s tire and road, and parallel to the slope of road
Normal force (N)- It is a reaction force of the gravitational force exerted by the road on car
Centripetal force
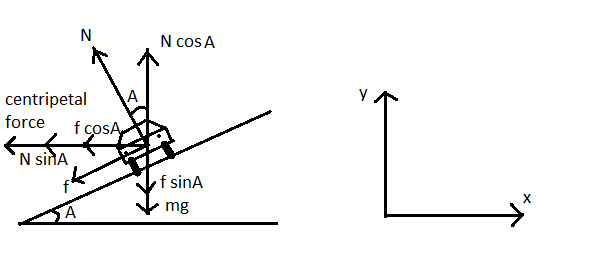
Let’s take inclination angle A of road with respect to the x-axis as shown in the above diagram. Now we can balance all the x-axis and y-axis factors of forces. Refer to the figure.
(1) Balancing all the forces acting along the x-axis-
Substituting the value
(2) Balancing all the forces acting along the y-axis-
(3) Frictional force-
Now we will use these three equations to find the value of speed (v).
Now we can divide equation (1) and (2), we get
This is an expression for maximum speed of a car on a banked road in circular motion.
Note: (1) Roads are banked to prevent high speed cars from skidding.
(2) If a car is moving at a speed more than mentioned above, it will skid.
(3) If the force of friction is not strong enough, the vehicle will skid. Also, note that friction acts downwards along the road, as the tendency of the car is to skid up.
To solve any balancing problems, whether motion is translational or rotational, we always balance forces acting along x-axis and y-axis.
In case of rotational motion, we balance torques acting on the body.
Complete step-by-step answer:
Several formulas used in our calculations are-
Gravitational attraction force – mg
Frictional force (f) – acting between car’s tire and road, and parallel to the slope of road
Normal force (N)- It is a reaction force of the gravitational force exerted by the road on car
Centripetal force

Let’s take inclination angle A of road with respect to the x-axis as shown in the above diagram. Now we can balance all the x-axis and y-axis factors of forces. Refer to the figure.
(1) Balancing all the forces acting along the x-axis-
Substituting the value
(2) Balancing all the forces acting along the y-axis-
(3) Frictional force-
Now we will use these three equations to find the value of speed (v).
Now we can divide equation (1) and (2), we get
This is an expression for maximum speed of a car on a banked road in circular motion.
Note: (1) Roads are banked to prevent high speed cars from skidding.
(2) If a car is moving at a speed more than mentioned above, it will skid.
(3) If the force of friction is not strong enough, the vehicle will skid. Also, note that friction acts downwards along the road, as the tendency of the car is to skid up.
Recently Updated Pages
JEE Main 2022 (June 29th Shift 2) Maths Question Paper with Answer Key

JEE Main 2023 (January 25th Shift 1) Maths Question Paper with Answer Key

JEE Main 2022 (July 29th Shift 1) Maths Question Paper with Answer Key

JEE Main 2022 (July 26th Shift 2) Chemistry Question Paper with Answer Key

JEE Main 2022 (June 26th Shift 2) Maths Question Paper with Answer Key

JEE Main 2022 (June 29th Shift 1) Physics Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Degree of Dissociation and Its Formula With Solved Example for JEE

Displacement-Time Graph and Velocity-Time Graph for JEE

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line




