




Signum Function
A signum function is essentially an odd function that has its application in different fields of science. Before you go any further let us first understand what an odd function is. An odd function is one whose mathematical representation is given as f(-x)=-f(x). This means that the function f(-x) has the same value or final output as that of the function being taken in terms of –x. Examples of an odd function would include f(x)=x13. If the x was replaced by –x, f(-x)=-x13=-f(x)
What is the Signum Function?
A signum function is one such odd function that is extensively used. The signum function basically gives us the sign of the real value function. This means this function is implemented to check whether the value of an input function will be positive, negative or zero. The signum function assigns either a +1 value for all the positive input i.e. input > 0, or a -1 value to all the negative inputs i.e. where input < 0. Primarily used for the purpose of prediction, this function can be immensely helpful for determining the positivity and negativity of another given function. The mathematical expression for the signum function is given as follows:
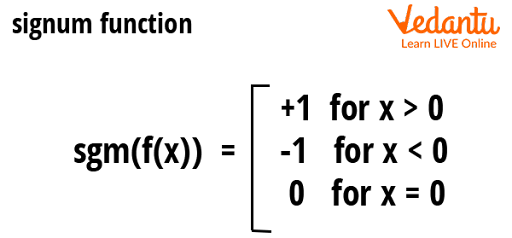
Image: The signum function
As we can see from the above mathematical representation of the signum function, the function gives us the value + 1 when the input variable x > 0. The function gives us a value of 0 and -1 when the input variable is either equal to zero or less than zero respectively, i.e. x = 0 and x < 0
Domain and Range of a Signum Function
As you already know every function has a domain and a range that ultimately defines the function. The domain is the set of numbers or values that are taken as the input i.e. all the x values where x is the independent variable. The range of any function gives us the set of elements that satisfy the function and comes out as the final output, i.e. the set of numbers consisting of all the y values where y is the dependent variable.
To understand this better let us plot the graph of the signum function.
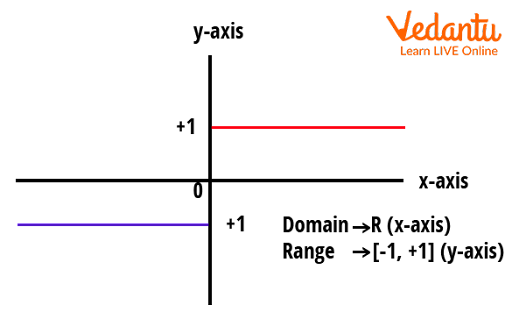
Image: Graph of the signum function
From the above-shown domain of the signum function graph, we can see that the domain of a signum function includes the whole set of real numbers. The input values are represented by the x-axis. The output values being either, +1, -1 or 0, the range of the signum function has only two values that are the range = $[-1, +1]$. The output values are represented by the y-axis, y being the dependent variable.
Applications of the Signum Function
The signum function is one of those functions that have its implementations across the other fields, including artificial intelligence and even machine learning. The Signum function can be applied to almost any absolute value function by taking the help of the identity $\operatorname{sgn}(x)=\dfrac{x}{|x|}$. In the case of complex numbers, the signum function of the complex number z (z is in the form of a + bi), is given as $\operatorname{sgn}(z)=\dfrac{z}{|z|}$. Some of the other applications of the signum function are given as follows:
The signum function is most commonly used for extracting or figuring out the signs for the set of real numbers as that is the domain of the function.
Integrating a signum function would give us a straight line that is inclined either positively or negatively from the x-axis.
Signum functions are not only used to find the sign of a complex expression but also to project them on a unit circle in the field of trigonometry.
As mentioned before, in the field of statistics, particularly for probability, the signum function is used for predictions and for the probability of the occurrence of a particular event.
The thermostat is a classic real-life example of the application of the signum function. Above the threshold temperature defined before, the thermostat starts to cool down the area and once the temperature falls beyond a certain value, the thermostat starts heating up the room. Since there are only two outputs (either cooling or heating) for an array of different input temperatures, the signum function is greatly applied here.
On and off switches heavily depend on this function. Depending on the input the switch has only two corresponding outputs, either it gets switched on or switched off. Therefore based on the input value of the switches the switch either flips on ( when y = +1) or flips off ( when y = -1 ).
Solved Problem
1. Using the signum function, find the output for the following y values,
$\begin{align} &f(y)=\left|\begin{array}{ccc} +2 & \text { if } & y>0 \\ -2 & \text { if } & y<0 \\ 0 & \text { if } & y=0 \end{array}\right| \\ &y=\{4.93,7.66,0,11,-4.2,3.33333,-5.10\} \end{align}$
Solution: For the input values of y, we utilise the signum function to determine the output,
Given us,
$\begin{align} &f(y)=\left|\begin{array}{ccc} +2 & \text { if } & y>0 \\ -2 & \text { if } & y<0 \\ 0 & \text { if } & y=0 \end{array}\right| \\ &y=\{4.93,7.66,0,11,-4.2,3.33333,-5.10\} \end{align}$
Output$= \{+2,+2,0,+2,-2,+2,-2\}$
The output for the following y value is $\{+2,+2,0,+2,-2,+2,-2\}$.
Conclusion
The signum function is an odd function used to determine the sign ( whether positive or negative ) for an absolute value function. The domain of the signum function is the set of all real numbers and the range of the signum function includes only two values, i.e. range = $[ -1, +1 ]$. The graph of the signum function can be used for a better understanding of the domain and the range. A signum function has its applications in different spheres. They are extensively used both in the field of theoretical and applied mathematics and science along with their practical applications for the same.
FAQs on Relations and Functions Signum Constant Identity and Polynomial Function
1. Is a signum function differentiable?
A signum function is one where there are only three y values. When the input is positive that is greater than 0, the output is + 1 and when the input is negative that is less than zero the output is -1. When the input is 0, the signum function upholds a value of zero as well. Thus the signum function is differentiable at all the points, through the derivative 0, except for when the input is equal to zero.
2. Is the signum function a continuous function?
A continuous function is defined as a function that does not have any sudden jumps or discontinuities owing to a change in the values. A signum function is essentially a continuous function but not everywhere. As we can see there is no sudden change along x > 0 and x < 0. The $\displaystyle \lim_{x \to 0+}=+1$ and the $\displaystyle \lim_{x \to 0-}=-1$. This means that both x > 0 and x < 0 have limits. w no limits exist at x = 0 and thus the integer valued function defined above, the set of all real numbers is discontinuous at x = 0.






















