Maths Integral Calculus Chapter - Maths JEE Main

The branch of calculus where we examine integrals and their properties is known as integral calculus. Integration is a crucial concept because it is the inverse of differentiation. The fundamental theorem of calculus connects integral and differential calculus. 

In this article, we will go over integral calculus in detail, including why it's employed, its types, formulas, examples, and applications. Also, for a better understanding and knowledge, we will go over some of the solved problems and previous year's questions.

JEE Main Maths Chapters 2025 

Important Topics of Maths Integral Calculus Chapter

• Indefinite integral

• Properties of indefinite integral

• Definite integral and its properties

• Methods of integration

• Integration by substitution

• Integration using trigonometric functions

• Integration by partial fractions

• Integration by parts

• Integral of some special types

• The fundamental theorem of calculus

Maths Integral Calculus Important Concept for JEE Main

What is Integral Calculus?

The values of the function discovered through the integration process are known as integrals. Integration is the process of obtaining f(x) from f'(x). Integrals assign numbers to functions in a way that represents displacement and motion problems, area and volume problems, and other problems that arise when little data is combined. We can find the function f by using the derivative f' of the function f and that function f is referred to as antiderivative or integral of f' in this case.

Types of Integrals

Integral calculus is used to solve problems of the following types given below:

A. The first type of problem is to find the function if its derivative is given.

B.  Also, the problem is related to finding the area bounded by the graph of a function under given conditions. Further,  the Integral calculus is divided into two types.

• Definite Integrals (in which the value of the integrals are definite)

• Indefinite Integrals (in which the value of the integral is indefinite with an arbitrary constant, C)

Indefinite Integrals

The integrals which do not have a pre-existing value of limits, which makes the final value of integral indefinite, I.e, ∫g'(x)dx = g(x) + c. Indefinite integrals are part of the family parallel curves.

Definite Integrals

Definite integral is one that has a pre-existing value of limits, which makes the final value of the integral, definite. if  a function of the curve is f(x) , then $\int\limits_a^b f(x) dx = f(b) - f(a)$

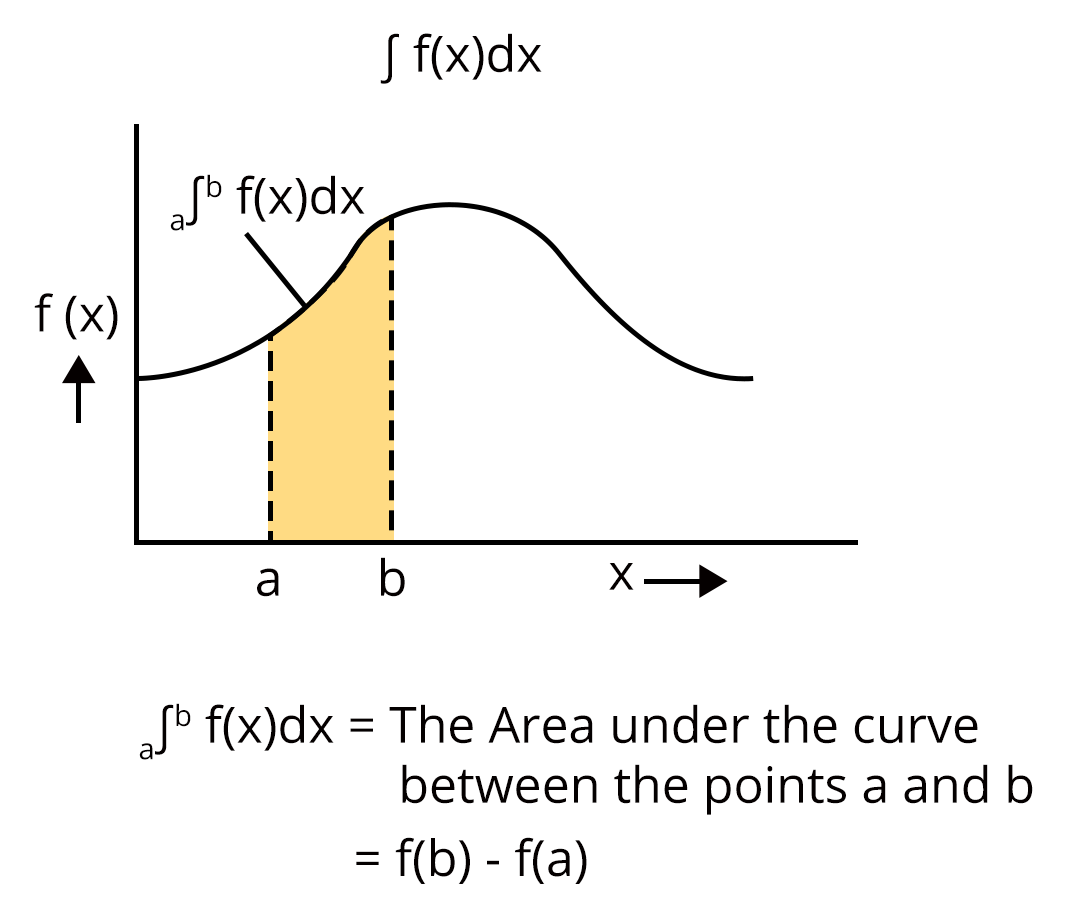
Definite Integral

Fundamental Theorems of Integral Calculus

Integrals are defined as the function of the area bounded by the curve y = f(x), a ≤ x ≤ b, at X-axis where the ordinates are x = a and x =b, and b>a. Let x be a given point in $[$a,b$]$. Then $\int\limits_a^b f(x) dx$ represents the area function. This concept of area function leads to the fundamental theorems of integral calculus.

• First Fundamental Theorem of Integral Calculus

• Second Fundamental Theorem of Integral Calculus

First Fundamental Theorem of Integrals

A(x) = $\int\limits_a^b f(x) dx$ for all x ≥ a, where the function is continuous on $[$a,b$]$. Then A'(x) = f(x) for all x ϵ $[$a,b$]$

Second Fundamental Theorem of Integrals

If f is continuous function of x defined on the closed interval $[$a,b$]$ and F is another function such that d/dx F(x) = f(x) for all x in the domain of f, then $\int\limits_a^b f(x) dx$ = f(b) -f(a). This is known as the definite integral of f over the range $[$a,b$]$, Where a is the lower limit and b is the upper limit. 

Properties of Integral Calculus

Let us go through some of the properties of the indefinite integrals:

• The derivative of an integral is the integrand of itself, ∫ f(x) dx = f(x) +C.

• Since two indefinite integrals with the same derivative produce the same family of curves, they are equivalent, ∫ $[$ f(x) dx - g(x) dx$]$ = 0.

• The sum or the difference of a finite number of functions is equal to the sum or difference of the individual functions' integrals, ∫ $[$ f(x) dx+g(x) dx$]$ = ∫ f(x) dx + ∫ g(x) dx.

• The constant is taken outside the integral sign, ∫ k f(x) dx = k ∫ f(x) dx, where k ∈ R.

• The previous two properties are combined to get the form: ∫ $[k_1 f_1(x)$ + $k_2 f_2(x)$ +... $k_n f_n(x)]\; dx$ = $k_1 \lim f_1(x)\;dx$ + $k_2 \lim f_2(x)\;dx$+ ... $k_n \lim f_n(x)\;dx$

Methods of Finding Integrals of Functions

We have different methods to find the integral of a given function in integral calculus. Some of the most commonly used methods of integration are:

• Integration by Parts

• Integration using Substitution

• Integration by Partial Fractions

Integration By Parts

Integration by parts is a technique for combining the output of two or more functions. The two $f(x)$ and $g(x)$ functions to be integrated are of the form $\int{f(x) \cdot g(x)}$. As a result, it's referred to as a product rule of integration. The first function, f(x), is chosen because its derivative formula exists, while the second function, g(x), is chosen because an integral of such a function exists.

$\int f(x) \cdot g(x) dx=f(x) \int g(x) dx-\int f^{\prime}(x) \cdot\left(\int g(x) dx\right) dx$

If we consider f to be the first function and g to be the second, we can pronounce this formula as:

The integral of the product of two functions = (first function) × (integral of the second function) – Integral of {(differential coefficient of the first function) × (integral of the second function)}

Integration By Parts Formula

If u and v are two differentiable functions of the same variable x, Then, according to the product differentiation rule, we have;

$\dfrac{d}{dx}(u v)=u\dfrac{dv}{dx}+v\dfrac{du}{dx}$

Integrating both sides, we get;

$u v=\int u \dfrac{dv}{dx} dx+\int v \dfrac{du}{dx} dx$

or

$\int u \dfrac{dv}{dx} dx=u v-\int v \dfrac{du}{dx} dx$ —(1)

Let us consider, $u=f(x)$ and $\dfrac{dv}{dx}=g(x)$

Thus,the above equation becomes

$\dfrac{du}{dx}=f^{\prime}(x)$ and $v=\int g(x) dx$

On substituting in equation 1, we get 

$\int f(x) g(x) dx=f(x) \int g(x) dx-\int\left[\int g(x) dx\right] f^{\prime}(x) dx$

or

$\int f(x) g(x) dx=f(x) \int g(x) dx-\int\left[f^{\prime}(x) \int g(x) dx\right] dx$

Integration By Substitution 

Integration by substitution is a method used to evaluate integrals that are difficult to solve using other methods. It works by substituting a new variable into the integral, which makes the integrand easier to integrate.

To use integration by substitution, we follow these steps:

• Identify a function u(x) that appears in the integrand.

• We let u=u(x) and substitute it into the integral. This gives us an integral in terms of the new variable u.

• Integrate the integral in terms of u.

• Substitute x back into the integral to get the answer in terms of x.

Integration by Partial Fractions

Integration by partial fractions is a method for decomposing and integrating a rational fraction with complex terms in the denominator. We calculate and decompose the expression into simpler terms using partial fractions so that we can easily calculate or integrate the resulting expression.

Integration Using Trigonometric Functions

Integration using trigonometric functions is a technique used to evaluate integrals that involve trigonometric functions. It is based on the trigonometric identities that relate the different trigonometric functions.

Some common trigonometric identities that are used in integration include:

$\sin^2(x)+\cos^2(x)=1$

$1+\tan^2(x)=\sec^2(x)$

$1+\cot^2(x)=\csc^2(x)$

$\sin(2x)=2\sin(x)\cos(x)$

$\cos(2x)=\cos^2(x)−\sin^2(x)$

Note: While solving trigonometric identities and substitutions don't forget to substitute back to the original variable if you initially made a substitution. This step is crucial to express your final answer in terms of the original variable.

Area Under the Curve

A definite integral between two points is used to find the area under a curve between two points. Integrate y = f(x) between the limits of a and b to find the area under the curve y = f(x) between x = a and x = b. This area can be calculated by integrating within certain bounds.

Formula for Area under the Curve $= \int_{a}^{b} f(x)dx$

Special Types of Integral

• Integrals of Rational Functions: Rational functions are functions that can be written as the quotient of two polynomials. The integrals of rational functions can be evaluated using a variety of methods, including partial fractions, u-substitution, and integration by parts.

• Integrals of Trigonometric Functions: The integrals of trigonometric functions can be evaluated using a variety of methods, including trigonometric identities, u-substitution, and integration by parts.

• Integrals of Exponential Functions: The integrals of exponential functions can be evaluated using the following formula:

∫ e^x dx = e^x + C

where C is an arbitrary constant.

• Integrals of Logarithmic Functions: The integrals of logarithmic functions can be evaluated using the following formula:

∫ ln(x) dx = x ln(x) - x + C

where C is an arbitrary constant.

• Integrals of Improper Integrals: Improper integrals are integrals that have an infinite interval of integration or an integrand that is infinite at a point in the interval of integration. Improper integrals can be evaluated using a variety of methods, including limits and l'Hospital's rule.

Uses of Integral Calculus

Integral Calculus is mainly used for the two purposes mentioned below:

• To calculate f from f’: If a function f is differentiable in the interval of consideration, then f’ is defined. We've already seen how to calculate a function's derivatives in differential calculus, and we can "undo" that with the help of integral calculus.

• To find the area under a curve.

Application of Integral Calculus

Some of the important applications of the integral calculus are - Integration is applied to find:

• The area between two curves

• Centre of mass

• Kinetic energy

• Surface area

• Work

• Distance, velocity and acceleration

• The average value of a function

• Volume

Example 1: Find the integral of the function f(x) = $\sqrt{x}$.

Solution:

Given,

f(x) = $\sqrt{x}$

∫f(x) dx = $\int \sqrt{x} dx$

$\begin{array}{l}\int \sqrt{x}\ dx = \int x^{\frac{1}{2}}\ dx\end{array} $

We know that, $\begin{array}{l}\int x^{n}\ dx = \frac{x^{n+1}}{n+1}+C\end{array} $

Now, $\begin{array}{l}\int \sqrt{x}\ dx = \frac{x^{\frac{1}{2}+1}}{\frac{1}{2}+1}+C\\= \frac{x^{\frac{1+2}{2}}}{\frac{1+2}{2}}+C\\=\frac{2}{3}x^{\frac{3}{2}}+C\end{array} $

Example 2: Find the integral of cos2n with respect to n.

Solution:

Let f(n) = cos2n 

we know that 2 cos2A = cos 2A + 1

So, f(n) = (1/2)(cos 2n + 1)

Let us find the integral of f(n).

∫f(n) dn = ∫(1/2)(cos 2n + 1) dn

= (1/2) ∫(cos 2n + 1) dn

= (1/2) ∫cos 2n dn + (1/2)∫1 dn

= (1/2) (sin 2n/2) + (1/2) n + C

= (sin 2n/4) + (n/4) + C

=(1/4)$[$sin 2n + n$]$ + C

Previous Year Questions For JEE Main

Question 1: ∫{$[$sin8x − cos8x$]$ / $[$1 − 2 sin2x cos2x$]$} dx = _________.

Solution:

∫{$[$sin8x − cos8x$]$ / $[$1 − 2 sin2x cos2x$]$} dx

= ∫{$[$(sin4x + cos4x) * (sin4x − cos4x)$]$ / $[$(sin2x + cos2x)2 − 2 sin2x cos2x$]$} dx

= ∫(sin4x – cos4x) dx

= ∫$[$sin2x + cos2x$] \times [$sin2x – cos2x$]$ dx

= ∫(sin2x - cos2x) dx

= ∫−cos2x dx

= $[$−sin2x/2$]$ + c

Question 2: ∫x2dx / (a + bx)2 = ___________.

Solution:

$ \int \frac{x^{2}}{(a+b x)^{2}} d x $

Let $ a+b x=t \Rightarrow b\; d x=d t $

$ s_{0}, \frac{1}{b} \int \left(\frac{t-a}{b t}\right)^{2} d t=\frac{1}{b^{3}} \int\left(1-\frac{a}{t}\right)^{2} d t $

$ =\frac{1}{b^{3}} \int\left(1+\frac{a^{2}}{t^{2}}-\frac{2 a}{t}\right) d t $

$=\frac{1}{b^3}\left [ \int dt +a^2\int\frac{dt}{t^2}-2a\int \frac{dt}{t}\right ]$

$ =\frac{1}{b^{3}}\left[ t+a^{2} \left( -\frac{1}{t} \right) -2 a\; ln\; t \right]+c $

$ =\frac{a+b x}{b^{3}}-\frac{a^{2}}{b^{3}(a+b x)}-\frac{2 a}{b^{3}} ln (a+bx)+c $

$ =\frac{x}{b^{2}}-\frac{a^{2}}{b^{3}(a+b x)}-\frac{2 a}{b^{3}} ln (a+bx)+k $

Question 3: $\int \left [ {x^5}/{\sqrt{1+x^3}} \right ] dx$ = ________.

Solution:

Put 1 + x3 = t2

⇒ 3x2 dx = 2tdt and x3 = t2 − 1

So, ∫$x^5 /\sqrt{1+x^3} \; dx$ = ∫{$[x^2$ $\times$ $x^3]$ / $\sqrt{1+x^3} \; dx$

= $\left [ \dfrac{2}{3} \right ]$ ∫{$[$(t2 − 1) $\times$ t$]$ dt / $[$t$]$}

= $\left [ \dfrac{2}{3} \right ]$ ∫(t2 − 1) dt

= $\left [ \dfrac{2}{3} \right ]$ $[$(t3 / 3) − t$]$ + c

= $\left [ \dfrac{2}{3} \right ]$ $[${(1 + x3)3/2 / 3} − {(1 + x3)½}$]$+ c

Practise Problems 

1. Let p(x) be a function defined on R such that p'(x) = p'(1 – x), for all x $\in$0, 1], p(0) = 1 and p(1) = 41. Then $\int_{0}^{1}$ p(x) dx equals:

(A) $\sqrt{41}$ 

(B) 21 

(C) 41 

(D) 42

2. The value of $\int_{0}^{1} \frac{8 \log (1+x)}{1+x^{2}} d x$ is:

(A) $\frac{\pi}{2} \log 2$

(B) $\log 2$

(C) $\pi \log 2$

(D) $\frac{\pi}{8} \log 2$

Answers: 1. (B), 2. (C)

JEE Main Maths - Integral Calculus Study Materials

Here, you'll find a comprehensive collection of study resources for Integral Calculus designed to help you excel in your JEE Main preparation. These materials cover various topics, providing you with a range of valuable content to support your studies. Simply click on the links below to access the study materials of Integral Calculus and enhance your preparation for this challenging exam.

JEE Main Maths Study and Practice Materials

Explore an array of resources in the JEE Main Maths Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Maths preparation.

Conclusion

In this article, we will dive deep into the world of Integral Calculus, a crucial chapter for JEE Main. We will unravel fundamental concepts and provide step-by-step solutions to tackle various integral calculus problems. Whether you're struggling with integration techniques, definite or indefinite integrals, or applications of integrals, you will find all the answers right here. We've simplified complex theories and provided free downloadable resources like PDFs, so you can grasp these concepts effortlessly. Mastering Integral Calculus through this guide will undoubtedly boost your confidence and performance in your exams.