Question

In the circuit shown in the figure, find the current in \[45\Omega \].

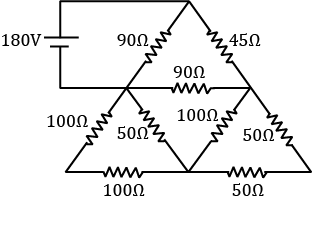
A. \[4\;{\rm{A}}\]
B. \[2.5\;{\rm{A}}\]
C. \[2\;{\rm{A}}\]
D. None of these

Answer 1

Hint: In this case, we have to calculate the voltage drops across the resistance elements using Ohm's Law. The values of these currents are then entered into the Current law expression to obtain a suitable value for current. We'll keep simplifying the circuit by employing equivalent resistances instead of a resistor combination. On the last simplest circuit, we will discover the current, divide it into branches, and then find the current via each branch.

Formula used:
The formula for finding the current in \[45\Omega \] are:
\[v = i \times R\]
Equivalent resistance in series is given by
\[R = {R_1} + {R_2} + ....... + {R_9}\]
Equivalent resistance in parallel is given by
\[\dfrac{1}{{{R_p}}} = \dfrac{1}{{{R_1}}} + \dfrac{1}{{{R_2}}} + ....... + \dfrac{1}{{{R_n}}}\]

Complete step by step solution:
We have been given the circuit diagram in the question. Now, let us split the circuit into branches for easy understanding, we obtain



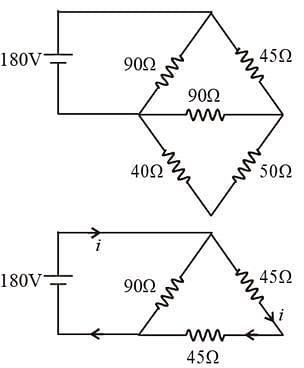
In the given circuit, we have been understood that voltage is
\[180\;{\rm{V}}\]
The sign “I” is commonly used to represent current. Ohm's law states that the current flowing through a conductor is proportional to the voltage V and resistance R.
That is, \[{\rm{V = IR}}\]
Ohm's law can also be stated as,
\[{\rm{i = }}\dfrac{{\rm{V}}}{{\rm{R}}}\]
Now, we have to substitute the values given in the circuit in the above formula, we get
\[i = \dfrac{{180}}{{90}}\]
Now, let’s simplify the above expression, we get
\[{\rm{i = 2A}}\]
Therefore, the current in \[45\Omega \] is \[{\rm{2}}\] Ampere.

Hence, the option C is correct.

Note: Keep in mind that current does not always divide evenly between the two branches of the circuit. It is determined by the amount of resistance between them. The current was divided equally in this case because the resistance on the two branches of the circuit was equal.