
If the angular distance between the stars turns out to be approximately

A)
B)
C)
D)
Answer
153.9k+ views
Hint: The phenomenon mentioned in the given question is parallax. Parallax is the apparent angular displacement of a celestial body due to its being observed from the surface of the earth instead of the centre of the earth. Parallax also arises due to a change in viewpoint caused by relative motion.
Complete step by step solution:
From the given question, we can say that the shift in the angular distance of the moon when viewed from two different vantage points is
Since angular measurements are usually made in radians, we must convert the given angular distance into radians.
Using the properties of circles and arcs, we can say that arc length in a circle is equal to the product of the radius and the angle subtended by the arc.
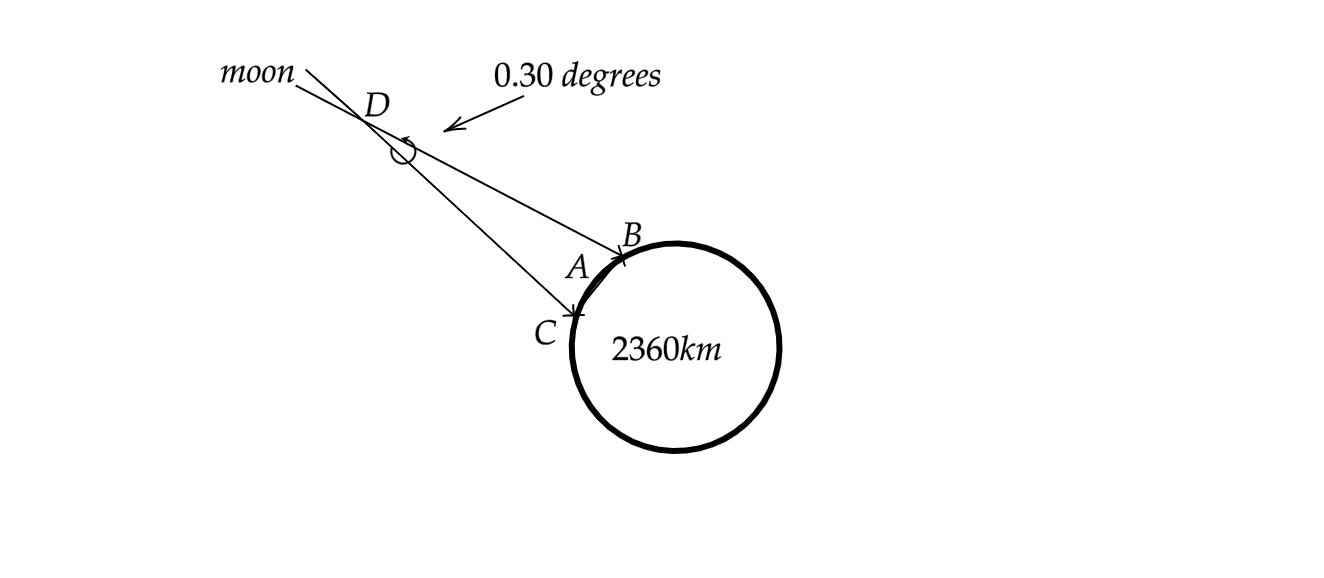
Since the distance between the moon and the earth is more than the radius of the earth, the distance between the moon and the earth, DA, is roughly equal to DC.
Now for the arc BAC, we can say that arc length will be equal to the product of the radius, DC, and the angle subtended at DC.
Mathematically, we can say that
Substituting the values, we get
Hence, option (A) is the correct answer.
Note:An alternative method to approach this question is using the definition of parallax. The Parallax formula states that the distance to a star is equal to the distance between the vantage points divided by the parallax angle, where the parallax angle is measured in arcseconds.
Complete step by step solution:
From the given question, we can say that the shift in the angular distance of the moon when viewed from two different vantage points is
Since angular measurements are usually made in radians, we must convert the given angular distance into radians.
Using the properties of circles and arcs, we can say that arc length in a circle is equal to the product of the radius and the angle subtended by the arc.

Since the distance between the moon and the earth is more than the radius of the earth, the distance between the moon and the earth, DA, is roughly equal to DC.
Now for the arc BAC, we can say that arc length will be equal to the product of the radius, DC, and the angle subtended at DC.
Mathematically, we can say that
Substituting the values, we get
Hence, option (A) is the correct answer.
Note:An alternative method to approach this question is using the definition of parallax. The Parallax formula states that the distance to a star is equal to the distance between the vantage points divided by the parallax angle, where the parallax angle is measured in arcseconds.
Recently Updated Pages
JEE Main 2022 (June 29th Shift 2) Maths Question Paper with Answer Key

JEE Main 2023 (January 25th Shift 1) Maths Question Paper with Answer Key

JEE Main 2022 (July 29th Shift 1) Maths Question Paper with Answer Key

JEE Main 2022 (July 26th Shift 2) Chemistry Question Paper with Answer Key

JEE Main 2022 (June 26th Shift 2) Maths Question Paper with Answer Key

JEE Main 2022 (June 29th Shift 1) Physics Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Degree of Dissociation and Its Formula With Solved Example for JEE

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Electrical Field of Charged Spherical Shell - JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line




