Question

If d is the distance between the source of sound and reflector \[{{\text{t}}_{\text{1}}}\] , \[{{\text{t}}_{\text{2}}}\] are times at which echoes are heard, the expression for determination of velocity of sound in air by echo method is:
(A) \[\dfrac{{{\text{2d}}}}{{{t_2} - {t_1}}}\]
(B) \[\dfrac{{{\text{2d}}}}{{{t_1} - {t_2}}}\]
(C) \[\dfrac{{{\text{4d}}}}{{{t_1} - {t_2}}}\]
(D) \[\dfrac{{{\text{2d}}}}{{{t_1} + {t_2}}}\]

Answer 1

Hint: We will find the total distance travelled and total time taken by the echo to reach the source. Then, we will substitute it in the formula for finding the speed of any object.

Complete step by step answer
An echo is the repetition of sound waves, after reflection from any obstacle.
We are already familiar with the formula for calculating the speed, i.e.,
Speed \[{\text{ = }}\dfrac{{{\text{distance}}}}{{{\text{time}}}}\]…………………………………(i)
We use the Echo method to find the large distances of objects like Hill or a mountain.
Now, let’s consider a man standing at a point A x meters away from a hill situated at point B as shown in the figure.

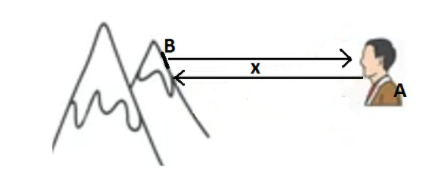
So, the sound wave will travel from point A to point B. Here it will reflect back by touching the mountain and again travel back from point B to A. Suppose that \[{{\text{t}}_{\text{1}}}\] is the time at which the man produces the sound and \[{{\text{t}}_{\text{2}}}\] be the time at which the man heard the echo of the sound produced by him.
This means the total time taken for the echo to be heard will be, \[t = {t_2} - {t_1}\]
Now, the total distance travelled by the sound wave is, \[{\text{AB + BA = x + x = 2x}}\]
We will apply the formula of speed given in Eq.(i),
speed\[{\text{ = }}\dfrac{{{\text{2x}}}}{{{t_2} - {t_1}}}\](on substituting the values of distance and time)
In the question, the total distance travelled by the wave is given as ‘d’. Substitute this value in place of x.
Speed\[{\text{ = }}\dfrac{{{\text{2d}}}}{{{t_2} - {t_1}}}\]
This is the speed of the sound wave after an echo.

So option (a.) is the correct option.

Note Since we are calculating the distance for an echo, it is important to consider both the distances (i.e., distance travelled by the sound wave before and after the reflection). Forgetting even one might result in getting a wrong answer.