
If a body moving in a circular path maintains a constant speed of $410m{s^{ - 1}}$, then which of the following correctly describes the relation between acceleration and radius?
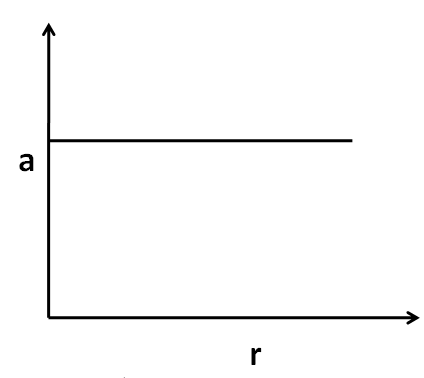
(A)
(B)
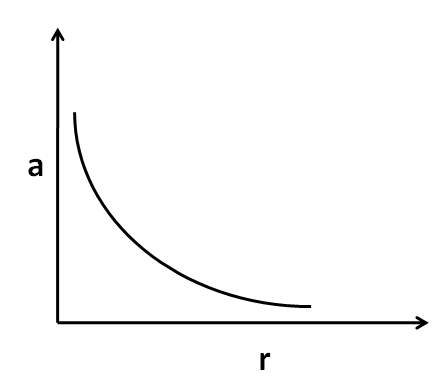
(C)
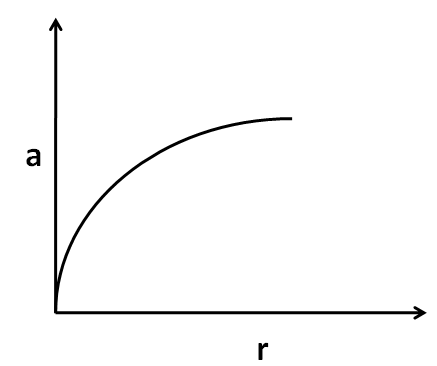
(D)
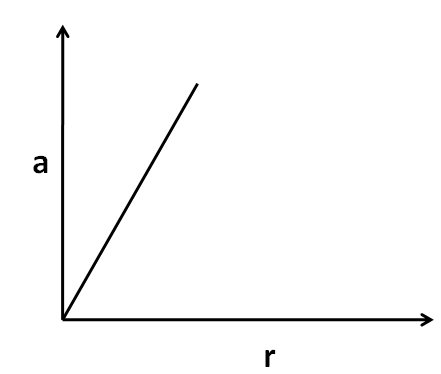




Answer
153.6k+ views
Hint: To solve this question, we need to use the formulae of the tangential and the centripetal acceleration of the body. Then, according to the information given in the question, we can find out the expression for the net acceleration of the body in terms of the radius. From that expression, the shape of the graph can be predicted.
Formula used: The formulae used for solving this question are
${a_C} = \dfrac{{{v^2}}}{r}$
${a_T} = \alpha r$
Here ${a_C}$ and ${a_T}$ are respectively the centripetal and the tangential acceleration of a body moving in a circle of radius $r$ with the velocity $v$ and the angular acceleration of $\alpha $.
Complete step-by-step solution:
We know that for a body moving in the circular motion, the acceleration of the body has two components, one is the tangential acceleration and the other is the centripetal acceleration.
Now, we know that the centripetal acceleration is given by the expression
${a_C} = \dfrac{{{v^2}}}{r}$.......................(1)
Also, we know that the tangential acceleration is given by the expression
${a_T} = \alpha r$..........................(2)
According to the question, the speed of the body is constant. This means that the body moves in the uniform circular motion. We know that in the uniform circular motion, the angular acceleration is equal to zero. Therefore substituting $\alpha = 0$ in (2) we have
${a_T} = 0$............................(3)
Now, the tangential acceleration is along the tangent to the circle, and the centripetal acceleration is along the radius of the circle. We know that the tangent is perpendicular to the radius. So the tangential acceleration is perpendicular to the centripetal acceleration, and hence, the net acceleration of the body is given by
$a = \sqrt {{a_C}^2 + {a_T}^2} $
Putting (2) and (3) in the above equation, we get
$a = \sqrt {{{\left( {\dfrac{{{v^2}}}{r}} \right)}^2} + {{\left( 0 \right)}^2}} $
$ \Rightarrow a = \dfrac{{{v^2}}}{r}$
So this is the net acceleration of the body moving in the circular motion. According to the question the speed of the body is constant. So we can write
$a = \dfrac{c}{r}$.........................................(4)
In the given options, the acceleration is plotted along the y-axis, and the radius is plotted along the x-axis. Therefore substituting $a = y$ and $r = x$ in (4) we get
$y = \dfrac{c}{x}$
$ \Rightarrow xy = c$
We know that the above equation represents the equation of a rectangular hyperbola. So the graph between the acceleration and the radius should look like
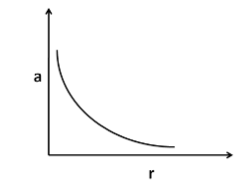
Hence, the correct answer is option B.
Note: We should not neglect the tangential component of the acceleration of the body. It is equal to zero for a uniform circular motion, but in the non-uniform circular motion it is non zero. Also, the value of the speed of the particle given in the question is just extra information as we do not need it to predict the shape of the graph.
Formula used: The formulae used for solving this question are
${a_C} = \dfrac{{{v^2}}}{r}$
${a_T} = \alpha r$
Here ${a_C}$ and ${a_T}$ are respectively the centripetal and the tangential acceleration of a body moving in a circle of radius $r$ with the velocity $v$ and the angular acceleration of $\alpha $.
Complete step-by-step solution:
We know that for a body moving in the circular motion, the acceleration of the body has two components, one is the tangential acceleration and the other is the centripetal acceleration.
Now, we know that the centripetal acceleration is given by the expression
${a_C} = \dfrac{{{v^2}}}{r}$.......................(1)
Also, we know that the tangential acceleration is given by the expression
${a_T} = \alpha r$..........................(2)
According to the question, the speed of the body is constant. This means that the body moves in the uniform circular motion. We know that in the uniform circular motion, the angular acceleration is equal to zero. Therefore substituting $\alpha = 0$ in (2) we have
${a_T} = 0$............................(3)
Now, the tangential acceleration is along the tangent to the circle, and the centripetal acceleration is along the radius of the circle. We know that the tangent is perpendicular to the radius. So the tangential acceleration is perpendicular to the centripetal acceleration, and hence, the net acceleration of the body is given by
$a = \sqrt {{a_C}^2 + {a_T}^2} $
Putting (2) and (3) in the above equation, we get
$a = \sqrt {{{\left( {\dfrac{{{v^2}}}{r}} \right)}^2} + {{\left( 0 \right)}^2}} $
$ \Rightarrow a = \dfrac{{{v^2}}}{r}$
So this is the net acceleration of the body moving in the circular motion. According to the question the speed of the body is constant. So we can write
$a = \dfrac{c}{r}$.........................................(4)
In the given options, the acceleration is plotted along the y-axis, and the radius is plotted along the x-axis. Therefore substituting $a = y$ and $r = x$ in (4) we get
$y = \dfrac{c}{x}$
$ \Rightarrow xy = c$
We know that the above equation represents the equation of a rectangular hyperbola. So the graph between the acceleration and the radius should look like

Hence, the correct answer is option B.
Note: We should not neglect the tangential component of the acceleration of the body. It is equal to zero for a uniform circular motion, but in the non-uniform circular motion it is non zero. Also, the value of the speed of the particle given in the question is just extra information as we do not need it to predict the shape of the graph.
Recently Updated Pages
JEE Main 2022 (June 29th Shift 2) Maths Question Paper with Answer Key

JEE Main 2023 (January 25th Shift 1) Maths Question Paper with Answer Key

JEE Main 2022 (July 29th Shift 1) Maths Question Paper with Answer Key

JEE Main 2022 (July 26th Shift 2) Chemistry Question Paper with Answer Key

JEE Main 2022 (June 26th Shift 2) Maths Question Paper with Answer Key

JEE Main 2022 (June 29th Shift 1) Physics Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Degree of Dissociation and Its Formula With Solved Example for JEE

Displacement-Time Graph and Velocity-Time Graph for JEE

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line




