Question

From the top of a hill h meters high, the angles of depressions of the top and bottom of a pillar are $\alpha $ and $\beta $, respectively. The height (in meters) of the pillar is?
A. $\dfrac{{h[\tan \beta - \tan \alpha ]}}{{\tan \beta }}$
B.$\dfrac{{h[\tan \alpha - \tan \beta ]}}{{\tan \alpha }}$
C. $\dfrac{{h[\tan \alpha + \tan \beta ]}}{{\tan \beta }}$
D. $\dfrac{{h[\tan \alpha + \tan \beta ]}}{{\tan \alpha }}$

Answer 1

Hint: Since the problem is based on applications of trigonometry hence, it is necessary to draw the figure for better visualization of the problem. Here, we will use proper trigonometric identities and ratios and then equate them to get the required dimension of a pillar and find out the solution to a given problem.

Complete step by step Solution:
The angles of depression of the top and bottom of a pillar $\alpha $ and $\beta $ respectively (given).
Let the height of the hill $(AD)$ be $h$ and the height of the pillar $(CE)$ be ${h_1}$ as shown in the figure.
Let the distance between the feet of the hill and pillar $(DE)$ be $x$.

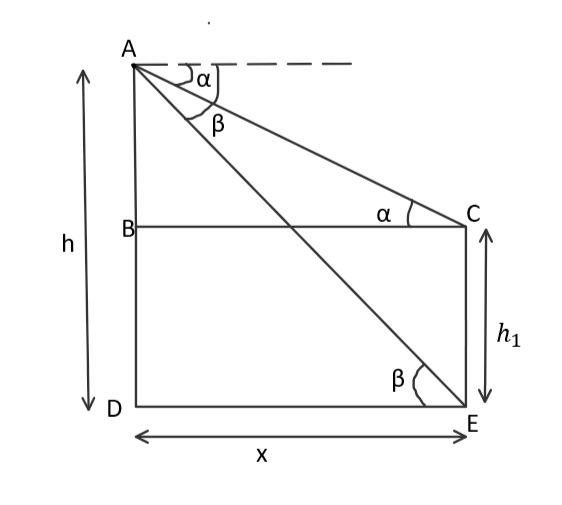
In \[\vartriangle ABC\],
$\tan \alpha = \dfrac{P}{B} = \dfrac{{AB}}{{BC}}$
From the figure, it is clear that $AB = h - {h_1}$ and $BC = x$, substitute these values in the above expression, we get
$\tan \alpha = \dfrac{{h - {h_1}}}{x}$
$x = \dfrac{{h - {h_1}}}{{\tan \alpha }}$ …. (1)
In \[\vartriangle ADE\],
$\tan \beta = \dfrac{P}{B} = \dfrac{{AD}}{{DE}}$
From the figure, it is clear that $AD = h$ and $DE = x$, substitute these values in the above expression, we get
$\tan \beta = \dfrac{h}{x}$
$x = \dfrac{h}{{\tan \beta }}$ …. (2)
Equating eq. (1) and (2), we get
$\dfrac{{h - {h_1}}}{{\tan \alpha }} = \dfrac{h}{{\tan \beta }}$
By Cross-multiplication,
$h.\tan \beta - {h_1}.\tan \beta = h.\tan \alpha $
$h.\tan \beta - h.\tan \alpha = {h_1}.\tan \beta $
Taking common$h$from the above expression, we get
$h(\tan \beta - \tan \alpha ) = {h_1}.\tan \beta $
${h_1} = \dfrac{{h(\tan \beta - \tan \alpha )}}{{\tan \beta }}$
Thus, the Height of the pillar is $\dfrac{{h(\tan \beta - \tan \alpha )}}{{\tan \beta }}$ .

Hence, the correct option is A.

Note: In the problems based on the application of trigonometry, apply the required trigonometric ratios to get the accurate solution to the problem. Sometimes, the problem seems to be complex while analyzing the problem hence, it is advised to draw a figure and calculate ratios one by one carefully.