
How many electrons should be removed from a coin of mass $1.6g$, so that it may float in an electric field of intensity ${10^9}N{C^{ - 1}}$ directed upward?
A) $9.8 \times {10^7}$
B) $9.8 \times {10^5}$
C) $9.8 \times {10^3}$
D) $9.8 \times {10^1}$
Answer
154.5k+ views
Hint: In the question, it’s given that the coin is floating. The coin will float only when the net force is zero or the forces are balanced i.e. the force due to the electric field is equal to the weight of the electron. From this, we can easily calculate the charge required to balance the electron and from that, we can calculate the amount of electron which will be removed.
Formulae used:
${N_e} = \dfrac{q}{{{C_e}}}$
Here ${N_e}$ is the number of electrons, $q$ is the total charge and ${C_e}$ is the charge on one electron.
Complete step by step answer:
In the question, a coin of mass $1.6g$ is given. It is said that it is floating in the electric field of $109N{C^{ - 1}}$.
Let us draw the free body diagram of the coin.
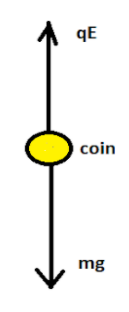
For the coin to float it should be in a balanced condition. The upward force acting on the coin should be equal to the downward force acting on the body. Hence
$ \Rightarrow qE = mg$
Where $q$ is the net charge on the coin, $E$ is the electric field acting on the body, $m$ is the mass of the coin and $g$ is the acceleration due to gravity.
Let this be equation 1.
It’s given that,
$m = 1.6g = 1.6 \times {10^{ - 3}}kg$
$E = {10^9}N{C^{ - 1}}$
$g = 9.8m{s^{ - 2}}$
Putting the values of $m$, $E$ and $g$ in the equation 1, we get,
$ \Rightarrow q \times 109 = 1.6 \times {10^{ - 3}} \times 9.8$
$ \therefore q = \dfrac{{1.6 \times {{10}^{ - 3}} \times 9.8}}{{{{10}^9}}}$
We know that the charge on one electron is $1.6 \times {10^{ - 19}}C$.
The number of electrons that is required to be removed,
$ \Rightarrow {N_e} = \dfrac{q}{{{C_e}}}$
Here ${N_e}$ is the number of electrons, $q$ is the total charge and ${C_e}$ is the charge on one electron.
$ \Rightarrow {N_e} = \dfrac{q}{{{C_e}}}$
$ \therefore {N_e} = \dfrac{{\dfrac{{1.6 \times {{10}^{ - 3}} \times 9.8}}{{{{10}^9}}}}}{{1.6 \times {{10}^{ - 19}}C}} = 9.8 \times {10^7}$
So option (A) is the correct answer.
Note: Any charge when placed in an external electric field will experience an electric force. In this case the force experienced by all the electrons in the coin should balance the weight of the coin. We will not consider the electric force acting on the protons because this electric force acting on them is negligible as compared to the intranuclear forces inside the nucleus of the atoms. So, only the force on the electrons is considered.
Formulae used:
${N_e} = \dfrac{q}{{{C_e}}}$
Here ${N_e}$ is the number of electrons, $q$ is the total charge and ${C_e}$ is the charge on one electron.
Complete step by step answer:
In the question, a coin of mass $1.6g$ is given. It is said that it is floating in the electric field of $109N{C^{ - 1}}$.
Let us draw the free body diagram of the coin.

For the coin to float it should be in a balanced condition. The upward force acting on the coin should be equal to the downward force acting on the body. Hence
$ \Rightarrow qE = mg$
Where $q$ is the net charge on the coin, $E$ is the electric field acting on the body, $m$ is the mass of the coin and $g$ is the acceleration due to gravity.
Let this be equation 1.
It’s given that,
$m = 1.6g = 1.6 \times {10^{ - 3}}kg$
$E = {10^9}N{C^{ - 1}}$
$g = 9.8m{s^{ - 2}}$
Putting the values of $m$, $E$ and $g$ in the equation 1, we get,
$ \Rightarrow q \times 109 = 1.6 \times {10^{ - 3}} \times 9.8$
$ \therefore q = \dfrac{{1.6 \times {{10}^{ - 3}} \times 9.8}}{{{{10}^9}}}$
We know that the charge on one electron is $1.6 \times {10^{ - 19}}C$.
The number of electrons that is required to be removed,
$ \Rightarrow {N_e} = \dfrac{q}{{{C_e}}}$
Here ${N_e}$ is the number of electrons, $q$ is the total charge and ${C_e}$ is the charge on one electron.
$ \Rightarrow {N_e} = \dfrac{q}{{{C_e}}}$
$ \therefore {N_e} = \dfrac{{\dfrac{{1.6 \times {{10}^{ - 3}} \times 9.8}}{{{{10}^9}}}}}{{1.6 \times {{10}^{ - 19}}C}} = 9.8 \times {10^7}$
So option (A) is the correct answer.
Note: Any charge when placed in an external electric field will experience an electric force. In this case the force experienced by all the electrons in the coin should balance the weight of the coin. We will not consider the electric force acting on the protons because this electric force acting on them is negligible as compared to the intranuclear forces inside the nucleus of the atoms. So, only the force on the electrons is considered.
Recently Updated Pages
Wheatstone Bridge - Working Principle, Formula, Derivation, Application

Young's Double Slit Experiment Step by Step Derivation

JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

Chemical Properties of Hydrogen - Important Concepts for JEE Exam Preparation

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Degree of Dissociation and Its Formula With Solved Example for JEE

Displacement-Time Graph and Velocity-Time Graph for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

JEE Advanced 2025 Notes

Electrical Field of Charged Spherical Shell - JEE

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025




