
Consider the circuit shown in the figure
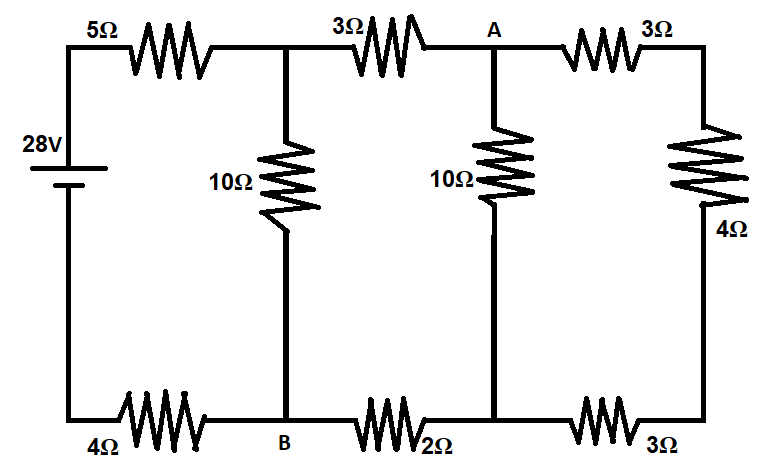
A) The current in the
B) The current in the
C) The potential difference
D) The potential difference
Answer
153.9k+ views
Hint:To find the current through the voltage source, we have to evaluate resistance and apply Ohm's law. Calculate the equivalent resistance from the rightmost resistors and evaluate the voltage. Then use Kirchhoff's law to evaluate the potential. According to Kirchhoff's law, the algebraic sum of all currents entering a junction will be equal to the algebraic sum leaving the junction.
Formula used:
i) In series,
ii) In parallel,
iii) The current using Ohm’s law is given by,
Complete step by step solution:
Let us consider the circuit from the point marked A.
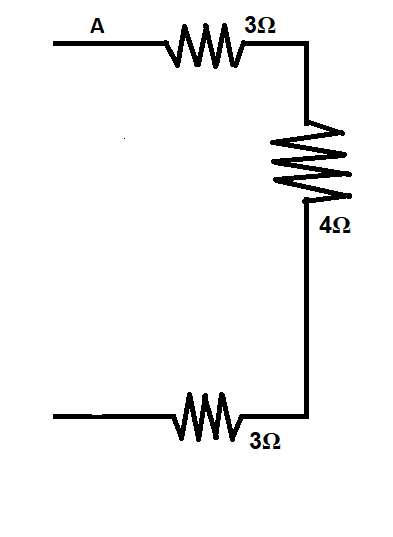
The three resistors
Resistance equivalent of
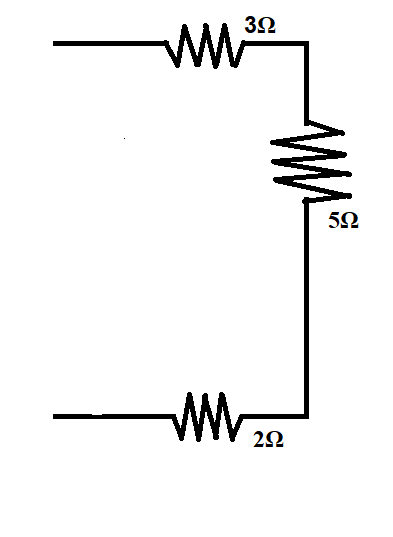
Now let us replace the three resistors in series with their equivalent resistance and consider the next part of the circuit
Now the two resistors are in parallel, their equivalent resistance can be calculated in the following steps.
Effective resistance,
The equivalent resistance,
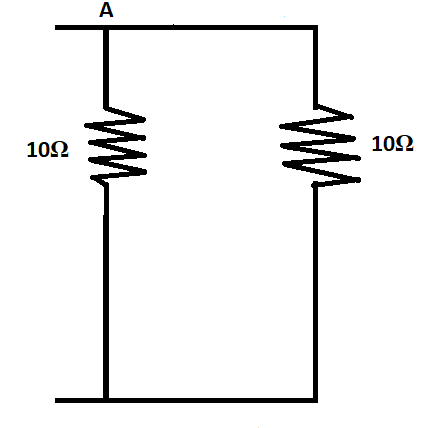
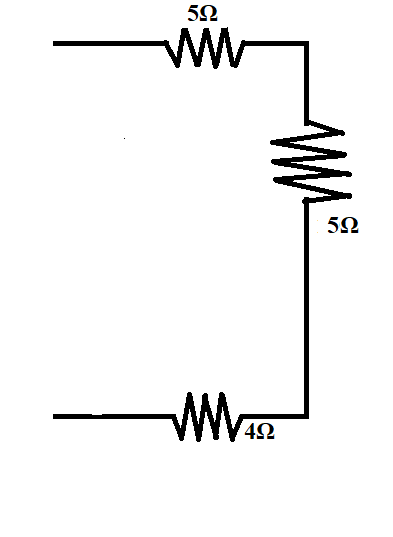
Now we can replace the two resistance with their equivalent resistance in the circuit,
Now, these three resistances are in series, their equivalent resistance can be calculated as,
Resistance equivalent
Now these three resistors can be replaced by their equivalent resistance
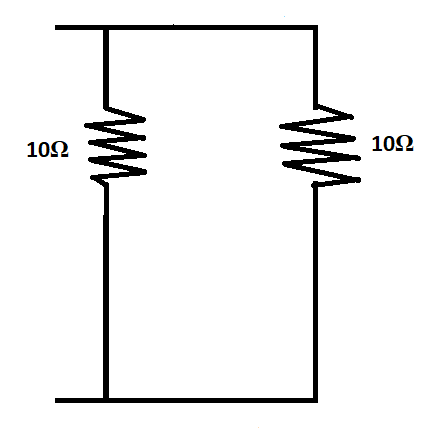
These two resistors are in parallel connection, hence their equivalent resistance is given by,
Effective resistance,
The equivalent resistance,
Replacing these two resistors in the circuit,
Now the effective resistance of these three resistors will be the total equivalent resistance of the entire circuit.
Since the three resistors are in series,
The equivalent resistance of the circuit,
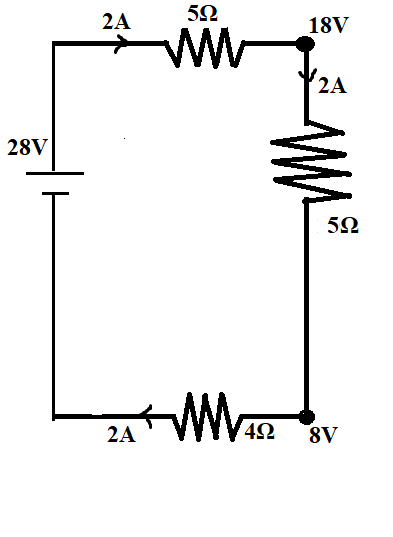
The Potential difference is
Current through the source can be obtained by applying Ohm’s law
Given that
The current through the source,
To find the current through each branch, we have to go through the simplified circuit in the reverse order.
The current through
The current through the next
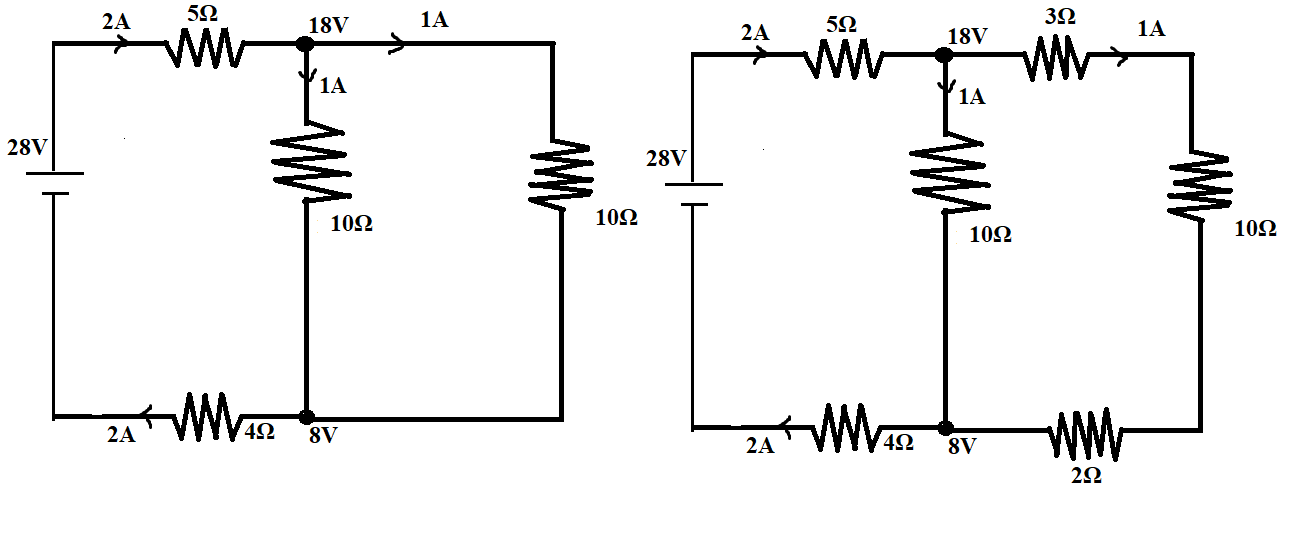
The
The current through the next
In the same way, the current through the next

There are again two branches with equal resistance, the current will again split into half,
The current through the next
The current through the next
The current through the next
Applying Kirchhoff’s law from A to B,
From the above equation, we get
The correct answer is (A), The current in the
Note:To find the equivalent resistance of several resistors that are connected in series we take the individual sum of resistances. In a series combination, the equivalent resistance will be higher than the value of the highest resistance in the circuit. On the other hand, in parallel combination, the effective resistance will be less than the lowest value of resistance in the circuit.
Formula used:
i) In series,
ii) In parallel,
iii) The current using Ohm’s law is given by,
Complete step by step solution:
Let us consider the circuit from the point marked A.

The three resistors
Resistance equivalent of
Now let us replace the three resistors in series with their equivalent resistance and consider the next part of the circuit

Now the two resistors are in parallel, their equivalent resistance can be calculated in the following steps.
Effective resistance,
The equivalent resistance,
Now we can replace the two resistance with their equivalent resistance in the circuit,

Now, these three resistances are in series, their equivalent resistance can be calculated as,
Resistance equivalent
Now these three resistors can be replaced by their equivalent resistance

These two resistors are in parallel connection, hence their equivalent resistance is given by,
Effective resistance,
The equivalent resistance,
Replacing these two resistors in the circuit,

Now the effective resistance of these three resistors will be the total equivalent resistance of the entire circuit.
Since the three resistors are in series,
The equivalent resistance of the circuit,
The Potential difference is
Current through the source can be obtained by applying Ohm’s law
Given that
The current through the source,
To find the current through each branch, we have to go through the simplified circuit in the reverse order.

The current through
The current through the next

The
The current through the next
In the same way, the current through the next

There are again two branches with equal resistance, the current will again split into half,
The current through the next
The current through the next
The current through the next
Applying Kirchhoff’s law from A to B,
From the above equation, we get
The correct answer is (A), The current in the
Note:To find the equivalent resistance of several resistors that are connected in series we take the individual sum of resistances. In a series combination, the equivalent resistance will be higher than the value of the highest resistance in the circuit. On the other hand, in parallel combination, the effective resistance will be less than the lowest value of resistance in the circuit.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Wheatstone Bridge - Working Principle, Formula, Derivation, Application

Young's Double Slit Experiment Step by Step Derivation

JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

Chemical Properties of Hydrogen - Important Concepts for JEE Exam Preparation

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Degree of Dissociation and Its Formula With Solved Example for JEE

Displacement-Time Graph and Velocity-Time Graph for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

JEE Advanced 2025 Notes

Electrical Field of Charged Spherical Shell - JEE

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025




