
A body of mass 6 kg rests in limiting equilibrium on an inclined plane whose slope is
Answer
156.3k+ views
Hint: As the slope is inclined a component of its weight will be pulling it downwards, as the body is at rest friction force will be acting on it. We can find this force and use it so find how much extra force is needed to hold the body at rest when inclination is increased.
Formula Used: Newton’s second law of motion F = ma, m is mass & a is acceleration maximum frictional force possible =
Complete step-by-step answer:
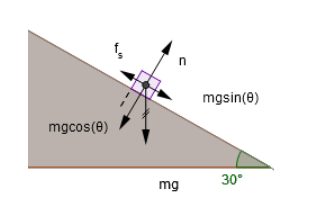
Here, m is mass of body, g is acceleration due to gravity, f is maximum sliding friction, n is normal from incline,
When the angle of incline is
Now since the body is in limiting equilibrium i.e. just about to slide then friction will have its maximum possible value. So,
Also the body is at rest along the incline. Hence net force along the incline is zero. So,
Putting value of f from equation (1) and putting given value
Here, m is mass of body, g is acceleration due to gravity, f is maximum sliding friction, n is normal from incline,
When the angle of incline is
The maximum possible friction force is
Component of force due to gravity along the incline is
So net force along the incline is down the incline with magnitude,
Hence an extra force of
So the correct option is B.
Note: Our final answer was
Also the mentioned term “limiting equilibrium“ tells that the body is in equilibrium but just about to move.
Formula Used: Newton’s second law of motion F = ma, m is mass & a is acceleration maximum frictional force possible =
Complete step-by-step answer:

Here, m is mass of body, g is acceleration due to gravity, f is maximum sliding friction, n is normal from incline,
When the angle of incline is
Now since the body is in limiting equilibrium i.e. just about to slide then friction will have its maximum possible value. So,
Also the body is at rest along the incline. Hence net force along the incline is zero. So,
Putting value of f from equation (1) and putting given value
Here, m is mass of body, g is acceleration due to gravity, f is maximum sliding friction, n is normal from incline,
When the angle of incline is
The maximum possible friction force is
Component of force due to gravity along the incline is
So net force along the incline is down the incline with magnitude,
Hence an extra force of
So the correct option is B.
Note: Our final answer was
Also the mentioned term “limiting equilibrium“ tells that the body is in equilibrium but just about to move.
Recently Updated Pages
JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

Chemical Properties of Hydrogen - Important Concepts for JEE Exam Preparation

JEE Energetics Important Concepts and Tips for Exam Preparation

JEE Isolation, Preparation and Properties of Non-metals Important Concepts and Tips for Exam Preparation

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Uniform Acceleration

Degree of Dissociation and Its Formula With Solved Example for JEE

Displacement-Time Graph and Velocity-Time Graph for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry




