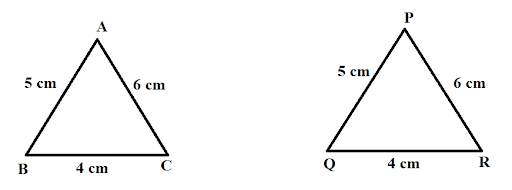Revision Notes for CBSE Class 7 Maths Chapter 7 - Free PDF Download





















FAQs on Congruence of Triangles Class 7 Notes CBSE Maths Chapter 7 (Free PDF Download)
1. Are NCERT Solutions for Class 7 Maths Chapter 7 important from an exam point of view?
Yes, NCERT solutions of Class 7 Maths Chapter 7 is important from an exam point of view as these questions will make your exam preparation easier and you will get accurate solutions to all the questions. You can prepare from those questions while you are revising for your exam and cross-check all the solutions so that you do not write wrong answers in your exams. The solutions are accurate and you can rely on them.
2. Give me a summary on NCERT Solutions for Class 7 Maths Chapter 7?
This chapter is a congruence of triangles. It has two exercises that are related to different topics. The main topics include:
Plain figure’s congruence
Congruence inline segments
Congruence in triangle and angles
Right-angled triangle’s congruence
For more information, visit Vedantu’s official website. There you will find the solved answers which will be helpful in your exams. These solutions will cover all the important topics which are curated together from the exam's point of view. You can download these questions and save them on your PC for future reference.
3. What will students learn after solving questions from this chapter?
Students will appreciate the usefulness of superposition after going through the fundamental ideas of congruence outlined in this chapter. Superposition is a useful means of assessing whether any two-plane figures are congruent. Students can try applying the congruence requirements for line segments, angles, and triangles to different planar figures after learning about them. When establishing that two triangles are congruent, there are a few theorems that must be taken into account. In NCERT solutions Class 7 maths chapter 7, they are logically proven and discussed.
4. What are the criteria for the congruence of triangles according to Class 7?
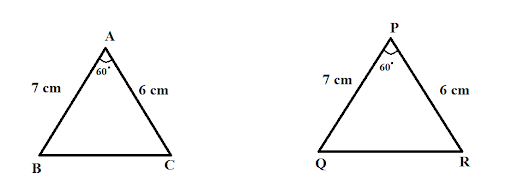
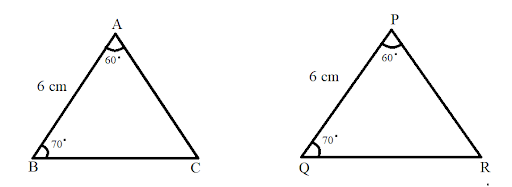
They are called congruent when the three angles of one triangle are equal to the three angles of the other triangle. This is the SSS rule. When two angles and one side of the triangle are similar to the other triangle then it is called the ASA rule. When two angles and other angles of one triangle are equal to the other sides of another triangle then it is called the SAS rule.
5. Is it necessary for me to go over all of the questions in NCERT Solutions Class 7 Maths Congruence of Triangles?
The NCERT Solutions Class 7 Maths Congruence of Triangles properly explains the triangles, which are the beginning of the geometry subject. As a result, students will benefit from regular practice of the exercise problems to have a clear grasp of all of the principles and properties of triangles, which will ensure a solid basis for knowledge of future geometry topics. The CBSE board always recommends these answers to students for test preparation because they are simple to obtain and understand.