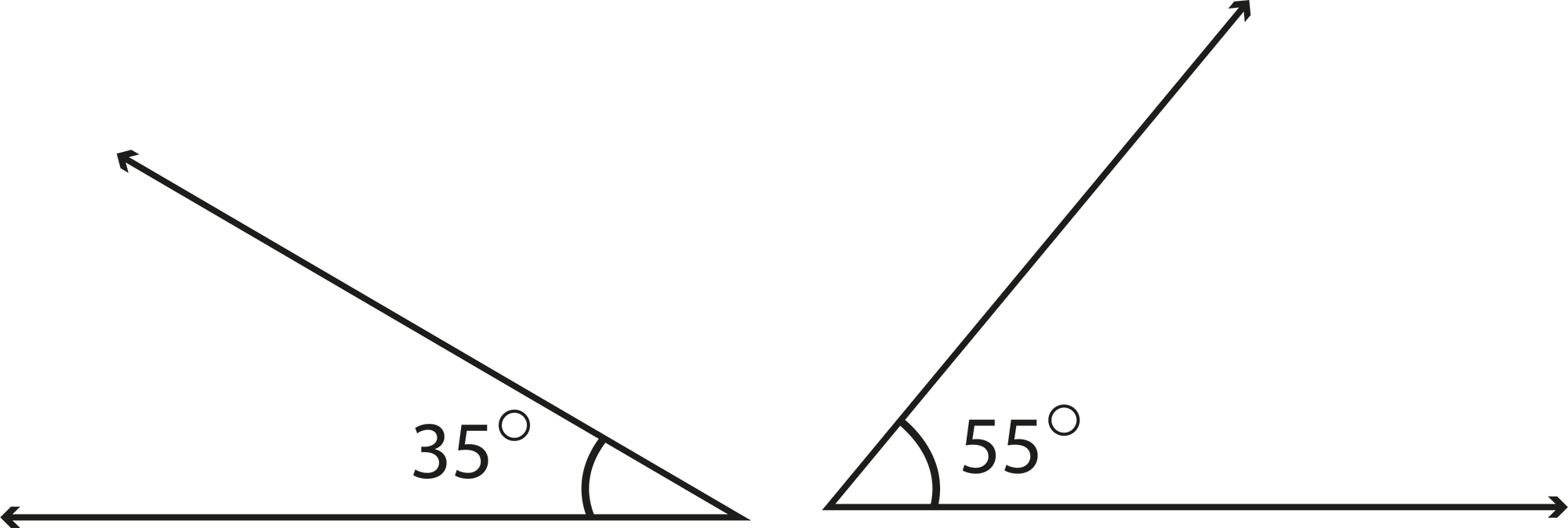Revision Notes for CBSE Class 7 Maths Chapter 5 - Free PDF Download
FAQs on Lines and Angles Class 7 Notes CBSE Maths Chapter 5 (Free PDF Download)
1. Where will I Find the Best Class 7 Revision Notes Lines and Angles?
By referring to Revision Notes of Class 7 Maths Chapter 5 created by the subject specialist at Vedantu, you will be able to get the last moment run down preparation in a place. Students will have no doubt and be able to rest anxiety-free before the final examinations as you will be fully prepared in advance using these revision notes and sample papers.
Also, the CBSE Class 7 Revision Notes Lines and Angles PDF is simple to read and includes all the material, clearly described in the shortest possible manner. You can also use the sample papers that Vedantu provides for free in a PDF format for the CBSE Class 7 along with the Class 7 Maths Notes on Lines and Angles. Together, students will be exam ready to answer every type of question, both subjective and objective and targets for the best in their school examination.
2. What are lines for Class 7?
As discussed in the chapter, a line is a straight, one-dimensional figure that has no beginning and no end. In other words, when the endpoints of a line segment are extended endlessly, a line is formed. The line can also be interpreted as the shortest distance between any two points in a plane. The line is the most basic figure in geometry, thus, to understand geometry, one first has to be thorough with the basics of the line.
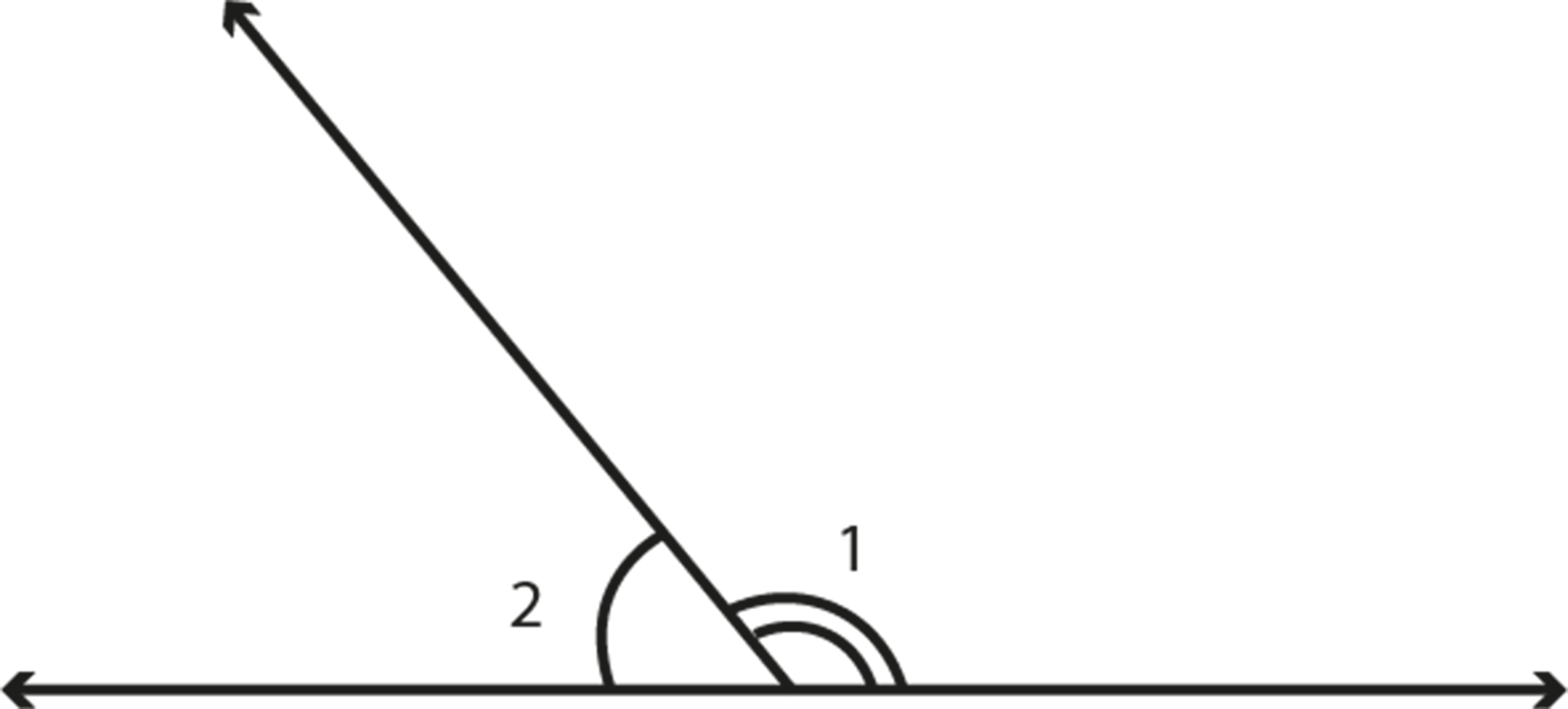
3. What are angles for Class 7 Maths?
As discussed in Class 7 Maths, Chapter 5 - Lines and Angles, an angle is the geometrical figure made up of two rays that meet at a common, fixed point. Ray is, in simple words, a line that has a fixed point on one end. Instead of extending endlessly on both sides, a ray has a fixed point, and the other end extends endlessly. An angle is calculated in degrees and can be measured with the help of a protractor.
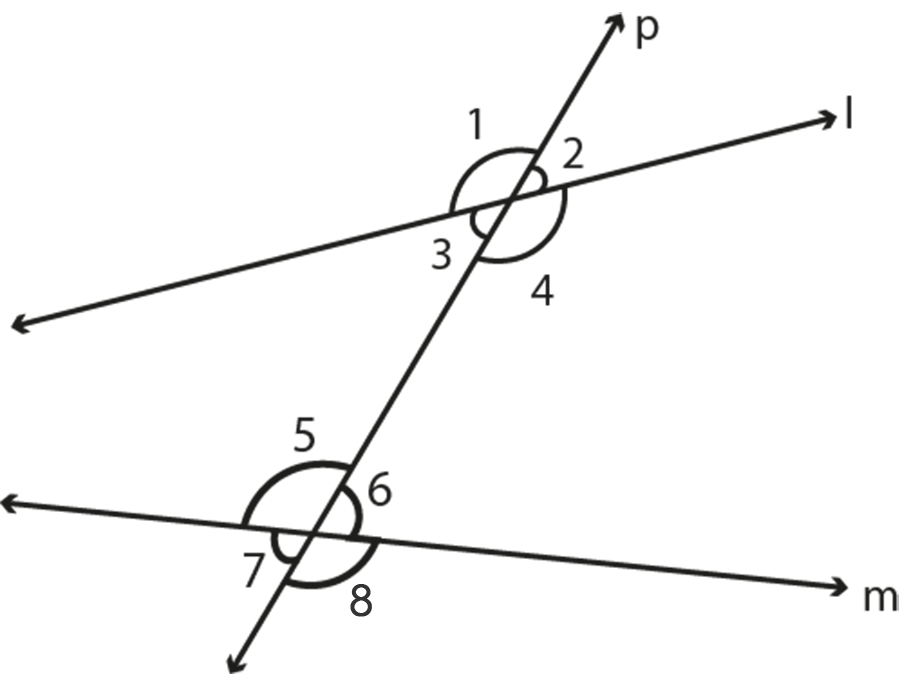
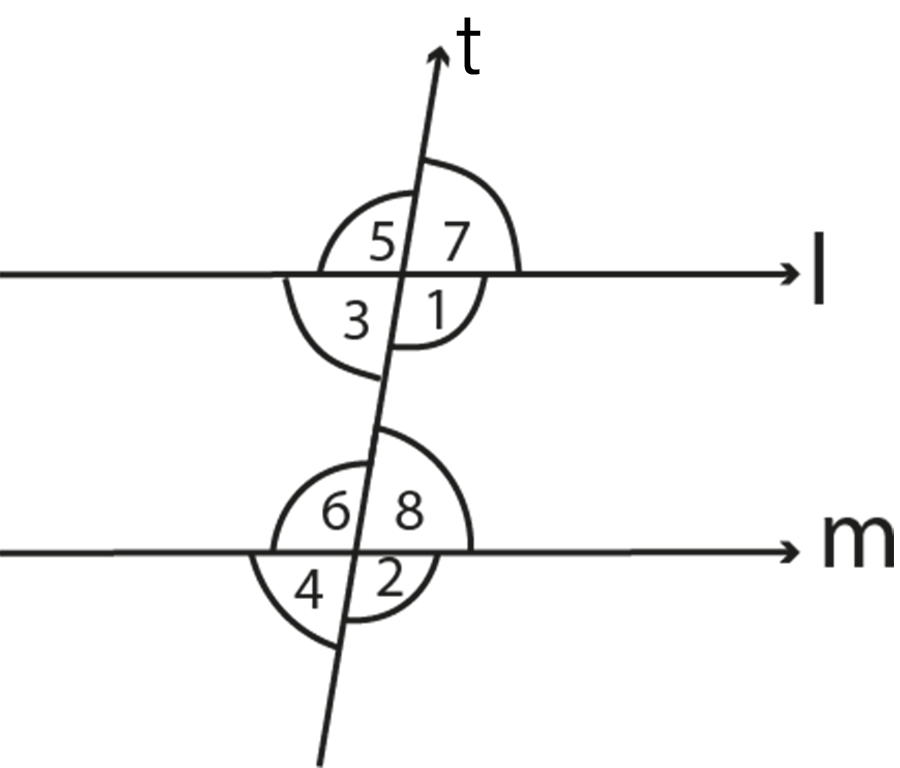
4. What is the corresponding angle for Class 7?
Corresponding angles can be understood with the help of an example. Let us suppose that there is a pair of parallel lines in a plane and they are cut by a transversal. At the place where the transversal passes through these lines, angles are being formed. The angles that are on the same side of the transversal, i.e, the angles that are formed in the corresponding or the matching corners with the transversal, are referred to as the corresponding angles.
5. How Many Questions are there in NCERT Solutions Class 7 Maths Chapter 5 Lines and Angles?
Vedantu's NCERT Solutions and Vedantu's Revision Notes for Class 7 Maths, Chapter 5- 'Lines and Angles,' have covered all the questions and topics of the chapter. This study material will help you find answers to each question given in the Maths NCERT book. By referring to Vedantu's study materials, you can simplify your Maths examination preparation. The study material provided by Vedantu is highly credible, well-structured, easy-to-understand and based on the latest CBSE syllabus, exam pattern and marking scheme.
6. What are the important topics covered in NCERT Revision Notes Class 7 Maths Chapter 5?
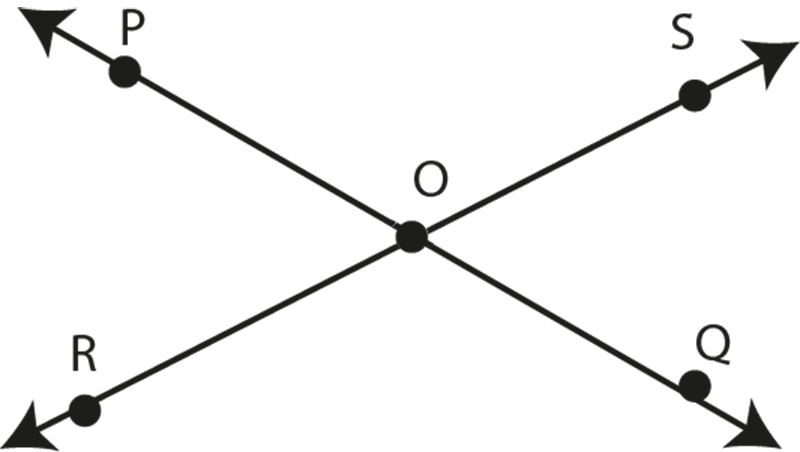
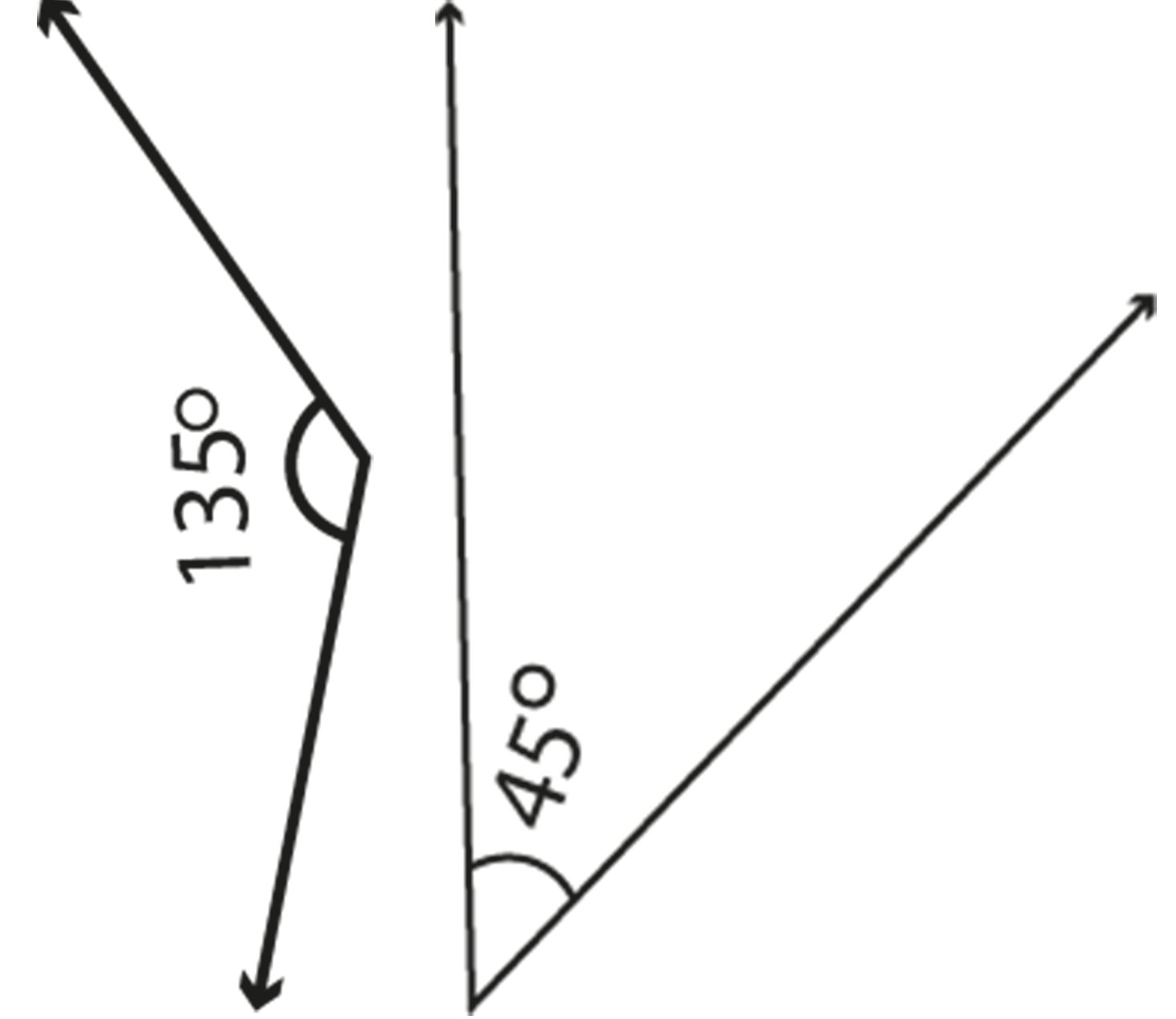
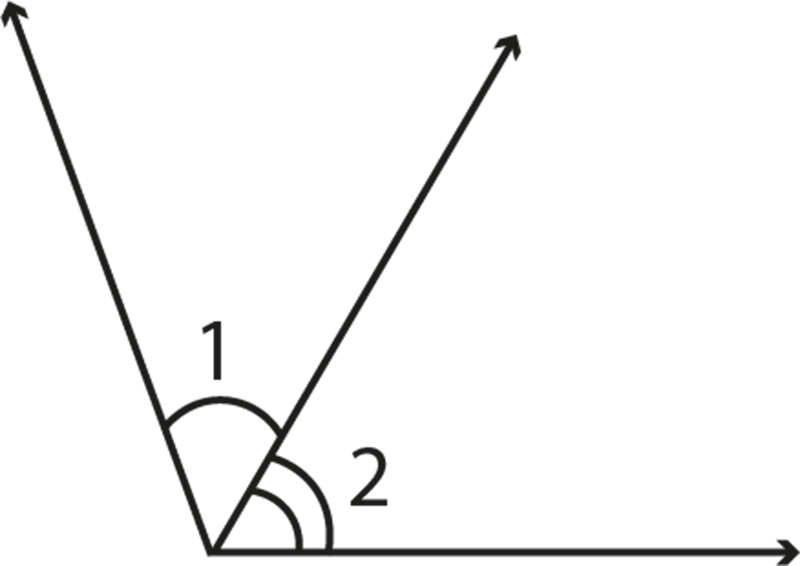
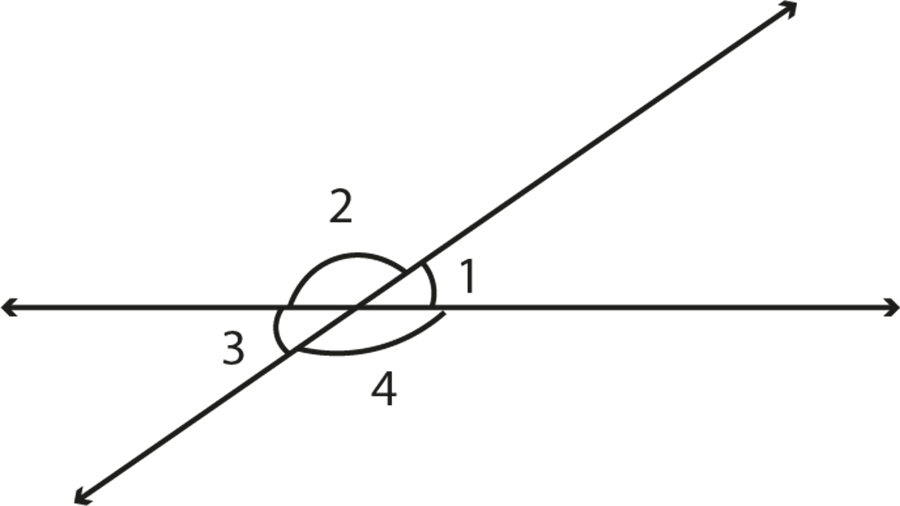
Class 7 Maths, Chapter 5- 'Lines and Angles' gives students an introduction to the world of Geometry. The chapter begins with basic concepts such as a line, ray, line segment and angles. Under the topic of angles, different types of angles discussed are- corresponding angles, alternate angles (alternate interior and alternate exterior angles). Next, questions are given based on supplementary angles, adjacent angles, linear pair, vertically opposite angles and Interior angles on the same side of the transversal. The chapter then moves on to discuss the basic properties of the triangle.
If you want to understand these concepts more accurately and to make detailed notes, then go through the given link CBSE Class 7 Math Chapter 5 notes .This link will redirect you to the official website of Vedantu where you can access the content related to Chapter 5 for free. Additionally, you can also download its PDF if you want to study offline.