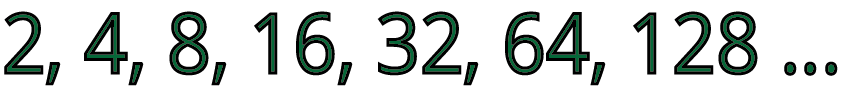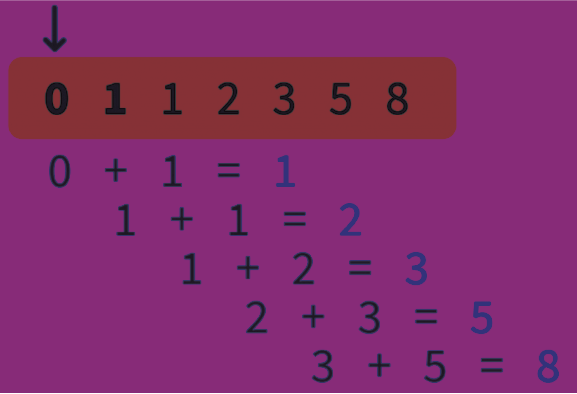Class 5 Maths Chapter 7 Summary Notes PDF Download
FAQs on Can You See The Pattern Class 5 Maths Chapter 7 CBSE Notes - 2025-26
1. How to learn and revise Class 5 Maths Can You See The Pattern?
Your first step is to follow the classroom sessions. Proceed to learn the concepts covered in this chapter and solve the exercise problems. Download and refer to the NCERT Solutions for Class 5 Chapter 7 and find out how the experts have solved them. Move on to the worksheets provided with the revision notes. Learn where you need to improve yourself to solve the questions. Once done, use the revision notes to revise the concepts before your exam.
2. Will I be able to learn the rules to make patterns?
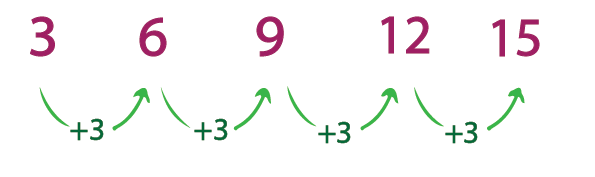
Yes. Follow how the experts have explained this rule in the revision notes. Relate the explanations given with that of the textbook. It will help you find out the patterns easily and answer the questions.
3. How can I find the patterns in number hexagons?
Check the shape of the boxes and their positions. You will find three numbers on each side. Use mathematical operations to find their relations. This is how you can find a pattern.