Answer
35.4k+ views
Hint We know that PE is the amount of energy started at the highest point.
Here, two springs are converted in series
Therefore, using $P.E=\dfrac{1}{2}{{K}_{2}}$
Complete Step by Step Solution
$2:1$
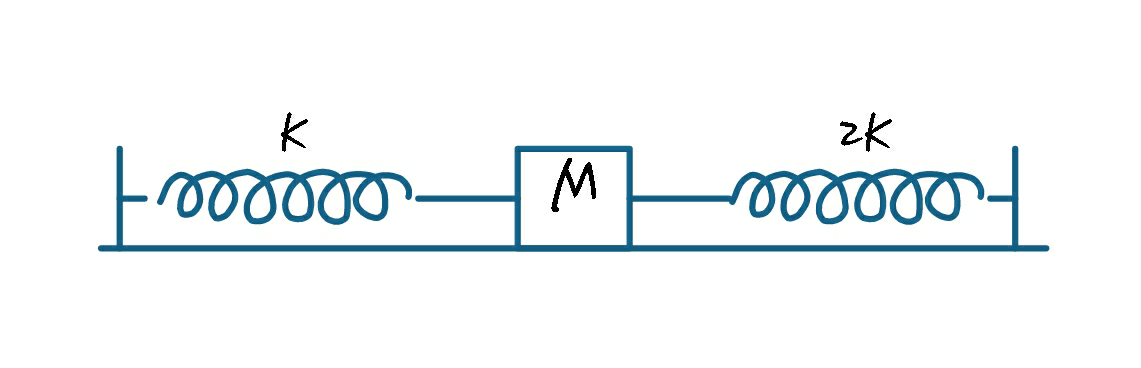
For spring ${{S}_{1}}\text{ the spring constant is }K$
For spring${{S}_{2}}$ the spring constant is $2K$
Now, applying the potential energy formula for spring ${{S}_{1}}$
i.e. $P{{E}_{1}}=\dfrac{1}{2}K{{r}^{2}}$ …… (1)
Similarly, we use the above formula for spring ${{S}_{2}}$
i.e.$P{{E}_{2}}=\dfrac{1}{2}2K\text{ }{{x}^{2}}$ …… (2)
Now, dividing equation (2) by equation (1)
We get $\dfrac{P{{E}_{2}}}{P{{E}_{1}}}=\dfrac{1}{2}K{{x}^{2}}\times \dfrac{2}{\left( 2K \right){{x}^{2}}}$
Now, cancelling all the common factors in the above equation we get
$\dfrac{P{{E}_{2}}}{P{{E}_{1}}}=\dfrac{1}{2}$
Or for simplicity we can write this as also
$\dfrac{P{{E}_{2}}}{P{{E}_{1}}}=\dfrac{1}{2}$
Therefore, the ratio of the two potential angles is $2:1$
Note Above discussion of Potential Energy that is stored in the spring can be found out using $\dfrac{1}{2}k{{x}^{2}}$formula in which$k=\text{Spring Constant}$.
In this case, we reciprocate this energy with respect to the other.
Here, two springs are converted in series
Therefore, using $P.E=\dfrac{1}{2}{{K}_{2}}$
Complete Step by Step Solution
$2:1$
For spring ${{S}_{1}}\text{ the spring constant is }K$
For spring${{S}_{2}}$ the spring constant is $2K$
Now, applying the potential energy formula for spring ${{S}_{1}}$
i.e. $P{{E}_{1}}=\dfrac{1}{2}K{{r}^{2}}$ …… (1)
Similarly, we use the above formula for spring ${{S}_{2}}$
i.e.$P{{E}_{2}}=\dfrac{1}{2}2K\text{ }{{x}^{2}}$ …… (2)
Now, dividing equation (2) by equation (1)
We get $\dfrac{P{{E}_{2}}}{P{{E}_{1}}}=\dfrac{1}{2}K{{x}^{2}}\times \dfrac{2}{\left( 2K \right){{x}^{2}}}$
Now, cancelling all the common factors in the above equation we get
$\dfrac{P{{E}_{2}}}{P{{E}_{1}}}=\dfrac{1}{2}$
Or for simplicity we can write this as also
$\dfrac{P{{E}_{2}}}{P{{E}_{1}}}=\dfrac{1}{2}$
Therefore, the ratio of the two potential angles is $2:1$
Note Above discussion of Potential Energy that is stored in the spring can be found out using $\dfrac{1}{2}k{{x}^{2}}$formula in which$k=\text{Spring Constant}$.
In this case, we reciprocate this energy with respect to the other.
Recently Updated Pages
To get a maximum current in an external resistance class 1 physics JEE_Main

f a body travels with constant acceleration which of class 1 physics JEE_Main

A hollow sphere of mass M and radius R is rotating class 1 physics JEE_Main

If the beams of electrons and protons move parallel class 1 physics JEE_Main

Two radioactive nuclei P and Q in a given sample decay class 1 physics JEE_Main

If a wire of resistance R is stretched to double of class 12 physics JEE_Main

Other Pages
Oxidation state of S in H2S2O8 is A 6 B 7 C +8 D 0 class 12 chemistry JEE_Main

Two identical charged spheres suspended from a common class 12 physics JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main

Explain the construction and working of a GeigerMuller class 12 physics JEE_Main

In a CO molecule the distance between C mass12 amu class 12 physics JEE_Main

A gas is compressed isothermally to half its initial class 11 physics JEE_Main