Electric Field due to Infinite Linear Charge and Cylinders for JEE

The field of electrostatics had the main purpose of explaining the field created by a charged particle known as an electric field. When a charge is sitting in a space, field lines emanate from it which is collectively given the name of an electric field capable of influencing other charges nearby. 

Depending on the nature of the charge, i.e., positive or negative, the field line directions are also different. In positive charge, the field lines go out while in negative charge, the field lines are directed inwards as shown in the figure below. The electric field can exert electrostatic force on other static or moving charges in the vicinity of the test charge. This force can be either repulsive or attractive depending on the nature of charges. 

Gauss’s Law: Formula and Derivation

In electrostatics, Gauss’s Law is a saviour when it comes to calculating the electric field of a symmetric charge distribution. By symmetric charge distribution, it is meant that the charge is spread out in a manner that resembles geometric figures we all are familiar with. This law takes into account the charge and the electric flux through a surface and is generally applicable for any type of charge distribution but preferably the symmetric ones. 

Its importance is so immense that it has been used as one of Maxwell's equations of electromagnetism. The statement is that the complete electric flux magnitude through a symmetric surface is equal to the total enclosed charge in that surface divided by the material permittivity. Electric flux is nothing but the field lines passing through a surface and is the product of the electric field with the area of the concerned surface.

Mathematically,

$\nabla .\vec{E}=\dfrac{\rho }{{{\varepsilon }_{0}}}$       (Differential form)

$\oint{\vec{E}\cdot d\vec{a}}=\dfrac{{{Q}_{enc}}}{{{\varepsilon }_{0}}}$   (Integral form)

In the case of calculating the electric field using Gauss’s law, one has to assume a hypothetical Gaussian surface around the charge to enclose it completely. If the field is uniform, then the calculation is easier. We only have to find the area of the symmetric figure. 

What is Linear Charge Density?

Charge density can be of different nature depending on the surface on which charge is getting distributed. The charge density is the measure of how much charge is contained in that particular field. So, based on this argument, charge density can be linear, surface, and volume. Linear means anything that is in a line. So, linear charge density is the charge distributed in a line per unit length of the line. 

Mathematically,

$\lambda \ \text{=}\ \dfrac{\text{Q}}{\text{L}}$ 

Here, Q is the total charge distributed on the line and L is the total length of the line. 

Therefore, $\lambda $denotes the charge per unit length or linear charge density. The S.I. the unit of $\lambda $ is coulomb/(metre)2.

Electric Field due to a Linear Charge Distribution

Consider a straight infinite conducting wire with linear charge density of $\lambda $. Consider a point P at a distance r from the wire in space measured perpendicularly. The wire cross-section is cylindrical in nature, so the Gaussian surface drawn is also cylindrical in nature. The area of a cylindrical surface is equal to $\text{S}\ \text{=}\ \text{2 }\!\!\pi\!\!\text{ rL}$. 

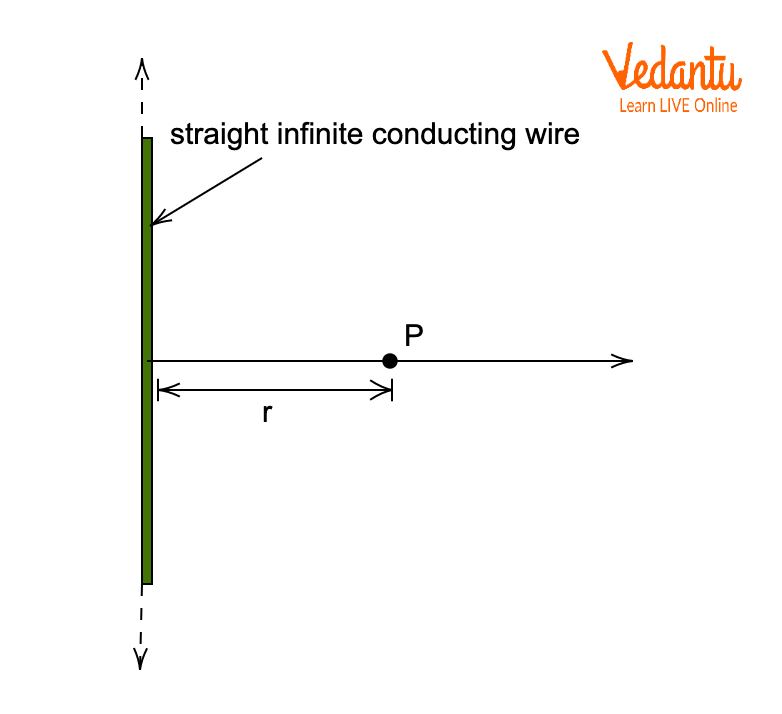
Electric Field due to a Linear Charge Distribution

The total amount of positive charge enclosed in a cylinder is $Q=\lambda \text{L}$.

Our goal is to calculate the total flux coming out of the curved surface and the two flat end surfaces numbered 1, 2, and 3.

Flux through surface 1 is

${{\text{ }\!\!\varphi\!\!\text{ }}_{\text{1}}}\ \text{=}\ \text{0}$ 

Flux through surface 2 is

${{\text{ }\!\!\varphi\!\!\text{ }}_{2}}\ \text{=}\ \text{0}$

Flux through surface 3 is

${{\text{ }\!\!\varphi\!\!\text{ }}_{\text{3}}}\ \text{=}\ \ \text{Ecos }\!\!\theta\!\!\text{ }\times \text{S}$

The field is uniform and its magnitude is E, so, the flux becomes,

${{\text{ }\!\!\varphi\!\!\text{ }}_{\text{3}}}\ \text{=}\ \ \text{E }\!\!\times\!\!\text{ 1 }\!\!\times\!\!\text{ 2 }\!\!\pi\!\!\text{ rL}$

Therefore, the total electric flux is calculated as

$\text{ }\!\!\varphi\!\!\text{ }\ \text{=}\ {{\text{ }\!\!\varphi\!\!\text{ }}_{\text{1}}}\ \text{+}\ {{\text{ }\!\!\varphi\!\!\text{ }}_{\text{2}}}\ \text{+}\ {{\text{ }\!\!\varphi\!\!\text{ }}_{\text{3}}}$

$\text{ }\!\!\varphi\!\!\text{ }\ \text{=}\ \text{2 }\!\!\pi\!\!\text{ rLE}$  

Now, from Gauss’s Law, this flux is equal to the net closed charge divided by the permittivity of the material. So, 

$\text{ }\!\!\varphi\!\!\text{ }\ \text{=}\ \dfrac{\lambda \text{L}}{{{\text{ }\!\!\varepsilon\!\!\text{ }}_{\text{0}}}}$ 

Substitute the value of the flux in the above equation and solving for the electric field E, we get

$\text{2 }\!\!\pi\!\!\text{ rLE}=\dfrac{\lambda \text{L}}{{{\text{ }\!\!\varepsilon\!\!\text{ }}_{\text{0}}}} $

$\text{E}=\dfrac{1}{\text{2 }\!\!\pi\!\!\text{ }{{\text{ }\!\!\varepsilon\!\!\text{ }}_{\text{0}}}}\dfrac{\lambda }{\text{r}}$ 

This is the electric field intensity (magnitude) due to a line charge density using a cylindrical symmetry.

Conclusion

The following points can be concluded for the topic:

• The density of electric field lines tells us about the electric field intensity at that point.

• Electric field is the space where charged particles experience force of attraction or repulsion due to a source charge.

• Linear charge density is the charge distributed per unit length along a line.

• According to Gauss’s Law, the total flux due to a charge through a surface is equal to the total charge enclosed in the closed surface divided by the permittivity of the medium.

• The electric field due to a line charge distribution makes use of a cylindrical Gaussian surface. The magnitude of the electric field at a point in space which is at a distance r from the wire is $\text{E}=\dfrac{1}{\text{2 }\!\!\pi\!\!\text{ }{{\text{ }\!\!\varepsilon\!\!\text{ }}_{\text{0}}}}\dfrac{\lambda }{\text{r}}$.