Square Root of 200: An Introduction
The square root of a number can be in the form of a natural number or decimal, or radical. It depends upon the given number. If it is a perfect square, the square root will be a natural number; if the given number is not a perfect square, the square root will be a decimal or radical.
The square root of 200 will be a decimal or radical, as 200 is not a perfect square. We will use long division and prime factorisation to find the square root of 200.
Value of Root 200
The square root of 200 is a number when it is multiplied by the number itself gives us 200. The symbol of representation of the square root is “\[\sqrt {} \]”, so we can represent the square root of 200 as \[\sqrt {200} \].
Let’s take the square root of 200 as “x”
Hence, \[\sqrt {200} = \sqrt {x \times x} \]
The value of x or we can say the square root of 200, is 14.1421.
In the radical form, we can write the square root of 200 as \[10\sqrt 2 \], which cannot be reduced further.
In the exponential form, we can write the square root of 200 as \[{200^{\dfrac{1}{2}}}\]
Square root of 200 is an irrational number as it cannot be expressed in the form of the \[\dfrac{p}{q}\].
Prime Factorization Method to Find the Square Root of 200
In Prime factorization method first we have to find the prime factors of 200 and then the square root of 200.
Prime factors of \[100 = 2 \times 2 \times 5 \times 5\]
and prime factors of square root of \[\sqrt {100} = \sqrt {2 \times 2 \times 2 \times 5 \times 5} \]
According to prime factorisation method , the square root of a number is done by dividing the factors in pairs and selecting one factor per pair, i.e., for our case,
\[\sqrt{100} = 2 \times 5 = 10\]
Long Division Method to Find Square Root of 200
Now we will do the long division method to find the square root of 200
Step 1: First we will write 200 in decimal form. We will add 6 zeros after the decimal point. Then pair the numbers by putting the bar on the top of the numbers from left to right.
\[\overline 2 \overline {00} .\overline {00} \overline {00} \overline {00} \]
Step 2: Now we will divide 2 by a number such that the product of the number with itself is less than 2. We know \[1 \times 1 = 1\] which is less than 2. Thus the quotient is 1 and the remainder is 1.
Step 3: Bring down 00. Our new dividend is 100. The divisor will be the sum of 20 times the quotient and a number. The number will be such that the product of \[\left( {20 \times 1} \right) + number\] and the number must be less than 100. \[24 \times 4 = 96 < 100\]. The new quotient is 14 and the remainder is 4.
Step 4: Now we will put the decimal point in the quotient and bring down 00. Our new dividend is 400. The divisor will be the sum of 20 times the quotient and a number. The number will be such that the product of \[\left( {20 \times 14} \right) + number\] and the number must be less than 400. \[281 \times 1 = 281 < 400\]. The new quotient is 14.1 and the remainder is 119.
Step 5: Bring down 00. Our new dividend is 11900. The divisor will be the sum of 20 times the quotient (don’t consider decimal point) and a number. The number will be such that the product of \[\left( {20 \times 141} \right) + number\]and the number must be less than 11900. \[2824 \times 4 = 11296 < 11900\]. The new quotient is 14.14 and the remainder is 604.
Step 6: Bring down 00. Our new dividend is 60400. The divisor will be the sum of 20 times the quotient (don’t consider decimal point) and a number. The number will be such that the product of \[\left( {20 \times 1414} \right) + number\] and the number must be less than 60400. \[28282 \times 2 = 11296 < 11900\]. The new quotient is 14.142 and the remainder is 3836.
The square root of 200.
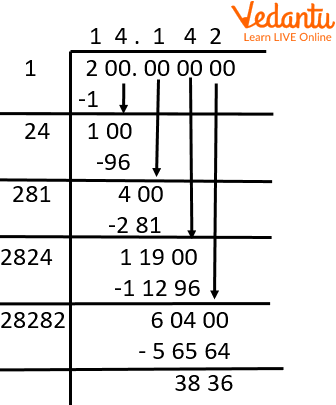
Therefore the Value of Square Root 200 is 14.142 (Approx.)
Find the Square Root of 200 with Approximation Method
Finding square root without actual multiplication is also called an approximation method to find square root. Let’s take two consecutive perfect square numbers in which the given number 200 lies. The two numbers will be 196 \[\left( {{{14}^2}} \right)\]and 225 \[\left( {{{15}^2}} \right)\].
Clearly, the whole number part of the square root of 200 is 14 and for the decimal part of the square root, we will use the below formula
\[\dfrac{{{\rm{Given}}\,{\rm{number}} - {\rm{smaller}}\,{\rm{perfect}}\,{\rm{square}}\,{\rm{number}}}}{{{\rm{greater}}\,{\rm{perfect}}\,{\rm{square}}\,{\rm{number}} - {\rm{smaller}}\,{\rm{perfect}}\,{\rm{square}}\,{\rm{number}}}}\]
\[ = \dfrac{{200 - 196}}{{225 - 196}}\]
\[ = \dfrac{4}{{29}}\]
=0.1379
Hence we get the square root of 200 is 14.1379 without actual multiplication or Approximation Method.
Repetitive Subtraction Method to Find the Square Root of 200.
In this method, we subtract odd numbers from 200. This process will continue until we get 0.
1. 200 – 1 =199
2. 199 – 3 =196
3. 196 – 5 =191
4. 191 – 7 = 184
5. 184 – 9 = 175
6.175 – 11=164
7. 164 – 13 = 153
8. 153 – 15 = 138
9. 138 – 17 =121
10. 121 – 19=102
11. 102– 21 = 81
12. 81 – 23 = 58
13. 58 – 25 =33
14. 33 – 27 = 6.
We do not get 0 in this process. Since it ends at the 14th step, thus the square root of 200 lies between 14 and 15.
Square of 200
Square of a number can be found by multiplying the number itself. For example, If have to find the square of a number ‘\[x\]’, the square will be \[{x^2}\].
So if we have to find the square of 200 it will \[{200^2}\]and by calculating we get 40,000.
\[200 \times 200 = 40000\]
Some Interesting Facts
If a number is not a perfect square, it’s square root will be an irrational number.
Solved Examples
1. Evaluate square of \[10\sqrt 2 \]
Solution: It can be calculated by multiplying the given number itself
\[10\sqrt 2 \times 10\sqrt 2 = 100 \times 2 = 200\]
2. Evaluate \[100 + \sqrt {200} \]
Solution: To simplify the question first we find the square root of 200 and then add the 100.
As we know the square root of 200 is 14.1421, therefore the required value will be
\[100 + \sqrt {200} = 100 + 14.1421\]
= 114.1421
Hence the value of \[100 + \sqrt {200} \] is 114.1421.
3. Do you know the square root of 145 lies between two consecutive perfect square numbers?
Solution: We know that \[12^2=144\] and \[13^2=169\], 145 lies between 144 and 169. Thus the square root 145 lies between 12 and 13.
Practices Problem:
1. Find the square root of 224.
Answer: 14.9667
2. Find the numbers between which the square root of 230 lies.
Answer: 15 and 16.
FAQs on Square Root of 200: A Detailed Explanation
1. Check whether the statement is true or false.
The square of the square root of a number is the same square root of the square of that number.
Solution: Assume the number be a.
Square root of a is \[\sqrt a \] and the square of a is \[{a^2}\].
The square of \[\sqrt a \] is \[{\left( {\sqrt a } \right)^2} = a\].
The square root of \[{a^2}\] is \[\sqrt {{a^2}} = a\].
Therefore the given statement is true.
2. If a natural number multiplies by a radical, then find the type of the resultant number.
Solution: A radical is always an irrational number. A natural number is a rational number. The product of a rational and irrational is always an irrational number.
3. What should be subtracted from 200 to get the greatest perfect square root?
Solution: Using long division
1 4 | |
1 | 2 00 .00 00 1 |
24 | 1 00 96 |
4 |
The remainder is 4. Thus 4 should be subtracted from 200 to get a perfect square root.
