
Wind is blowing west to east along two parallel railway tracks. Two trains moving with the same speed in opposite directions have the steam track of one double that of the other. The speed of each train is
(A) Equal to that of the wind
(B) Three times that of the wind
(C) Double that of the wind
(D) Half that of the wind
Answer
154.5k+ views
Hint Draw the length of the first steam track as
Complete Step by step answer
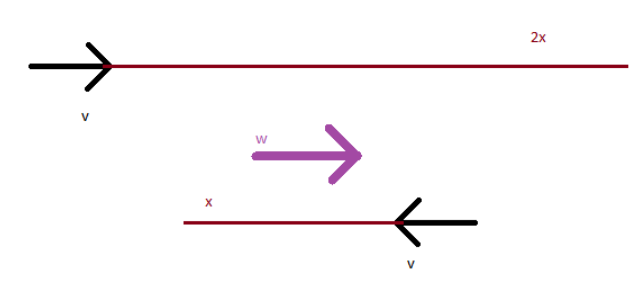
As shown in the above diagram, the steam track length is drawn in brown.
We will consider the speed of the trains as
The wind will be supporting the steam to spread and is shown in purple in the diagram.
So, from the first diagram, we get the equation as
Here we assume that the steam length per unit time is equal to the speed of the train and the speed of the wind combined, since both are assisting it.
From the second diagram, we get the equation as
Here we assume that the steam length per unit time is equal to the speed of the train minus the speed of the wind combined, since the length direction is in the direction of the velocity of the train. In this assumption, it is noted that the wind speed is less than the speed of the train as per the diagram, which can be otherwise.
From the above two equations, we get
Thus, the speed of the train which is
Therefore, the correct answer is option (2) Three times that of the wind.
Note: Here we have assumed simply that the steam track is of a certain length and that the wind and train speeds are acting for or against changing the length of the steam track. Therefore the simple equation of speed has been used.
Complete Step by step answer

As shown in the above diagram, the steam track length is drawn in brown.
We will consider the speed of the trains as
The wind will be supporting the steam to spread and is shown in purple in the diagram.
So, from the first diagram, we get the equation as
Here we assume that the steam length per unit time is equal to the speed of the train and the speed of the wind combined, since both are assisting it.
From the second diagram, we get the equation as
Here we assume that the steam length per unit time is equal to the speed of the train minus the speed of the wind combined, since the length direction is in the direction of the velocity of the train. In this assumption, it is noted that the wind speed is less than the speed of the train as per the diagram, which can be otherwise.
From the above two equations, we get
Thus, the speed of the train which is
Therefore, the correct answer is option (2) Three times that of the wind.
Note: Here we have assumed simply that the steam track is of a certain length and that the wind and train speeds are acting for or against changing the length of the steam track. Therefore the simple equation of speed has been used.
Recently Updated Pages
JEE Main 2022 (June 29th Shift 2) Maths Question Paper with Answer Key

JEE Main 2023 (January 25th Shift 1) Maths Question Paper with Answer Key

JEE Main 2022 (July 29th Shift 1) Maths Question Paper with Answer Key

JEE Main 2022 (July 26th Shift 2) Chemistry Question Paper with Answer Key

JEE Main 2022 (June 26th Shift 2) Maths Question Paper with Answer Key

JEE Main 2022 (June 29th Shift 1) Physics Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Degree of Dissociation and Its Formula With Solved Example for JEE

Displacement-Time Graph and Velocity-Time Graph for JEE

Electrical Field of Charged Spherical Shell - JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line




