
The number of real roots of the equation ${\log _e}x + ex = 0$
$
{\text{A}}{\text{. 0(zero)}} \\
{\text{B}}{\text{. 1}} \\
{\text{C}}{\text{. 2}} \\
{\text{D}}{\text{. 3}} \\
$
Answer
181.8k+ views
Hint: -You can solve this question by two method first simply solve and get value of x and check whether it is real or not and we can see there is only two terms so, put one term one side of equal to and another term on opposite side of equal to and draw graph of both to solve further.
Complete step-by-step solution -
From given
${\log _e}x + ex = 0$
You can write it as
${\log _e}x = - ex$
Now you have to draw the graph of $y = {\log _e}x$ and graph of y = -ex .
You should have knowledge of graphs of logarithmic function and also knowledge of graphs of straight line. Now you have to check the cutting points of both the graphs.
So, you can see in diagram graph cuts each other at only one point so there is only one real root of the equation.
Hence option B is the correct option.
Note: -Whenever you get this type of question the key concept of solving is you have to draw a graph of function and check the cutting points. Real roots means simply cutting points of the graphs. But you should have knowledge of drawing graphs. Drawing graphs and checking real roots are the best way of solving a number of real roots questions.
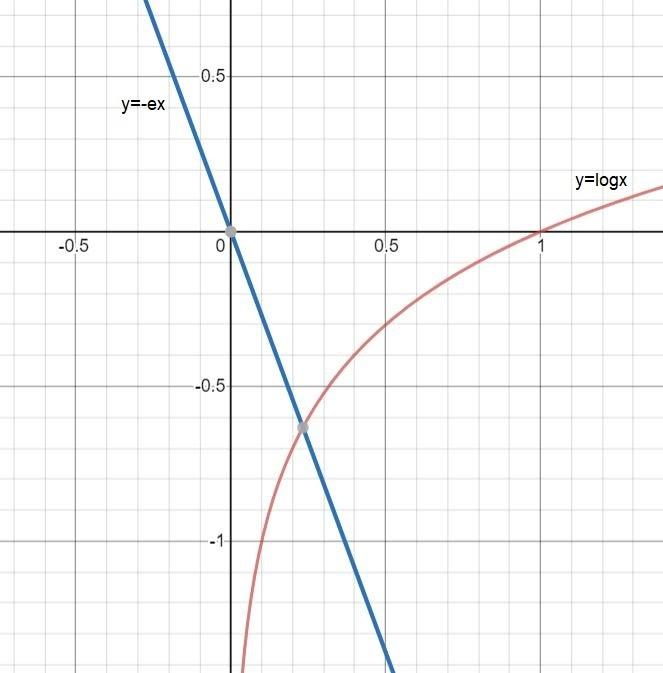
Complete step-by-step solution -
From given
${\log _e}x + ex = 0$
You can write it as
${\log _e}x = - ex$
Now you have to draw the graph of $y = {\log _e}x$ and graph of y = -ex .
You should have knowledge of graphs of logarithmic function and also knowledge of graphs of straight line. Now you have to check the cutting points of both the graphs.
So, you can see in diagram graph cuts each other at only one point so there is only one real root of the equation.
Hence option B is the correct option.
Note: -Whenever you get this type of question the key concept of solving is you have to draw a graph of function and check the cutting points. Real roots means simply cutting points of the graphs. But you should have knowledge of drawing graphs. Drawing graphs and checking real roots are the best way of solving a number of real roots questions.

Recently Updated Pages
Differential Equations Chapter For JEE Main Maths

Combined Translational and Rotational Motion – Definition, Examples & Formulas

States of Matter Chapter For JEE Main Chemistry

JEE Main Chemistry Exam Pattern 2026 (Revised) - Vedantu

JEE Main Chemistry Question Paper PDF Download with Answer Key

Centre of Mass of Semicircular Ring: Formula, Derivation & Examples

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Displacement-Time Graph and Velocity-Time Graph for JEE

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models

Electron Gain Enthalpy and Electron Affinity for JEE

What is Hybridisation in Chemistry?

Other Pages
NCERT Solutions For Class 11 Maths Chapter 4 Complex Numbers And Quadratic Equations - 2025-26

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

NCERT Solutions For Class 11 Maths Chapter 6 Permutations And Combinations - 2025-26

NCERT Solutions For Class 11 Maths Chapter 5 Linear Inequalities - 2025-26

NCERT Solutions For Class 11 Maths Chapter 8 Sequences And Series - 2025-26

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025




