
What is the relation between
(A)
(B)
(C)
(D)
Answer
148.5k+ views
Hint: Construct the square illustrating the four charges at each corner. Now, use the formula of potential difference for each charge and add them with each other and make them equal to zero.
Formula used The potential difference of the system for a point charge can be calculated by the formula –
where,
Complete Step by Step Solution
According to the question, it is given that, there are four – point charges
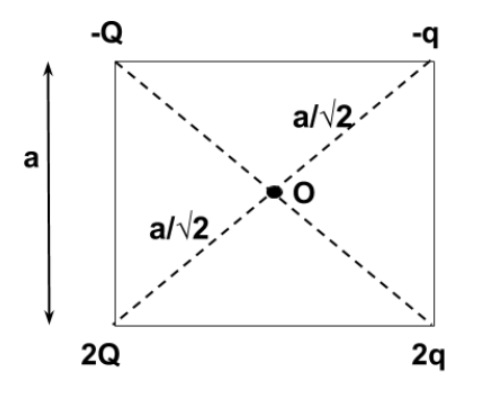
Let the side of the square be
Now, we know that, the potential difference of the system can be given by the formula –
As,
Hence, -
Now, the potential at the centre of square from each charge can be given by –
As it is given in question that potential at centre of square is equal to zero. So,
Now, we got the relation between the charges
Hence, the correct option is (C).
Note: Potential difference between two points is the work done in moving a unit positive charge between the two points. Its S.I unit is V.
The diagonal of the square can be calculated by multiplying the side of the square with
Formula used The potential difference of the system for a point charge can be calculated by the formula –
where,
Complete Step by Step Solution
According to the question, it is given that, there are four – point charges

Let the side of the square be
Now, we know that, the potential difference of the system can be given by the formula –
As,
Hence, -
Now, the potential at the centre of square from each charge can be given by –
As it is given in question that potential at centre of square is equal to zero. So,
Now, we got the relation between the charges
Hence, the correct option is (C).
Note: Potential difference between two points is the work done in moving a unit positive charge between the two points. Its S.I unit is V.
The diagonal of the square can be calculated by multiplying the side of the square with
Recently Updated Pages
Wheatstone Bridge - Working Principle, Formula, Derivation, Application

Young's Double Slit Experiment Step by Step Derivation

JEE Main 2021 July 25 Shift 1 Question Paper with Answer Key

JEE Main 2021 July 22 Shift 2 Question Paper with Answer Key

JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Uniform Acceleration

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Electrical Field of Charged Spherical Shell - JEE

Charging and Discharging of Capacitor

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics




