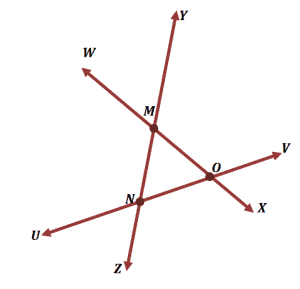
Definitions of Concurrent Lines And Intersecting Lines with Diagrams and Examples
FAQs on Difference Between Concurrent Lines And Intersecting Lines for Maths JEE Main 2026
1. What is an intersection point?
It refers to the precise location where two or more lines meet or cross each other. This point is common to all intersecting lines and can be denoted as a single coordinate on a coordinate plane.
2. What is a concurrency point?
It is the unique point where three or more lines intersect. Unlike intersecting lines, which can intersect at various points, concurrent lines converge at one specific location.
3. What are the types of angles formed by intersecting lines?
The intersection of lines leads to the formation of angles. Depending on the angle formed, intersecting lines can be classified as acute (less than 90 degrees), right (exactly 90 degrees), or obtuse (greater than 90 degrees) angles.
4. What is the centroid of a triangle?
The centroid is the concurrency point of the medians of a triangle. The centroid divides each median into segments with a ratio of 2:1.
5. Write some applications of intersecting lines.
Intersecting lines are foundational in geometry. They are crucial for understanding concepts such as angle relationships, the formation of polygons, and the identification of parallel and perpendicular lines.
6. What is the difference between concurrent lines and intersecting lines?
The difference between concurrent lines and intersecting lines is that concurrent lines are three or more lines meeting at a single point, while intersecting lines involve only two lines crossing at one point.
7. What are intersecting lines?
Intersecting lines are two lines that meet or cross each other at a specific point in the same plane.
8. Give Concurrent Lines Examples?
An example of concurrent lines is the altitudes of a triangle, which meet at a single point known as the orthocenter.
9. What are concurrent lines?
Concurrent lines are three or more lines that intersect or meet at exactly one point, called the point of concurrency.
10. How do concurrent lines and intersecting lines relate?
Concurrent lines and intersecting lines both involve lines meeting, but concurrent lines require three or more lines, while intersecting lines involve just two.