




What are The Fundamental Principles of Counting?
The Fundamental Principles of Counting is actually a field of Mathematics that deals with the number of possible permutations, combinations and the other methods of calculating the different arrangements and selections. The principles of counting essentially give us different basic counting techniques that can be applied to figure out the number of possible outcomes in the case of a given event. Extensively used in the field of Mathematics and Science, the basic principle of statistics can be of huge help, especially when there are large values involved.
There are four basic counting principles that are most commonly implemented and these include addition, subtraction, multiplication and division. There is another method that can be used in the same context, which is termed the principle of cardinality. In this article, we primarily focus on the four main operations excluding the principle of cardinality.
Different Types of Counting Principles
As mentioned before, there are four basic principles of counting that are most widely used. They are as follows:
Addition principle
Multiplication principle
Subtraction principle
Division principle
Of the four mentioned principles of counting, the fundamental multiplication of counting is probably the most useful one. This technique is also termed the ‘product rule’. To further understand each of these individual theorems and rules, we take a deeper dive into the topic. Here, we focus on the principles of addition and multiplication.
The Principle of Addition
The principle of addition, which is also termed the product rule, states that if we consider two tasks that have to be done or two separate events that cannot occur simultaneously, then the number of possible ways of completing the task will be given by the sum of the two different ways that could be used to complete.
This means that if a particular situation or a task can be resolved or completed in n1 or n2 number of ways, then the task can be performed in n1 + n2 when both the tasks cannot be performed simultaneously. Whenever there is a statement containing an ‘OR’, this method is applied.
The Principle of Multiplication
The principle of multiplication or the product rule deals with the ‘AND’ statement. According to this principle: ‘If a task can be performed in a sequence of tasks, where one task is being completed one after the other, then the total number of ways the job can be performed is a product of the total number of ways they can be performed individually.’
This means, that if a task can be performed in n₁ and n₂ ways respectively, then the total number of ways in which the task can be completed will be a product of the different ways. This means there are n₁ × n₂ ways of completing the task after the first task is done with.
Solved Examples Pertaining to the Fundamental Principle of Counting
Example 1: Four cards are chosen at random from a standard 52-card deck, with replacements. How many 4 card sequences can be determined when all four cards are of the same suite?
Solution:
Total number of suits in a deck = 4 ( diamonds, hearts, clubs and spades)
Total number of cards in each suite = 13.
Since we have to determine the number of sequences possible by taking 4 cards from the same suite, the 4 cards will either be all hearts, all spades, all diamonds or all clubs.
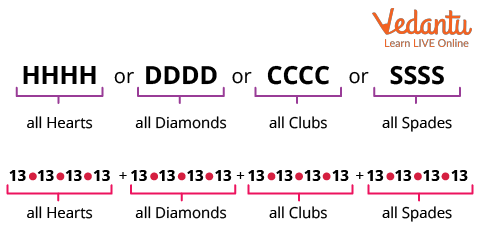
Ways of Selecting Four Cards from The Same suit
Therefore, the number of ways in which we can choose the hearts = 13 × 13 × 13 × 13.
Similarly, while selecting all diamonds, all clubs and all spades card sequences, we follow the same method.
Therefore, the total number of possible ways in which the four-card sequence can be selected from the same suit for a random pack of cards is = (13 × 13 × 13 × 13) × 4 = 114244.
Thus, if you have to choose a set of four cards where every card is from the same suit of an arbitrary deck of cards, there are 114244 different ways of selection.
Example 2: Suppose there are 10 different donuts, 20 different cookies and 5 types of pastries. How many possible choices can you make and how many different combinations of orders can you place?
Solution:
This particular example implements both, the sum rule for calculating the total number of choices and the product rule for calculating how many different orders can be placed.
Total number of different donuts = 10
Total number of different cookies = 20
Total number of different pastries = 5
Therefore, the total number of choices that we can make = 10 + 20 + 5 = 35.
Thus, there are 35 different choices at your disposal. Here, we can apply the sum rule. Therefore, the total number of possible orders that you can place = 10 × 20 × 5 = 1000.
Here, unlike the number of choices that are fixed, you can order either a single item, all the items, or even some of the items. Considering all these combinations and applying the multiplication principle, we get 1000 different ways of ordering.
Conclusion
The fundamental principle of counting deals with the different ways of selecting certain items or the number of ways in which a task can be performed. The addition principle adheres to states where the tasks are completed individually and not simultaneously. The product rule is applied in the case of a statement where one task is finished after the other and all mentioned methods can be applied for the completion of the task. The subtraction method or the PIE technique is implemented for either or all statements.
FAQs on Fundamental Counting Principle of Multiplication for JEE
1. Does the fundamental principle of counting allow repetitions?
The fundamental principle of counting includes all and every possible combination and permutation that an individual can apply. The combination is that field in mathematics that deals with the different ways or number of selections that one can make. Therefore, if there are 5 different articles to choose from, one can choose 1, 2, 3, 4 or all the articles. Similarly, if there are 5 same items, choosing more than one will give rise to repetition. Thus, the fundamental principle of counting does allow repetitions.
2. What do you mean by the PIE technique of counting?
The principle of subtraction is most commonly termed the PIE rule. PIE is the abbreviation for the term ‘Principle of Inclusion-Exclusion’. It states: ‘If a task can be done either in n₁ or in n₂ number of ways, then the total number of ways of completing the task in n1 + n1 - (the number of ways to do the task that are common to both).’ Here, we take the help of concepts like union and intersection, such that between 2 sets, A and B, A∪B = A + B + A ⋂ B.


















