Inequalities, Definition, Rules and Properties for JEE

The conventional meaning of inequality is that any two things are not equal. They might be discriminated against by their value or quality or importance. This can be easily related to mathematics. Also in mathematics, inequality states that two values are not equal. Those values can consist of expressions, functions, variables or any constant real numbers. This definition also delineates the fact that though two things are not equal, there is a relation between them which is likely regarded as the term, Inequality.  

This concept is used to compare any two values in the math system and tells the relation between those values, i.e., whether one value is less than or greater than or equal to the other value.  

Types of Inequalities 

There are symbols like less than <, greater than >, less than or equal to ≤, greater than or equal to ≥ and not equal ≠ which are used to compare any two values in arithmetic. 

If the two values taken are compared using only less than or greater than, then this type of inequality is termed Strict Inequality. 

E.g. a > 7

x+7 < y

But if those two values are compared using the symbols ≤ or ≥, then this type of inequality will come under Slack Inequality as these are not completely strict. 

E.g. 31 + x ≥ 40 

Z ≤ Y 

Note: If any inequality consists of only one variable, let’s say x, then it is supposed to be called an open sentence.  

 E.g.  x > 25, x ≤ 56 

Properties of Inequalities 

There are various properties of inequalities which are tested and proved. 

Let’s use some variable values here such as l, m and n where these are all real numbers. 

1. Addition Property: This states that when a constant number is added to the two sides of an inequality, the inequality doesn’t change. 

I.e., If l > m and n = constant, then l + n > m + n 

Similarly, 

If l < m and n = constant, then l + n < m + n  

2. Subtraction Property: As described in the addition property, likewise when a constant number is subtracted from both sides of the inequality, it will not change. Let’s demonstrate this as 

If l ≥ m, then l - n ≥ m - n 

And 

If l ≤ m, then l - n ≤ m - n 

3. Multiplication Property: Here there are two cases. 

• Case 1 - If a constant positive number is multiplied to both sides of the inequality, then it remains the same. 

• Case 2 - But if a negative number is multiplied to both sides of the inequality, then it must be reversed, i.e., changes the direction. 

E.g., If l > m, n > 0 then l.n > m.n → Sign remains same

But if l > m and n < 0 then l.n < m.n → Sign gets reversed 

4. Division Property: This is the same as the multiplication property. 

E.g.  If l ≤ m, n > 0 then  $\dfrac{l}{n} \leq \dfrac{m}{n}$  → Remains the same 

 Meanwhile, If l ≤ m and n < 0, then  $\dfrac{l}{n} \geq \dfrac{m}{n}$   → Reverses   

5. Transitive Property: This describes the relation between three values. 

If l ≥ m and m ≥ n, then l ≥ n 

If l ≤ m and m ≤ n, then l ≤ n 

Note: In this relation, if one inequality is strict, then the final one will also be strict. For example, l ≤ m and m < n then l < n. 

6. Converse Property: As the name says, if the two values at the left and the right side of the inequality are reversed, then the sign is also reversed. 

I.e.,    If l > m then m < l  

Important note: Only in Multiplication and Division, if both the sides are divided by or multiplied with a negative number, then the direction of the inequality sign will be reversed. In all the rest of the cases, the inequality sign remains the same. 

Solving Linear Inequalities

Linear inequalities look like linear equations just with a difference of an inequality sign in place of equal to. 

Linear equation → y = 5x - 2 

Linear Inequality → y > 5x - 2 

To solve such kinds of inequalities, we can choose the process of how we solve general linear equations. 

Let’s see a few kinds of example problems on inequalities. 

Problem 1: Solve 2x + 5 < 4x - 15, 

Ans: Solving this one using the rules of inequalities discussed above, 

Adding 15 on both sides, 

2x + 20 < 4x     

Subtracting 2x from both sides, we get 

20 < 2x 

Dividing both sides by 2, 

10 < x   →   x > 10 

Problem 2: Solve, 18 - x > 12 

Ans: Subtracting 12 from both sides, we get 

6 - x > 0 

Multiplication with - 1 on both sides reverses the sign 

x - 6 < 0 

Therefore, by adding 6 on both sides, we obtain 

x < 6 

This means any value of x less than 6 satisfies the given inequality condition.

Now let’s try solving inequalities with fractions.         

Problem 3: Solve $\dfrac{y-3}{2} \geq \dfrac{5}{4}$

Ans: Multiplying with 4 on each side, 

2(y - 3) ≥ 5 

 I.e.,  2y - 6 ≥ 5 

Adding 6 on both sides, 

 2y ≥ 11  

Dividing both sides by 2, we get the value of y as 

 y ≥ 5.5  

Graphing of an Inequality: Two Variables 

Let us take an example, 2y ≥ 4x - 8 which consists of two variables x and y respectively. 

Dividing both sides by 2, we obtain the equation y ≥ 2x - 4

We can plot this inequality on the XY graph by plotting the linear equation, y = 2x - 4 first. Let’s find two sets of points where the line cuts the axes. 

If x = 0, then y = -4, the point is (0, - 4). 

If y = 0, then x = 2, the point is (2, 0). 

Let’s draw the line passing through the above two points. Then choose origin and substitute in the inequality. If inequality holds true then the region which contains origin will be the solution region. If inequality does not hold true then the region which doesn’t contain origin will be the solution. The area above will be the solution area needed for this problem y ≥ 2x - 4. The solution lies in the highlighted area of the graph shown below. 

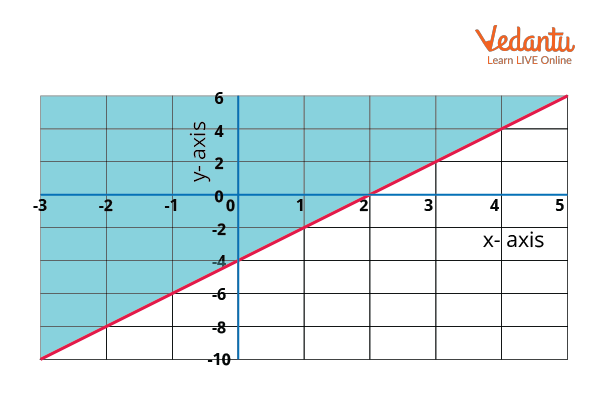
Graphing Inequalities with two variables,  y ≥ 2x - 4 

Conclusion 

Herein, we can conclude that any inequality could be solved with the above-mentioned rules of inequalities and properties. Inequality signs are used widely in mathematics and solving problems on inequalities and solving inequalities with fractions are also important.