Question

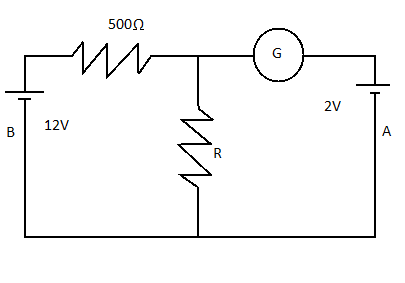
In the circuit, the galvanometer G shows zero deflection. If the batteries A and B have negligible internal resistance, the value of resistor R will be:

(A) \[100\Omega \]
(B) \[200\Omega \]
(C ) \[1000\Omega \]
(D) \[500\Omega \]

Answer 1

Hint: The circuit can be broken and considered as two separate loops. The current through both the loops are equal and thus the value of resistor R, can be calculated. The value of current can be calculated using Kirchhoff’s Law and then using that value of current in Ohm’s law, we can find out the value of R.

Complete step by step solution:
In the question it is mentioned that the galvanometer shows zero deflection. This means that the current through both the loops are equal.
Let us divide the circuit in two loops, ABEF and BCDE, as shown in the figure.

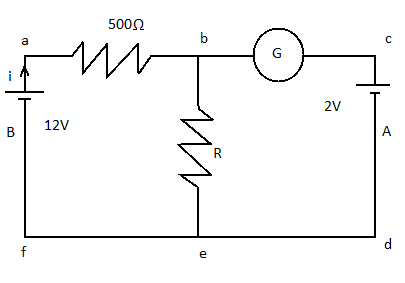
Let us consider current \[i\] flowing through the circuit.
Kirchhoff’s laws are basically two laws, one is referred to as Kirchhoff’s current law and another is Kirchhoff’s voltage law. Considering loop abcdefa , we apply Kirchhoff’s Voltage law:
This law states that in any loop, total voltage around any loop is equal to the sum of voltage drops within the same loop.
This law is applied as:
\[500i + 2 - 12 = 0\]
On solving the equation:
\[500i = 10\]
Thus, we obtain:
\[i = \dfrac{{10}}{{500}}A\]……………….(i)
Now, let us consider the loop abefa:
Again applying Kirchhoff’s Voltage law:
\[500i + Ri = 12\]
On solving the equation, we obtain:
\[i = \dfrac{{12}}{{R + 500}}\]……………………(ii)
Now, since there is no current flowing through the galvanometer, as it shows zero deflection, equation (i) and (ii) must be equal.
\[\dfrac{{10}}{{500}} = \dfrac{{12}}{{R + 500}}\]
On, solving the equation, we obtain:
\[R = 100\Omega \]
This is the required solution.
Thus option (A) is correct.

Note: Kirchhoff’s current law states that the total amount of current entering a loop must be equal to the total amount of current leaving the loop. While writing the KVL equation, if a positive terminal of voltage difference is encountered before a negative terminal, voltage difference is taken as positive.