
If specific heat capacity of a substance in solid and liquid state is proportional to temperature of the substance then it heat is supplied to the solid initially at
A)

B)
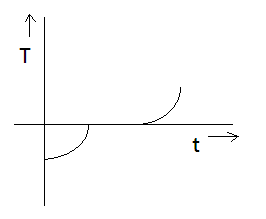
C)
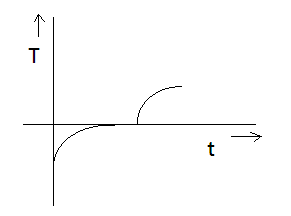
D)

Answer
154.2k+ views
Hint: We have to obtain a relation in Temperature (T) and time (t) from the given information. The solid is initially at
Complete step by step solution:
As we have discussed in the hint, we have to obtain a relation in temperature and time. Let’s first note down the given information. It makes it easier to solve the question.
The given information is:
i) Specific heat capacity is proportional to temperature:
Let there be a constant of proportionality
ii) The heat is supplied at a constant rate:
Let this constant be
Now, we have equations of rate of heat supplied and specific heat capacity. We have variables of temperature and time in them. Now we need to think of an equation which combines them.
The equation which relates them is as follows:
Where
If we divide equation
Thus, dividing equation
Also, from equation
But we have rate of heat transfer is constant, from equation
Here we have an equation in temperature and time. But the temperature and time are in differential form, we will have to integrate them. In order to integrate, we will have to take constant terms on one side.
As you can see the left-hand side, we have only constants. After rearranging the equation, we have:
Let the constant,
This simplifies our equation as follows:
Now, we have a relation in time and temperature.
We will have to integrate this equation from time
Here, we can put values of temperature and observe but that is not needed.
The given graph is of temperature versus time and our relation in temperature and time is of the following nature.
From the above discussion, it is clear that the graph cannot be linear. Therefore, option A is also eliminated.
The graph of
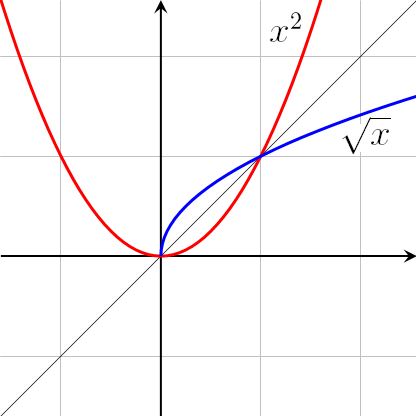
The blue curve denotes the required graph which is similar to option C.
Thus, Option (C) is the correct option.
Note: Remember all the steps in this question. The graph in option C is not uniform as at
Complete step by step solution:
As we have discussed in the hint, we have to obtain a relation in temperature and time. Let’s first note down the given information. It makes it easier to solve the question.
The given information is:
i) Specific heat capacity is proportional to temperature:
Let there be a constant of proportionality
ii) The heat is supplied at a constant rate:
Let this constant be
Now, we have equations of rate of heat supplied and specific heat capacity. We have variables of temperature and time in them. Now we need to think of an equation which combines them.
The equation which relates them is as follows:
Where
If we divide equation
Thus, dividing equation
Also, from equation
But we have rate of heat transfer is constant, from equation
Here we have an equation in temperature and time. But the temperature and time are in differential form, we will have to integrate them. In order to integrate, we will have to take constant terms on one side.
As you can see the left-hand side, we have only constants. After rearranging the equation, we have:
Let the constant,
This simplifies our equation as follows:
Now, we have a relation in time and temperature.
We will have to integrate this equation from time
Here, we can put values of temperature and observe but that is not needed.
The given graph is of temperature versus time and our relation in temperature and time is of the following nature.
From the above discussion, it is clear that the graph cannot be linear. Therefore, option A is also eliminated.
The graph of

The blue curve denotes the required graph which is similar to option C.
Thus, Option (C) is the correct option.
Note: Remember all the steps in this question. The graph in option C is not uniform as at
Recently Updated Pages
JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

Chemical Properties of Hydrogen - Important Concepts for JEE Exam Preparation

JEE Energetics Important Concepts and Tips for Exam Preparation

JEE Isolation, Preparation and Properties of Non-metals Important Concepts and Tips for Exam Preparation

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Degree of Dissociation and Its Formula With Solved Example for JEE

Displacement-Time Graph and Velocity-Time Graph for JEE

Electrical Field of Charged Spherical Shell - JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line




