
(A) A lobe along the z-axis and a ring along xy-plane
(B) A lobe along the z-axis and a lobe along xy-plane
(C) A lobe along the z-axis and a ring along yz-plane
(D) A lobe and ring along the z-axis
Answer
157.5k+ views
Hint: Every orbital has a different shape and different lobes. The shape is decided by the azimuthal quantum number which is denoted as ‘l’.
Complete step by step solution:
-There are 4 quantum numbers n, l, m and s. The principal quantum number (n) tells us about the shells of the atom. The azimuthal quantum number (l) tells us about the subshells of the atom. The magnetic quantum number (m) tells us about the orbitals of the atom and the spin quantum number (s) tells us about the orientation of the electrons in the orbital.
-Third quantum number is the magnetic quantum number and is denoted by m. It gives us the exact orbitals. Its total value can be given as
So, the d-subshell comes into picture when the value of n=3 and there are 5 orbitals for d-subshell since the value of l will be 2 for it. These orbitals are
-d-orbitals can be formed in 2 ways: between the axis and along the axis.
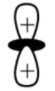
This is the shape of
So the correct option is A. A lobe along the z-axis and a ring along xy-plane.
Note:
Complete step by step solution:
-There are 4 quantum numbers n, l, m and s. The principal quantum number (n) tells us about the shells of the atom. The azimuthal quantum number (l) tells us about the subshells of the atom. The magnetic quantum number (m) tells us about the orbitals of the atom and the spin quantum number (s) tells us about the orientation of the electrons in the orbital.
-Third quantum number is the magnetic quantum number and is denoted by m. It gives us the exact orbitals. Its total value can be given as
So, the d-subshell comes into picture when the value of n=3 and there are 5 orbitals for d-subshell since the value of l will be 2 for it. These orbitals are
-d-orbitals can be formed in 2 ways: between the axis and along the axis.

This is the shape of
So the correct option is A. A lobe along the z-axis and a ring along xy-plane.
Note:
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

Chemical Properties of Hydrogen - Important Concepts for JEE Exam Preparation

JEE Energetics Important Concepts and Tips for Exam Preparation

JEE Isolation, Preparation and Properties of Non-metals Important Concepts and Tips for Exam Preparation

Trending doubts
Degree of Dissociation and Its Formula With Solved Example for JEE

Geostationary Satellites and Geosynchronous Satellites - JEE Important Topic

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

What is Hybridisation in Chemistry?

Nitrogen gas is prepared A By heating ammonium nitrate class 11 chemistry JEE_Main

IIIT JEE Main Cutoff 2024




