Question

A drop of water is placed on a glass plate. A double convex lens having a radius of curvature of each surface 20 cm is placed on it. The focal length of water lens (\[\mu \] for water = \[\dfrac{4}{3}\]​), will be ________.



(A) \[ - 0.20\]m
(B) \[ - 0.60\]m
(C) \[0.30\]m
(D) \[0.40\]m

Answer 1

Hint: This given problem can be solved by taking the consideration of combination of lenses in ray optics. Lenses are made of either two spherical surfaces or one spherical surface and the other plane surface.

Complete step by step answer:
Step 1:

First we have to see the spherical surfaces from which these lenses are formed.
A refracting surface which forms a part of a sphere of transparent material is called a spherical refracting surface. There are two types of these surfaces:
a) Convex spherical refracting surface: In this type of surface convex is towards the rarer medium side.
b) Concave spherical refracting surface: In this surface is concave towards the rarer medium side.
Step 2:
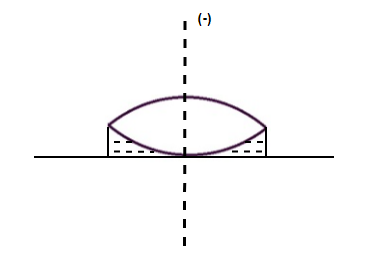
As we can see from the question that there is a drop of liquid on which the convex lens (double convex lens) is placed and liquid drop is on a glass plate so both of these combinations are making a Plano-concave lens. So, here we have to find the focal length of a Plano-concave lens.
Now, we know the formula to calculate the focal length of the lens –
\[\dfrac{1}{f} = \left( {\mu - 1} \right)\left( {\dfrac{1}{{\mathop R\nolimits_1 }} - \dfrac{1}{{\mathop R\nolimits_2 }}} \right)\] (1)
The given formula in the above equation (1) is also known as lens maker’s formula.
For the given water lens –
$\implies$ \[\mathop R\nolimits_1 = - \infty \] and \[\mathop R\nolimits_2 = 20\]cm and for water \[\mu = \dfrac{4}{3}\]
So, keeping all these values in equation (1), we will get –
$\implies$ \[\dfrac{1}{f} = \left( {\dfrac{4}{3} - 1} \right)\left( {\dfrac{1}{{ - \infty }} - \dfrac{1}{{20}}} \right)\], on solving this equation we will get –
$\implies$ \[\dfrac{1}{f} = \left( {\dfrac{1}{3}} \right)\left( { - \dfrac{1}{{20}}} \right)\] on further solving this equation
$\implies$ \[\dfrac{1}{f} = \left( { - \dfrac{1}{{60}}} \right)\], now on rearranging this equation
$\implies$ \[f = - 60\]cm i.e., \[f = - 0.60\]m.
So, the correct option is (B)

Note: It should be kept in mind while solving these types of problem that
1) For a concave lens, \[\mathop R\nolimits_1 \] is negative and \[\mathop R\nolimits_2 \] is positive. Therefore, its focal length is negative.
2)For a convex lens, \[\mathop R\nolimits_1 \] is positive and \[\mathop R\nolimits_2 \] is negative. Therefore, its focal length is positive.
Where, \[\mathop R\nolimits_1 \] and \[\mathop R\nolimits_2 \] being the radii of curvature of the two surfaces of the lens.