Question

A charged spherical conductor of radius a and charge q, is surrounded by another charged concentric sphere of radius b$(b > a)$. The potential difference between conductors is V. When, the spherical conductor of radius b is discharged completely, then the potential difference between conductor will be:
(A) V
(B) $\dfrac{{{V_a}}}{b}$
(C) $\dfrac{{{q_1}}}{{4\pi {\varepsilon _0}a}} - \dfrac{{{q_2}}}{{4\pi {\varepsilon _0}b}}$
(D) None of the these

Answer 1

Hint: we know that a spherical conductor cannot generate an electric field inside its surface by its own if an electric field exists there must be some potential we can use these concepts here.

Complete step by step answer




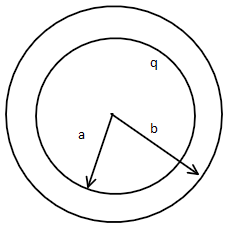
From the diagram, we can see that two concentric spheres, bigger one with radius b and smaller one with radius a and charge q are connected. It is given that the potential difference between them is V.
Let us assume on outer sphere the charge is ${q_1}$ at time t
Then after discharge ${q_1} = 0$
If there is potential difference, then there must be electric field in the sphere
This electric field cannot be due to outer sphere, it can be due to the inner sphere of radius ‘a’
Since the charge of the inner sphere remains ‘q’ throughout, hence the electric field between the two concentric spheres also remains the same.
Since the electric field is the same, this means that the potential difference will also remain the same that is V.

Hence, the correct option is A.

Additional information
To determine electric field on a body because of symmetric charge distribution Gauss theorem can be used. This law relates the electric field at a point on a closed surface to the enclosed charge by the surface. Electric potential is the work done on bringing a point charge from infinity that is your reference point to that point.

Note:
Electric field outside and inside the sphere is radially distributed. You should also note that the electric potential decreases in the electric field direction and is negative due to negative charge and positive due to positive charge except at infinity. The potential at infinity is zero.