Concepts of Thermal Physics for JEE Advanced Physics
The chapter Thermal Physics covers the most important concepts for JEE Advanced and other competitive exams. In the Thermal Physics chapter, students will study the basic heat transfer process and some laws related to the chapter.
The chapter on thermal physics begins with the very basic definition of Newton’s laws of cooling, the First law of thermodynamics and what are different other laws that are helpful in studying the chapter ahead. These concepts will lead us to a very important part of the chapter i.e. Reversible and Irreversible processes, Carnot engine, and efficiency. In this chapter, we will get to study many interesting and most important concepts such as thermal expansion, Specific heat capacity and Mean free path.
The concepts mentioned above will help us in studying the chapter deeply along with the hands-on practice of many important numerical problems that will help us in acing the exams.
In this article, we will cover the important concepts and topics that will help students to revise and boost their preparations for JEE.
JEE Advanced Physics Chapters 2025
Important Topics of Thermal Physics Chapter
Heat Transfer
First Law of Thermodynamics and Heat Capacity
Second Law of Thermodynamics
Newton’s Law of Cooling
Reversible and Irreversible Processes
Carnot Engine
Temperature and Thermal Expansion
Specific Heat Capacity and Mean Free Path
Thermodynamic Variables
Thermal Physics Important Concept for JEE Advanced
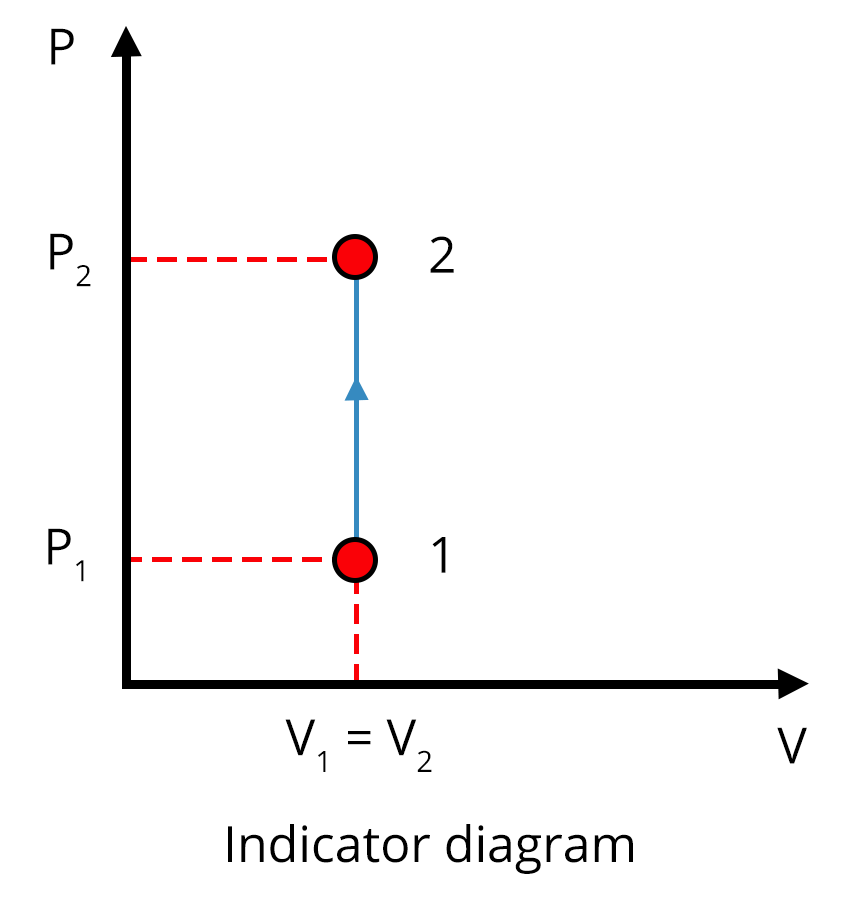
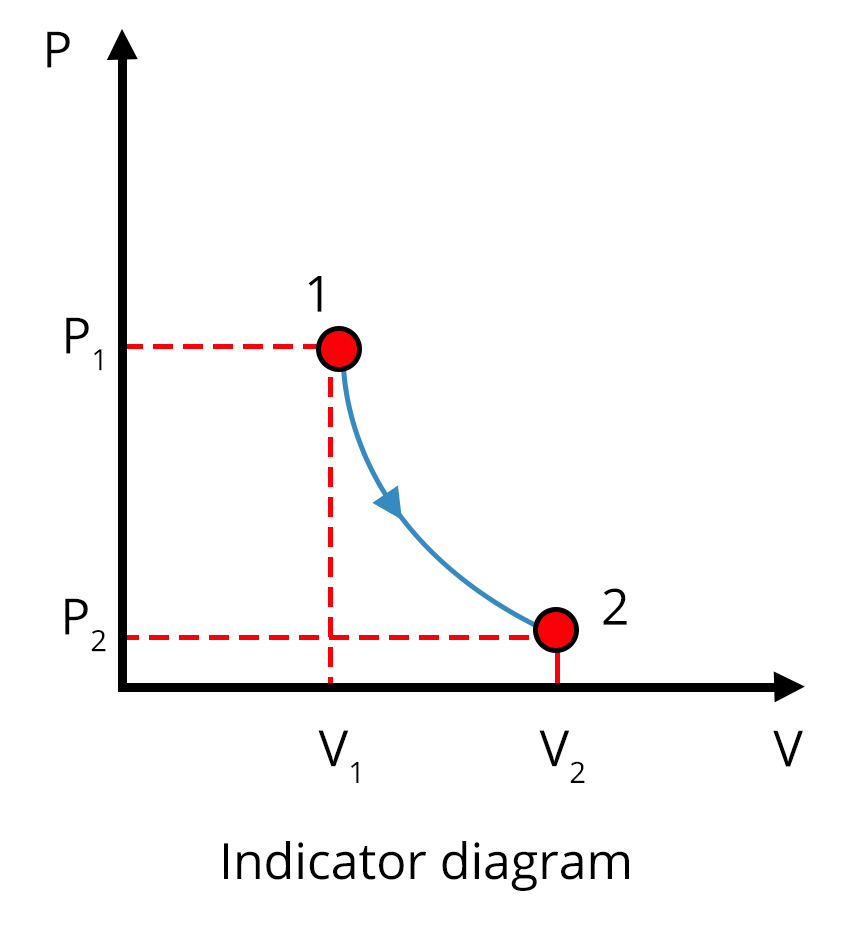
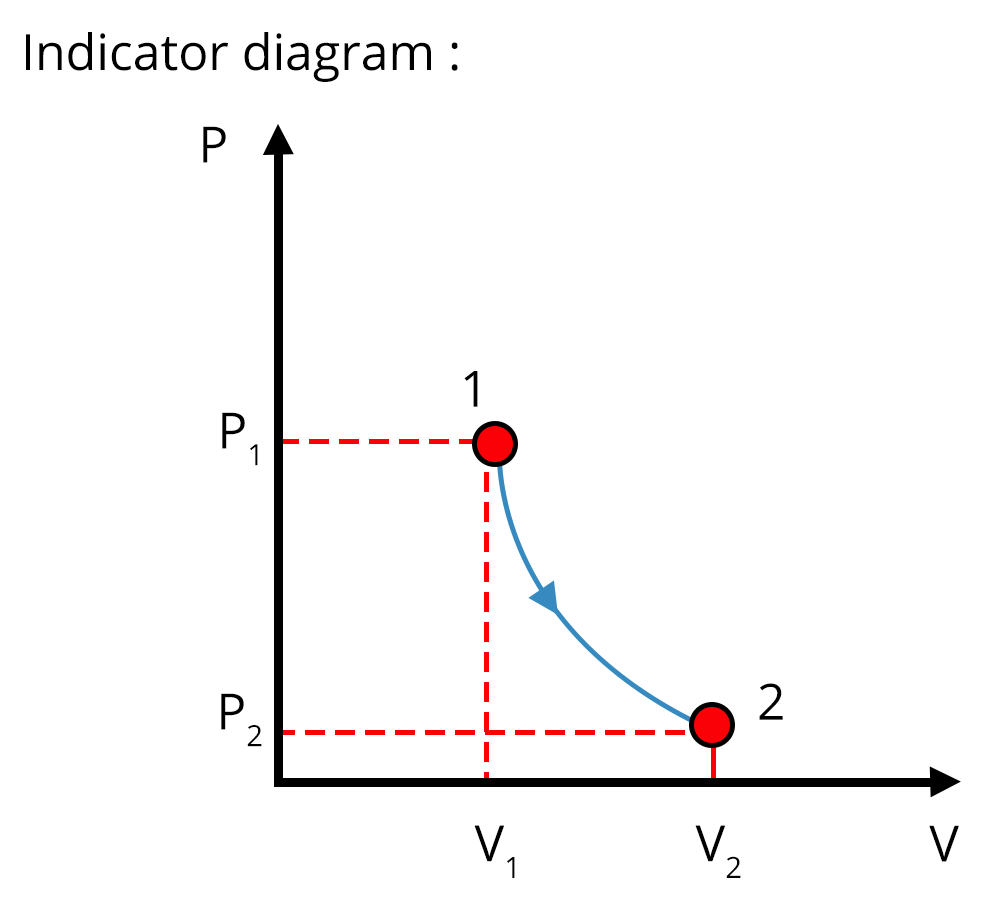
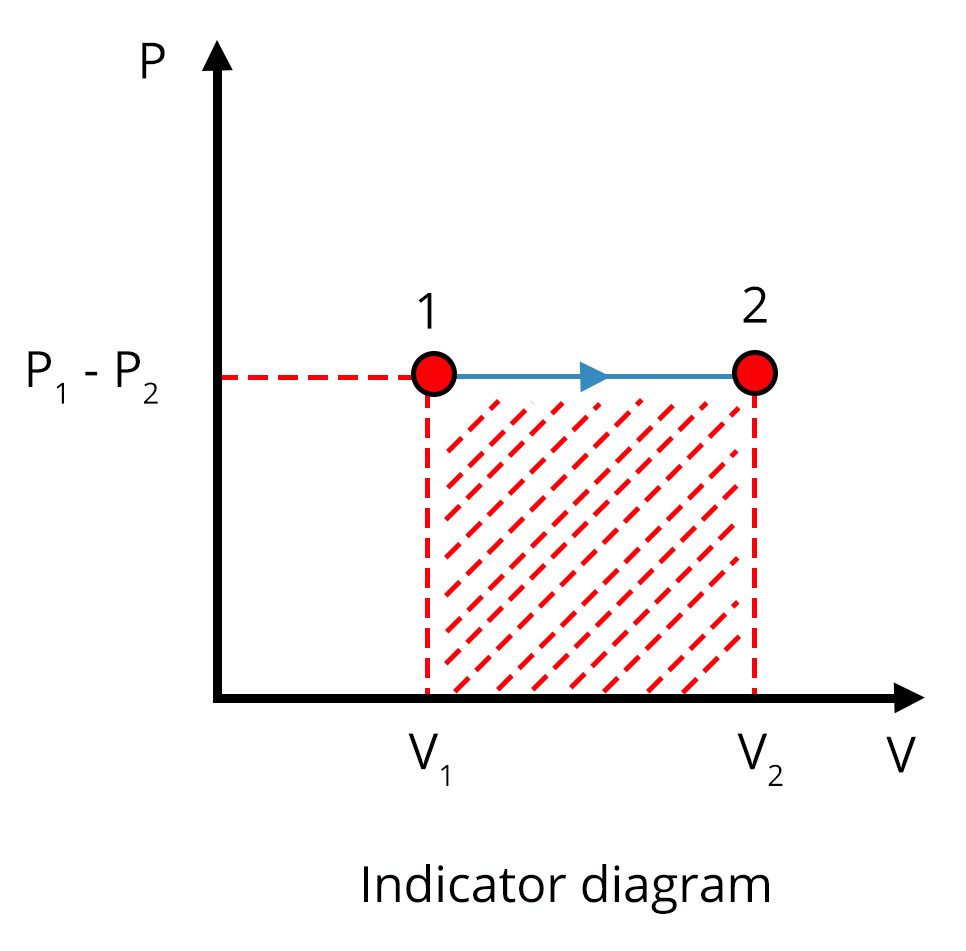
Different Thermodynamic Processes
List of Important Formulae of Thermal Physics
JEE Advanced Thermal Physics Solved Examples
1. A steam engine delivers 5.4 x 108 J of work per minute and services 3.6 x 109 J of heat per minute from its boiler. What is the efficiency of the engine? How much heat is wasted per minute?
Sol:
Given,
Work done by the boiler per minute (output work) = 5.4 x 108 J
Input work heat absorbed by boiler = 3.6 x 109 J
Hence efficiency = $\eta = \dfrac{\text{output}}{\text{input}}$
= $\dfrac{5.4\times 108} {3.6\times 109}$
=0.15
So the heat wasted per minute is equal to the difference between heat absorbed per minute and output work per minute.
= $\left(3.6 \times {10^9}\right)- \left (5.4 \times {10^8}\right) J$
=$3.06 \times {10^9} J$
Therefore, the efficiency of the engine is 0.15 and heat is wasted per minute.
Key Point: The relationship between the output work and input work is given by relation $\eta = \dfrac{\text{output}}{\text{input}}$
2. A cylinder with a movable piston contains 3 moles of hydrogen at standard temperature and pressure. The walls of the cylinder are made of a heat insulator, and the piston is insulated by having a pile of sand on it. By what factor does the pressure of the gas increase if the gas is compressed to half its original volume?
Sol:
Given,
The cylinder is undergoing adiabatic compression hence for a diatomic gas(hydrogen) the value of $\gamma$ = 1.4 and as mentioned in the ques final volume = half of the initial one
$V_{2} = \frac{V_{1}}{2}$
$P_{2}$ = 1atm
Therefore for adiabatic process
$P_{1}V_1^\gamma$ = $P_{2}V_2^\gamma$
$V_1^\gamma$ = $P_{2}V_2^\gamma$
$V_1^\gamma$ = $P_{2}(\frac{V_{1}}{2})^{\gamma}$
Hence $P_{2}= 2^{1.4}$
=2.64atm
Therefore, 2.64 atm pressure of the gas increase if the gas is compressed to half its original volume.
Key point: In the case of adiabatic processes, the system is always an insulated one so that no heat can be absorbed or released by the system.
Previous Year Questions of Thermal Physics
1. Under isothermal conditions, a gas at 300 K expands from 0.1 L to 0.25 L against a constant external pressure of 2 bar . The work done by the gas is( Given that 1L bar=100J)(NEET 2019)
5 kJ
25 J
30 J
-30 J
Sol:
Given,
Pressure of the gas = 2bar
Change in volume = $\triangle V$ = 0.25-0.1 = 0.15 L
Since the process is isothermal conditions hence temperature of gas = 0K
From 1st law of thermodynamics
$\triangle Q $ = $\triangle U$ + $\triangle W$
$\triangle Q $ = 0 which means
$\triangle U$ = - $\triangle W$
So the work done is calculated as $\triangle W$ = - $P\triangle V$
=-2$\times$ 0.15 = -0.3L-bar
And as given in ques 1L-bar = 100 J
Hence net work done in Joule is -30J Which is option (d)
Trick: As the given process is isothermal and by the formula of thermodynamics one can see that work done will be negative. So without calculations just by looking at the options, a student can go for an option with a negative sign.
2. A Carnot freezer takes heat from water at $0^{\circ} C$ inside it and rejects it to the room at a temperature of $27^{\circ}C$. The latent heat of ice is $336\times10^{3}$ J/kg. If 5 kg of water at $0^{\circ}C$ is converted into ice at $0^{\circ}C$ by the freezer, then the energy consumed by the freezer is close to: (JEE 2016)
$1.67\times10^{5}$ J
$1.68 \times10^{6}$ J
$1.51\times10^{5}$ J
$1.71 \times10^{7}$ J
Sol:
Given,
Temperature of sink = $T_{sink}$ = $0^{\circ} C$ = 273K
Temperature of source = $T_{source}$ = $27^{\circ} C$ = 300K
Now the latent energy required by freezer to convert ice to water will be the heat rejected by the freezer i.e. $Q_{sink}$ = mL
= 5 $\times 336 \times 10^{3}$ = $1680 \times 10^{3}$ J
From the formula of carnot engine
$\dfrac{T_{sink}}{T_{source}}$ = $\dfrac{Q_{sink}}{Q_{source}}$
Hence $\dfrac{273}{300}$ =$\dfrac{1680 \times 10^{3}}{Q_{source}}$
Or ${Q_{source}}$= $1846.1 \times 10^{3}$ J
So the energy consumed by the freezer = ${Q_{source}}$ - ${Q_{sink}}$
=$1846.1 \times 10^{3}$- $1680 \times 10^{3}$J
= $1.67\times10^{5}$ J which is option (a)
Trick: Here both the conversion of ice into water which is used as latent heat, formula of Carnot engine play an important role in solving the ques.
Practice Questions
A quantity of air at normal temperature is compressed (a) slowly (b) suddenly to one-third of its volume. Find the rise in temperature, if any in each case $\gamma$ = 1.4 (Ans-(a) zero (b) 150.6$^{\circ}C$)
The water of one kg mass at 373 K is converted into steam at the same temperature and at atmospheric pressure. On boiling, 1 cc of water takes a volume of 1671 cc. Calculate the change in internal energy of the system taking the heat of vaporization to be 540 cal/g.(Ans- 499.84k cal)
JEE Advanced Physics Thermal Physics Study Materials
Here, you'll find a comprehensive collection of study resources for Thermal Physics designed to help you excel in your JEE Advanced preparation. These materials cover various topics, providing you with a range of valuable content to support your studies. Simply click on the links below to access the study materials of Thermal Physics and enhance your preparation for this challenging exam.
JEE Advanced Physics Study and Practice Materials
Explore an array of resources in the JEE Advanced Physics Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Advanced exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Physics preparation.
Conclusion
We conclude that the chapter ‘Thermal Physics’ has many important concepts that will help us in scoring better marks in NEET and JEE. In this article, we covered thermal expansion, Heat engines, Efficiency and all other important concepts from the chapter along with solved and previous year questions. Students can test their knowledge with the help of practice questions.
FAQs on Thermal Physics - Physics JEE Advanced
1. Is Thermal Physics important for the JEE/NEET exam?
Yes, the thermal physics chapter is one of the important chapters for JEE and NEET exams. It carries around 2-3 questions. Due to the formula related questions from this chapter, it becomes easy to solve and secure marks in the exams.
2. Is the thermal physics chapter tough?
No, the thermal physics chapter is simple and easy to understand. The chapter includes the basic concepts that we got introduced to in our elementary school. If you understand the concepts discussed in this chapter and practice related questions then you can successfully attempt the questions asked in this chapter in both NEET and JEE exams.
3. Can I crack NEET by solving the previous years’ questions?
According to experts, applicants who practice previous year's papers for JEE and NEET have a good probability of passing the exam. Anyway, practice is the key to success. Students must focus on solving methods and study conceptually along with the PYQs.