




Essential Formulas and Problem-Solving Strategies for Success
The study of geometric figures using coordinate axes is known as coordinate geometry, which is an important chapter for JEE Main Exam. Straight lines, curves, circles, ellipses, hyperbolas, and polygons can all be conveniently drawn and represented to scale in the coordinate axes. Furthermore, using the coordinate system to work algebraically and examine the characteristics of geometric figures is referred to as coordinate geometry.
In this article, We will go through some of the important concepts of co-ordinate geometry like what is coordinate geometry - definitions, explanations and formulas. Also, we will go through some of the solved coordinate geometry problems for a better understanding of the concept.
Important Topics of Maths Coordinate Geometry Chapter
Cartesian Coordinates
Distance Formula
Distance Between Two Points
Slope
Midpoint formula
Equation of a line
Slope-Intercept Form of a Line
Point Slope Form
Euclidean Distance Formula
Maths Coordinate Geometry Important Concept for JEE Main
What is Coordinate Geometry?
Coordinate geometry is a field of mathematics that aids in the presentation of geometric shapes on a two-dimensional plane and to study their properties. To get a basic understanding of Coordinate Geometry, we will learn about the coordinate plane and the coordinates of a point initially.
Coordinate Plane
A cartesian plane divides plane space into two dimensions, making it easier to locate points. The coordinate plane is another name for it. The horizontal X-axis and the vertical Y-axis are the two axes of the coordinate plane. The origin is the place where these coordinate axes connect, dividing the plane into four quadrants (0, 0). Furthermore, any point in the coordinate plane is represented by a point (x, y), where the x value represents the point's position relative to the X-axis and the y value represents the point's position relative to the Y-axis.
The point represented in the four quadrants of the coordinate plane has the following properties:
The origin ‘O’ is the point of intersection of the X-axis and the Y-axis and has the coordinate points (0, 0) generally.
The X-axis to the right of the origin ‘O’ is the positive X-axis and to the left of the origin, ‘O’ is the -(ve) X-axis. Also, the Y-axis above the origin ‘O’ is the +(ve) Y-axis, and below the origin ‘O’ is the -(ve) Y-axis.
The point present in the first quadrant (x, y) has both +(ve) values and is plotted with reference to the +(ve) X-axis and the +(ve) Y-axis.
The point represented in the second quadrant is (-x, y) is plotted with reference to the -(ve) X-axis and +(ve) Y-axis.
The point represented in the third quadrant (-x, -y) is plotted with reference to the -(ve) X-axis and -(ve) Y-axis.
The point represented in the fourth quadrant (x, -y) is plotted with reference to the +(ve) X-axis and -(ve) Y-axis.

Coordinate Geometry Formulas
Coordinate geometry formulae make it easier to prove the various properties of lines and figures represented by coordinate axes. The distance formula, slope formula, midpoint formula, section formula, and line equation are included in the list of coordinate geometry formulas. In the below paragraph, we'll learn more about each of the formulas.
Coordinate Geometry Distance Formula
The distance between two points $(𝑥_1,𝑦_1$) and $(𝑥_2,𝑦_2)$ is equal to the square root of the sum of the squares of the difference between the X-coordinates and the Y-coordinates of the two given points. The formula for the distance between two points is as given below:
$d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}$
Applications of Coordinate Geometry
Coordinate geometry is a fascinating field with applications in various areas. Here are a few:
Used in designing structures, roads, and other infrastructures. Engineers and architects use coordinate geometry to create accurate plans and layouts.
In the world of computer graphics, coordinates are essential for creating images and animations. Gaming, virtual reality, and animation industries heavily rely on coordinate geometry.
GPS technology uses coordinates to pinpoint locations on the Earth's surface. This has applications in navigation for vehicles, airplanes, and even pedestrian directions on smartphones.
Physicists use coordinate geometry to describe the motion of objects and study physical phenomena. It's especially crucial in analyzing systems with multiple moving parts.
In economics, coordinate geometry can be used to analyze and visualize data. Businesses also use it for market research, spatial analysis, and planning.
In medical imaging, coordinate geometry is employed to locate and visualize structures within the body. It's vital in fields like radiology and surgery.
Maps use coordinates to represent locations on the Earth's surface. Cartographers use coordinate geometry to accurately depict geographical features.
In statistics, coordinate geometry helps in analyzing and visualizing data. Scatter plots, for example, are created using coordinates to understand the relationship between variables.
Astronomers use coordinates to locate celestial objects in the night sky. It's essential for observing and studying the movements of planets, stars, and galaxies.
Coordinate geometry is fundamental in programming the movements of robots. Robots are often designed to follow specific paths or reach certain coordinates in their operation.
Slope Formula
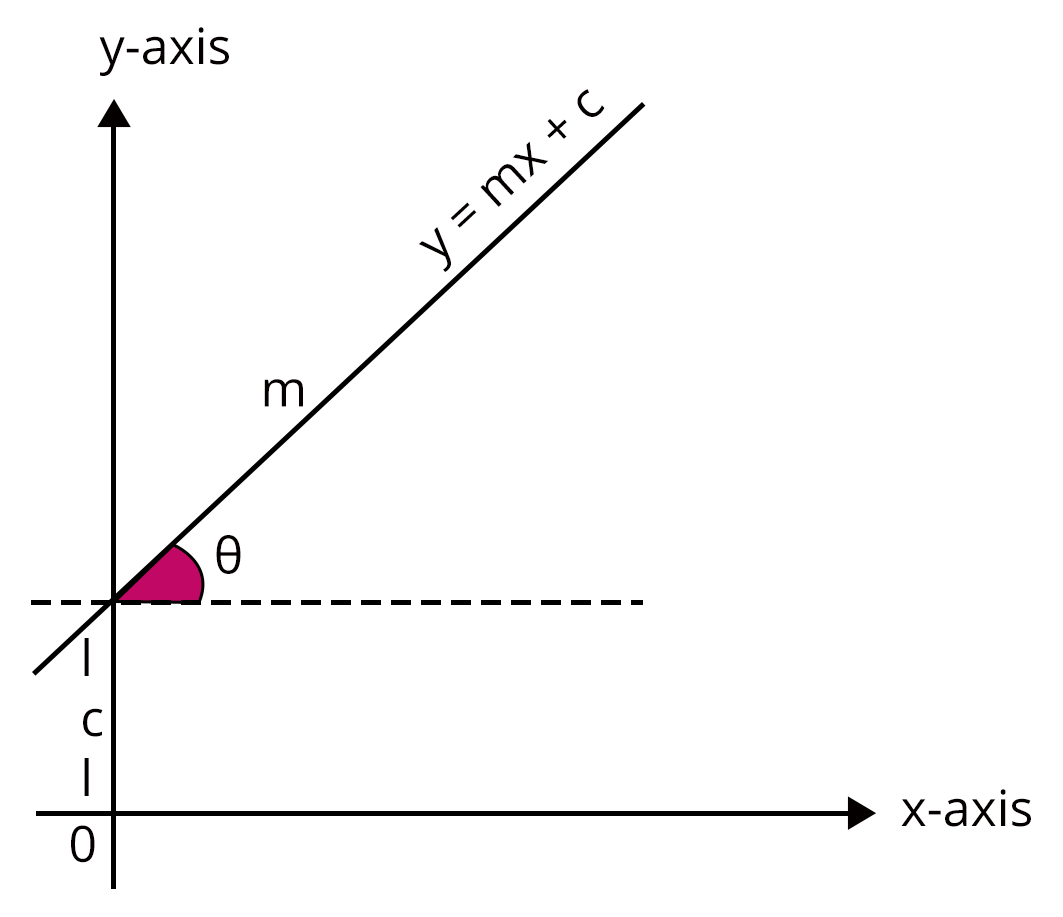
The inclination of a line is measured by its slope. The slope can be determined by selecting any two locations on the line and measuring the angle formed by the line with the positive X-axis. m = tanθ is the slope of a line that is inclined at an angle 'θ' with the positive X-axis. The slope of a line joining the two points $(𝑥_1,𝑦_1$) and $(𝑥_2,𝑦_2)$ is equal to
$m=\dfrac{y_2 - y_1}{x_2 - x_1}$
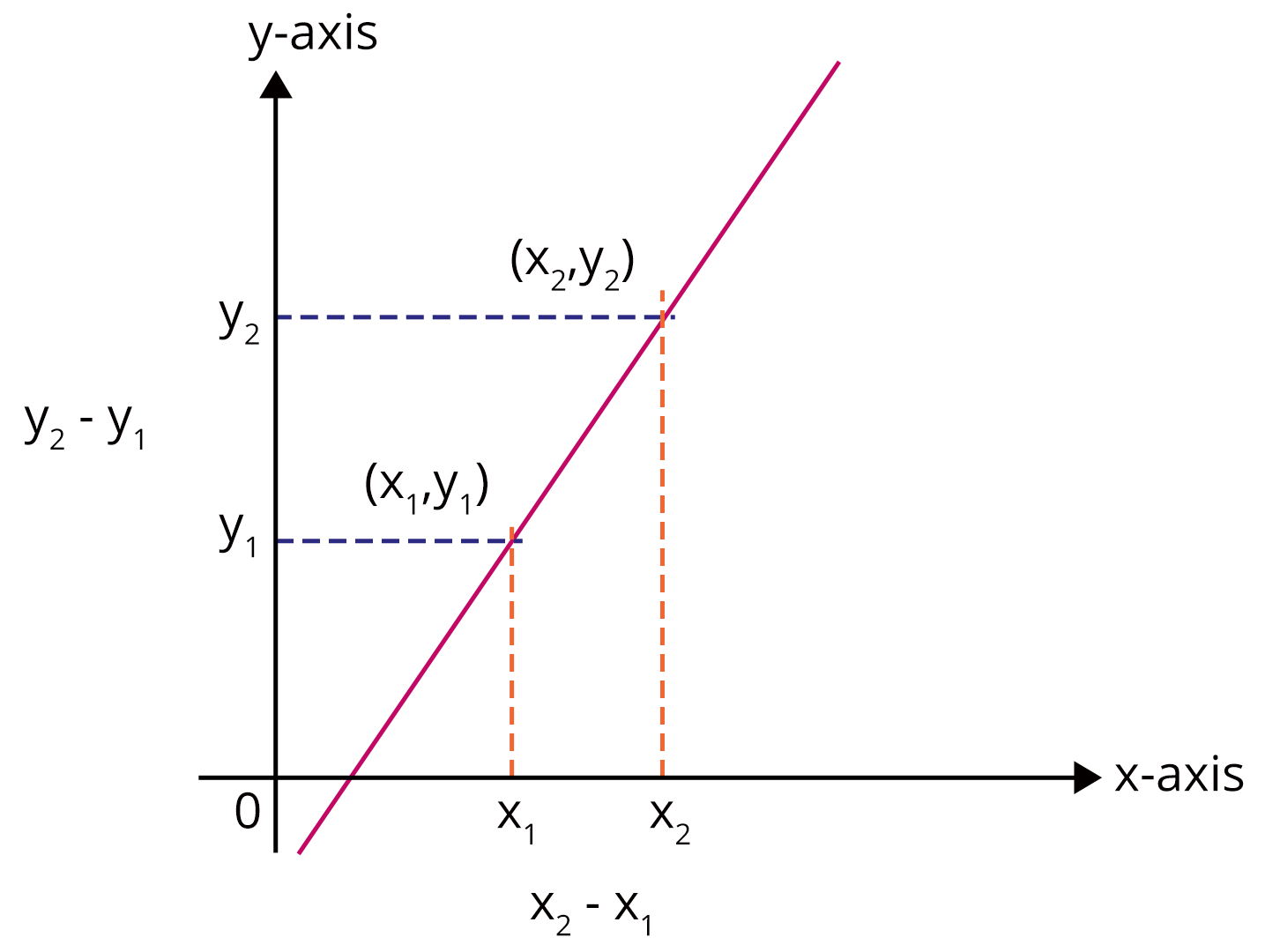
m = tanθ
$m=\dfrac{y_2 - y_1}{x_2 - x_1}$
Point Slope Form
The point-slope form of a line is a way of writing the equation of a line in terms of one point on the line and the slope of the line. The equation is in the form:
$y - y_1 = m(x - x_1)$
where:
$(x_1, y_1)$ is a point on the line
m is the slope of the line
The point-slope form of a line can be derived from the slope-intercept form of a line by substituting the coordinates of the point $(x_1, y_1)$ into the slope-intercept form equation.
To find the point-slope form of a line, you can use the following steps:
Find the slope of the line.
Choose a point on the line.
Substitute the slope and the coordinates of the point into the point-slope form equation.
$(x_1, y_1)$ is a point on the line
m is the slope of the line
Find the slope of the line.
Choose a point on the line.
Substitute the slope and the coordinates of the point into the point-slope form equation.
Mid-Point Formula
The formula for finding the midpoint of the line connecting the points $(𝑥_1,𝑦_1$) and $(𝑥_2,𝑦_2)$ is a new point, whose abscissa is the average of the x values of the two given points, and the ordinate is the average of the y values of the two given points. The midpoint is on the line that connects the two points and is right in the middle of them.
$\left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2} \right)$
Section Formula in Coordinate Geometry
The section formula is useful to find the coordinates of a point that divides the line segment joining the points $(𝑥_1,𝑦_1$) and $(𝑥_2,𝑦_2)$ in the ratio 𝑚:𝑛. The point that divides the provided two points is located on the line that connects them and can be found either between the two points or outside the line segment between them.
$(x, y)=\left( \dfrac{m x_2 + n x_1}{m + n}, \dfrac{m y_2 + n y_1}{m + n} \right)$
Locus of a Point
The locus of a point is the collection of all points that satisfy a given condition. For example, the locus of all points that are equidistant from two given points is the perpendicular bisector of the line segment connecting the two points.
$(x - h)^2 + (y - k)^2 = d^2$
The Centroid of a Triangle
The centroid of a triangle is the point of intersection of medians of a triangle. (Median is a line that joins the vertex of a triangle to the mid-point of the opposite side). The centroid of a triangle having its vertices A$(𝑥_1,𝑦_1)$, B$(𝑥_2,𝑦_2)$, and C$(𝑥_3,𝑦_3)$ is obtained from the following formula.
Centroid (G)=$\text{Centroid} \, (G) = \left( \frac{x_1 + x_2 + x_3}{3}, \frac{y_1 + y_2 + y_3}{3} \right)$
Area of a Triangle Coordinate Geometry Formula
The area of a triangle having the vertices A$(𝑥_1,𝑦_1)$, B$(𝑥_2,𝑦_2)$, and C$(𝑥_3,𝑦_3)$ is obtained from the following formula. This formula is used to find the area of a triangle for all types of triangles.
$A=\dfrac{1}{2} \left| x_1(y_2 - y_3) + x_2(y_3 - y_1) + x_3(y_1 - y_2) \right|$
How to Find Equation of a Line in Coordinate Geometry?
With the use of a simple linear equation, this line equation represents all of the points on the line, ax + by + c= 0 is the standard form of a line equation. There are several methods for determining a line's equation. The slope-intercept form of the equation of a line (y = mx + c) is another essential form of the equation of a line. The slope of the line is m, and the Y-intercept of the line is c. The equation of a line also includes other types of line equations, such as point-slope form, two-point form, intercept form, and normal form.
y = mx + c
Conic Section
Conic sections are curves that can be obtained by intersecting a plane with a cone. They are five types of conic sections: circle, ellipse, parabola, hyperbola, and degenerate conic sections (point, line, and line pair)
Properties of Conic Sections:
Circle: A circle is a conic section with a center and a radius. All points on a circle are equidistant from the center.
$(x−h)^2 +(y−k)^2 =r^2$
Ellipse: An ellipse is a conic section with two foci. The sum of the distances from any point on an ellipse to the two foci is constant.
$\dfrac{(x−h)^2}{a^2} + \dfrac{(y−k)^2}{b^2}=1$
Parabola: A parabola is a conic section with one focus. The distance from any point on a parabola to the focus is equal to the distance from the point to the directrix, which is a line parallel to the axis of the parabola.
$(y−k)^2 =4p(x−h)$
Hyperbola: A hyperbola is a conic section with two foci. The difference between the distances from any point on a hyperbola to the two foci is constant.
$\dfrac{(x−h)^2}{a^2} - \dfrac{(y−k)^2}{b^2}=1$
To understand conic sections, you can start by studying the properties of different types of curves formed by the intersection of a plane and a cone.
Eccentricity
Eccentricity is a measure of how much a conic section deviates from being a circle. It is defined as the ratio of the distance between a point on the conic section and one focus to the distance between the point and the directrix.
The eccentricity of a conic section can be calculated using the following formula:
$e = \dfrac{c}{a}$
where:
e is the eccentricity of the conic section (can never be negative)
c is the distance between a focus and the center of the conic section
a is the distance between a vertex and the center of the conic section
Circle
In coordinate geometry, a circle is defined as the set of all points that are equidistant from a given point, called the center. The distance between a point and the center of a circle is called the radius.
The standard equation of a circle with center (h,k) and radius r is given by:
$(x - h)^2 + (y - k)^2 = r^2$
This equation can be derived using the distance formula.
Important Properties of Circle:
The circumference of a circle is equal to $2\pi r$, where r is the radius of the circle.
The area of a circle is equal to $\pi r^2$, where r is the radius of the circle.
All circles are similar.
Some Important Equations of Circle
List of Important formulas for Maths Coordinate Geometry Chapter
JEE Main Maths Coordinate Geometry Solved Examples
Question 1. Find the equation of a line passing through (-2, 3) and having a slope of -1.
Solution:
The point on the line is $(x_{1},y_{1})=(-2,3)$ and the slope is m=-1.
From the coordinate geometry point and slope form of the equation of the line, we get:
$(y-y1)=m(x-x1)(y-3)=(-1)(x-(-2))y-3=-(x+2)y-3=--2x+y3-2x+y=1$
Therefore the equation of the line is: x + y = 1
Question 2. Find the equation of a line having a slope of -2 and -intercept of 1.
Solution:
The given information is m=-2and 𝑦-intercept is C=1
From coordinate geometry we can make use of the slope intercept form of the equation of a line.
$y=mx+cy=(-2)x+1y=-2x+12x+y=1$
Therefore the equation of the line we get is 2x + y = 1.
Previous year Question Paper From JEE Main
Question 1. The equations of two equal sides of an isosceles triangle are 7x − y + 3 = 0 and x + y − 3 = 0 and the third side passes through the point (1, 10). Then the equation of the third side is ___________.
Solution:
Any line passing through the point (1, 10) is given by y + 10 = m (x − 1)
Since it makes an equal angle say with the given lines 7x − y + 3 = 0 and x + y − 3 = 0, therefore tan α = m − 7 / 1 + 7m
= m − (−1) / 1 + m (−1)
⇒ m = 1/3 or 3
So, the two possible equations of the third side are: 3x + y + 7 = 0 and x − 3y − 31 = 0.
Question 2. The locus of a point P, which divides the line joining (1, 0) and (2cosθ, 2sinθ) internally in the ratio 2:3 for all θ, is a ________.
Solution:
Let us consider the coordinates of the point P, that divides the line joining (1, 0) and (2 cosθ, 2sinθ) in the ratio 2:3 be (h, k). Then,
h = 4 cosθ + 3/5 and k = 4 sinθ/5
cosθ = 5h − 3/4 and sinθ = 5k/4
$([5h − 3]/[4])^2 + ([5k]/[4])^2=1$
$(5x − 3)^2 + (5y)^2=16$
Therefore, locus of (h, k) is $(5x − 3)^2 + (5y)^2=16$, which is a circle.
Question 3. If the slope of a line passes through the point A (3, 2) is 3/4, then the points on the line which are 5 units away from A, are ___.
Solution:
The equation of line passes through the point (3, 2) and of slope 3/4 is 3x − 4y − 1 = 0
Let the point be (h, k) then,
3h−4k−1 = 0 …………..(i) and
$(h − 3)^2 + (y − 2)^2=52$ (ii)
On solving the equations above, we get h = −1, 7 and k = −1, 5.
So, the points are (1, 1) and (7, 5).
Practise Questions
Question 1. If the coordinates of vertices of a triangle are (0, 5), (1, 4) and (2, 5) then the coordinate of the circumcentre will be.
A. (1, 5)
B. (3,9)
C. (1, 4)
D. None of these
Question 2. The equation of the image of pair of rays y = |x| by the line x = 1 is
A. |y| = x + 2
B. |y| + 2 = x
C. y = |x – 2|
D. None of these
Answers: 2-A, 3-C
Tips for Studying Coordinate Geometry:
Draw Figures: When you're working on coordinate geometry problems, always draw the pictures. It makes things much clearer.
Formulas Notebook: Keep a special notebook just for formulas. Write down all the important formulas you come across while studying.
Memorize Formulas: Try to memorize as many formulas as you can. This will save you time during exams.
Read Carefully: When you're reading a question, take your time to understand what it's asking. This way, you won't make mistakes.
Stick to One Book: It's best not to use too many different books for the same topic. Stick to one main book and maybe one reference book. Using too many books can just confuse you.
Benefits of Using Vedantu for JEE Main Math - Coordinate Geometry
Vedantu transforms Maths Coordinate Geometry preparation for JEE Main with expert-guided, step-by-step clarity, interactive learning, and personalised doubt resolution. Engage in enjoyable, flexible sessions, access real-world applications, and master the subject visually for a comprehensive JEE Main readiness.
Topic-wise Clarity in Maths Coordinate Geometry: Vedantu ensures clear explanations for each topic in Coordinate Geometry, breaking down complex concepts step by step for a thorough understanding in preparation for JEE Main.
Expert Guidance for JEE Main Preparation: Expert tutors at Vedantu craft solutions, providing accurate and clear explanations for every concept in Maths Coordinate Geometry, aiding students in effective JEE Main preparation.
Interactive Learning Experience for Coordinate Geometry: Engage in interactive learning sessions tailored for Maths Coordinate Geometry, making the study process enjoyable, participative, and effective for JEE Main.
Personalised Doubt Resolution in Coordinate Geometry: Vedantu offers personalised doubt resolution during live sessions, addressing specific challenges in understanding Maths Coordinate Geometry, ensuring clarity for JEE Main aspirants.
Practice Questions and Tests for JEE Main: Access a variety of practice questions and tests in Maths Coordinate Geometry on Vedantu's platform, strengthening your grasp and thoroughly preparing for the JEE Main exam.
Flexible Learning Schedule for JEE Main Preparation: Vedantu's platform provides flexibility in your learning schedule for Maths Coordinate Geometry, allowing you to study at your own pace while preparing for JEE Main.
Real-world Applications of Coordinate Geometry: Understand the real-world applications of Coordinate Geometry through Vedantu's resources, making the subject more relatable and interesting for JEE Main aspirants.
Comprehensive Exam Preparation for JEE Main: Vedantu's resources cover the entire Maths Coordinate Geometry chapter comprehensively, offering a well-rounded approach to JEE Main preparation.
Visual Learning Aids for Coordinate Geometry: Benefit from visual learning aids in Vedantu's resources, simplifying complex concepts in Maths Coordinate Geometry, making it easier to visualise and understand while preparing for JEE Main.
JEE Main Maths - Coordinate Geometry Study Materials
Here, you'll find a comprehensive collection of study resources for Coordinate Geometry designed to help you excel in your JEE Main preparation. These materials cover various topics, providing you with a range of valuable content to support your studies. Simply click on the links below to access the study materials of Coordinate Geometry and enhance your preparation for this challenging exam.
JEE Main Maths Chapters 2026
JEE Main Maths Study and Practice Materials
Explore an array of resources in the JEE Main Maths Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Maths preparation.
Conclusion
Coordinate Geometry is recognized as one of the most fascinating mathematical concepts. Coordinate Geometry topics uses graphs with curves and lines to describe the relationship between geometry and algebra. Geometric aspects are provided in Algebra, allowing students to solve geometric problems. It is a type of geometry in which the coordinate points on a plane are expressed as an ordered pair of numbers. Here, the concepts of coordinate geometry are explained along with their formulas and solved coordinate geometry examples for better understanding.
Important Links for JEE Main and Advanced 2026
Access a curated collection of study materials and tips to excel in JEE Main and Advanced 2026, helping you prepare effectively for these prestigious engineering entrance exams.
FAQs on Coordinate Geometry for JEE Main 2026: Complete Guide
1. What is a Cartesian Plane?
A cartesian plane in coordinate geometry is made up of two perpendicular lines called the X-axis (horizontal axis) and the Y-axis (vertical axis). The ordered pair can be used to calculate the exact position of a point in the Cartesian plane (x, y).
2. Where is Coordinate Geometry Used in Maths?
Coordinate geometry has a wide range of applications in mathematics. Many applications of coordinate geometry may be found in math topics such as vectors, three-dimensional geometry, equations, calculus, complex numbers, and functions. All of these topics require the presentation of data graphically in a two or three-dimensional coordinate plane.
3. What is the Application of Coordinate Geometry Used in Real Life?
In real life, coordinate geometry is used in a variety of ways. The coordinate system takes into account all of the maps we use to pinpoint locations, including Google Maps and physical maps. Furthermore, drawing land maps to scale is beneficial in large-scale land projects. To pinpoint any place in the seas, naval engineers employ coordinate systems.
4. What are some common mistakes students make when solving problems on Coordinate Geometry?
Common mistakes students make in Coordinate Geometry:
Students often overlook fundamental concepts like the equation of a line, distance formulas, and coordinate geometry definitions. This can lead to errors in calculations and a lack of understanding of the problem.
Coordinate geometry problems often require visualizing shapes and relationships on the coordinate plane. Without proper visualization, it becomes difficult to interpret the problem and formulate an appropriate solution.
Careless mistakes in algebraic manipulations can significantly impact the solution. Students should focus on accuracy and double-check their calculations.
Some students adopt a rigid approach to problem-solving, sticking to a particular method even when it's not the most efficient or suitable. Flexibility in thinking and the ability to adapt to different problem types are crucial for success.
In coordinate geometry, units play a significant role in interpreting distances, slopes, and other quantities. Students should pay attention to the units provided in the problem and incorporate them correctly into their calculations.
5. What are some tips for solving problems on Coordinate Geometry?
Thoroughly understand fundamental concepts like equations of lines, circles, parabolas, and hyperbolas. Familiarity with these concepts will form a strong foundation for solving more complex problems.
Draw the coordinate plane and sketch the given shapes or objects. Visualization helps you understand the spatial relationships between different points and lines, making it easier to formulate equations and solve the problem.
Choose the correct formulas and apply them accurately. Double-check your calculations to ensure you haven't made any algebraic errors.
Don't limit yourself to a single method. Explore different approaches and choose the one that best suits the problem.
Always keep track of the units provided in the problem. Incorporate them correctly into your calculations and ensure your final answer is in the specified units.
6. What are some good resources provided by Vedantu for learning more about Coordinate Geometry?
Vedantu's JEE Main Coordinate Geometry Revision Notes: This comprehensive set of notes covers all the essential concepts and formulas in coordinate geometry for JEE Main. It includes clear explanations, solved examples, and practice questions.
Vedantu's JEE Main Coordinate Geometry Important Questions: This collection of practice questions is designed to help students improve their problem-solving skills and identify their areas of weakness. It includes questions from various difficulty levels and covers a wide range of topics.
Vedantu's JEE Main Coordinate Geometry Video Lectures: These interactive video lectures provide detailed explanations of important concepts and problem-solving strategies. The videos are accompanied by practice problems and interactive exercises.
Vedantu's JEE Main Coordinate Geometry Doubt Solving Forum: This online forum allows students to interact with experts and get their doubts clarified. Students can post their questions, share their solutions, and learn from the interactions of others.
7. What Is Co-Ordinate Geometry?
Coordinate Geometry is the branch of mathematics that studies geometric shapes and figures using a coordinate system, usually the Cartesian coordinate system. It allows us to represent geometric objects like points, lines, and curves with coordinates and equations.
8. How is Coordinate Geometry important for JEE?
Coordinate Geometry is a significant topic in JEE Main, covering a range of concepts like straight lines, circles, conic sections, and their equations. Mastery of this topic helps in solving various problems related to geometry efficiently.
9. What are some key Coordinate Geometry formulas with examples?
Some important Coordinate Geometry formulas include:
Distance formula: $d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}$
Midpoint formula: $M = \left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2} \right)$
Slope of a line: $m = \frac{y_2 - y_1}{x_2 - x_1}$
10. How is Coordinate Geometry applied in real life?
Coordinate Geometry is widely used in areas such as navigation systems (GPS), architecture, computer graphics, astronomy, and even in game development for positioning and motion tracking.
11. What are the main applications of Coordinate Geometry in JEE?
In JEE, Coordinate Geometry helps in solving problems related to:
Equations of straight lines, circles, and conic sections.
Finding the intersection of curves.
Understanding the geometric properties of figures like triangles, quadrilaterals, and more.
12. How can I prepare for Coordinate Geometry in JEE?
To prepare for Coordinate Geometry in JEE, practice solving problems on topics like the distance formula, midpoint formula, equation of a line, and conic sections. Understand the theoretical concepts and apply the formulas correctly in problem-solving.
13. What is the importance of Coordinate Geometry in the JEE syllabus?
Coordinate Geometry forms a significant portion of the JEE syllabus. It tests your ability to apply mathematical concepts to geometric figures and is a key part of both the JEE Main and Advanced exams.
14. What types of problems can I expect from Coordinate Geometry in JEE?
In JEE, you can expect problems based on:
Finding the distance between two points.
The equation of a line or circle.
Slope and angle between two lines.
Properties of conic sections.
Area of geometric figures using coordinates.
15. How do I apply Coordinate Geometry formulas in problem-solving?
Coordinate Geometry formulas are used to determine distances, slopes, areas, and equations of lines or curves. Identify the given coordinates and select the appropriate formula to calculate the required result, whether it’s for distance, midpoint, or line equations.























