Area of Isosceles Triangle Formula with Examples for JEE Main 2025

The formula for the area of an Isosceles Triangle helps calculate the space enclosed within its three sides, where two sides are equal in length. An isosceles triangle has a unique property of symmetry, making its area calculation straightforward. The area can be determined using the base and height or by applying Heron’s formula if all sides are known. This formula is essential in geometry for solving problems involving triangles in academics and practical applications for JEE Main 2025.

What is an Isosceles Triangle?

An Isosceles Triangle is a triangle that has two sides of equal length. These two equal sides are called the "legs," and the third side is called the "base." It also has two angles that are equal, which are the angles opposite the equal sides. Isosceles triangles are known for their symmetry and are commonly used in geometry.

By this definition, an equilateral Triangle is also an Isosceles Triangle. Let us consider an Isosceles Triangle as shown in the following diagram (whose sides are known, say a, a and b).

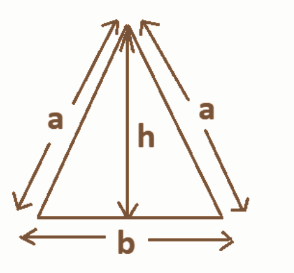
As the altitude of an Isosceles Triangle drawn from its vertical angle is also its angle bisector and the median to the base (which can be proved using congruence of Triangles), we have two right Triangles as shown in the figure above.

Derivation for Isosceles Triangle Area Using Heron’s Formula

Using the Pythagorean theorem, we have the following result.

\[AC^2=AD^2+DC^2\Rightarrow h^2=a^2-(\frac{b}{2})^2\Rightarrow h=\sqrt{a^2-\frac{b^2}{4}}\]

So the area of the Isosceles can be calculated as follows.

\[area=\frac{1}{2}bh=\frac{b}{2}\sqrt{a^2-\frac{b^2}{4}}\]

The perimeter of the Isosceles Triangle is relatively simple to calculate, as shown below.

Also, note that the area of the Isosceles Triangle can be calculated using Heron’s formula.

Area = \[\sqrt{s(s-a)(s-a)(s-b)}\]......(1)

s=\[\frac{a+a+a}{2}=a+\frac{b}{2}\]

Placing the value of ‘s’ in eq (i)

Area=\[\sqrt{(a+\frac{b}{2})(a+\frac{b}{2}-a)(a+\frac{b}{2}-a)(a+\frac{b}{2}-b)}\]

\[\sqrt{(a+\frac{b}{2})(\frac{b}{2})(\frac{b}{2})(\frac{2a-2b+b}{2})}\]

\[\sqrt{(\frac{2a+b}{2})(\frac{b^2}{4})(\frac{2a-b}{2})}\]

\[\frac{b}{2}\sqrt\frac{4a^2-b^2}{4}\]=\[\frac{b}{2}\sqrt{a^2-\frac{b^2}{4}}\]

Trigonometry can also be used in the case of Isosceles Triangles more easily because of the congruent right Triangles.

Formula of Area of Isosceles Triangle

The formula for the area of an isosceles triangle depends on the information available:

1. Using Base and Height:

If the base ($b$) and height ($h$) of the triangle are known:

$\text{Area} = \dfrac{1}{2} \times b \times h$

2. Using Side Lengths (Heron’s Formula):

If all three sides of the isosceles triangle are known (a and b, where a is the length of the equal sides and b is the base):

$\text{Area} = \sqrt{s(s-a)(s-a)(s-b)}$

where:

$s = \dfrac{2a + b}{2} \quad (\text{semi-perimeter})$

3. Using Equal Sides and Base:

If the lengths of the equal sides (a) and the base (b) are known:

$\text{Area}=\dfrac{b}{4} \times \sqrt{4a^2 - b^2}$

Let’s look at an example to see how to use these formulas.

Question: If the base and the area of an Isosceles Triangle are respectively

8cm and 12cm2, then find its perimeter.

Solution:

\[b=4cm, area=12cm^2\]

\[area=\frac{b}{2}\sqrt{a^2-\frac{b^2}{4}}\]

\[12=\frac{8}{2}\sqrt{a^2-\frac{8^2}{4}}\]

\[3=\sqrt{a^2-16}=a^2=25\Rightarrow a=5cm\]

Thus the perimeter of the Isosceles Triangle is calculated as follows.

\[perimeter=2a+b=2\times 5+8=18cm\]

Why don’t you try solving the following sum to see if you have mastered using these formulas?

Question: Calculate the area of an Isosceles Triangle whose sides are 13 cm, 13 cm and 24 cm.

Options:

1. 60cm2

2. 45cm2

3. 30cm2

4. none of these

Answer: (a)

Solution:

\[a=13cm,b=24cm\]

\[Area=\frac{b}{2}\sqrt{a^2-\frac{b^2}{4}}\]

\[Area=\frac{24}{2}\sqrt{13^2-\frac{24^2}{4}}=12=\sqrt{169-144}=12\times 5=60cm^2\]

Area of Isosceles Right Triangle Formula

The formula to calculate the area of an isosceles right triangle is straightforward. Since the two legs (perpendicular sides) are of equal length, let the length of each leg be a. The area is calculated using the formula:

$\text{Area} = \frac{1}{2} \times \text{Base} \times \text{Height}$

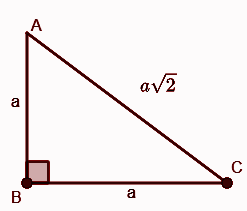
For an isosceles right triangle:

• Base = a

• Height = a

Substituting these values into the formula:

$\text{Area}=\dfrac{1}{2} \times a \times a=\dfrac{a^2}{2}$

Final Formula:

$\text{Area of Isosceles Right Triangle}=\dfrac{a^2}{2}$

This formula is specific to isosceles right triangles and applies when the two legs are equal in length.

Basic Rules About Isosceles Triangles

The triangle is constituted of three sides. But to identify a Triangle as an Isosceles Triangle it has to have some definite characteristics. One of the main characteristics of an Isosceles Triangle is that the two legs of the Triangle must be equal in length. Apart from these two, there will be the base of the Triangle that is not equal to the length of the two sides. Some other theorems consider another feature to be equally important to identify a particular Triangle as an Isosceles Triangle. According to this theorem, the angles that will be opposite to the sides of the Triangle that are equal in length will also be equal.

Purpose of Learning the Concepts of Isosceles Triangles

The students should know the basic and foundational concepts of Geometry. The concept of an Isosceles Triangle is included in the syllabus of the students so that they can develop their idea of angles and the lengths of a Triangle. Questions from each of the topics of Geometry should be included in the question papers. If the students want to score good marks in Mathematics then they should understand the concept of every chapter that is included in the syllabus.

The foundational knowledge of Geometry will be particularly helpful for those students who want to pursue their academics or want to undertake research projects in the field of Mathematics. They may have to solve questions regarding this particular chapter to qualify for various other engineering entrance examinations.

Understanding the concept of an Isosceles Triangle is important because, to pursue their career in the engineering field of studies, the students need to find out the values of unknown angles and they should be very good at determining the shapes and lengths of various objects.

Simple Methods to Prove that a Particular Triangle is Isosceles

There are some simple facts that the students need to remember to prove or identify a Triangle as an Isosceles Triangle. The first rule is to check if the two sides of the Triangle are equal in length or not. If the length of the two sides of a Triangle is equal in length, then you have to check whether the base angles of the Triangle are equal or not. The base angles signify those two angles that are formed between the base of the Triangle and the two sides of the Triangle that are equal in length. If you find that the third angle, that is the angle between the two sides of the Triangle that are equal in length, is 90 degrees, then you can conclude that this particular Triangle is a Right Isosceles Triangle.

Can Isosceles Triangles be Considered as Equilateral Triangles?

To find the answer to this particular question, you need to understand the concept of equilateral Triangles. Equilateral Triangles are those Triangles that are constituted by three sides that are equal in length. Since all three sides of an equilateral Triangle are of similar length, it is quite predictable that the angles formed between these three sides of the Triangle are also equal.

Isosceles Triangles are formed by three sides. Among these three, any two sides will be equal in length and the angles formed at the opposite of the sides will also be equal. Because of this special characteristic of Isosceles Triangles, it can be considered that every equilateral Triangle can also be an Isosceles Triangle. But every Isosceles Triangle cannot be considered an equilateral Triangle because all three angles of the Isosceles Triangle will not be equal.

Practice Questions on the Area of Isosceles Triangle

1. Find the area of an isosceles triangle with a base of 10 cm and a height of 12 cm.

2. The two equal sides of an isosceles triangle are each 13 cm, and the base is 10 cm. Calculate the area of the triangle.

3. An isosceles triangle has a base of 8 cm and an area of 24 cm². Find the height of the triangle.

4. Calculate the area of an isosceles right triangle whose equal sides are 5 cm in length.

5. If the base of an isosceles triangle is 14 cm, and its two equal sides are each 15 cm, find the area of the triangle.

6. The area of an isosceles triangle is 30 cm², and its base is 12 cm. Determine the length of its height.

7. A triangle has two equal sides of 10 cm and a base of 16 cm. What is the area of the triangle?

8. Find the area of an isosceles right triangle if the length of each leg is 7 cm.

9. An isosceles triangle has sides of 13 cm, 13 cm, and 10 cm. Calculate the height of the triangle from the base to the apex.

10. The height of an isosceles triangle is 15 cm, and the base is 18 cm. What is the area of the triangle?

Conclusion

The Area of an Isosceles Triangle can be easily calculated using a variety of formulas, depending on the available information. The most common formula involves using the base and height, where the area is given by $\dfrac{1}{2} \times \text{Base} \times \text{Height}$. Alternatively, if the sides are known, there are other formulas, such as using Heron's formula or applying a specific formula for isosceles triangles involving side lengths. By understanding these formulas, you can confidently solve problems involving isosceles triangles in various mathematical and real-world applications.

Other Subject-Wise Formula Links for JEE Main 2025

JEE Main 2025 Subject-Wise Important Chapters 

Check Other Important Links for JEE Main 2025

JEE 2025 needs well-structured study materials. Here are some valuable resources to help you plan your preparation and practice efficiently.