Question

The normal at any point \[P\] meets the axis in \[G\] and the tangent at the vertex in \[{{G}^{'}}\]. If \[A\] be the vertex and the rectangle \[AGQ{{G}^{'}}\] be completed, prove that the equation of locus of \[Q\] is
\[{{x}^{3}}=2a{{x}^{2}}+a{{y}^{2}}\]

Answer 1

Hint:Here we use the property of a rectangle that the mid-points of the diagonals of a rectangle are the same .

 Let’s consider the equation of the parabola to be \[{{y}^{2}}=4ax\]. So, its vertex is \[A(0,0)\].
We know , any point on the parabola , given by the equation \[{{y}^{2}}=4ax\], can be written as \[P\left( a{{t}^{2}},2at \right)\].



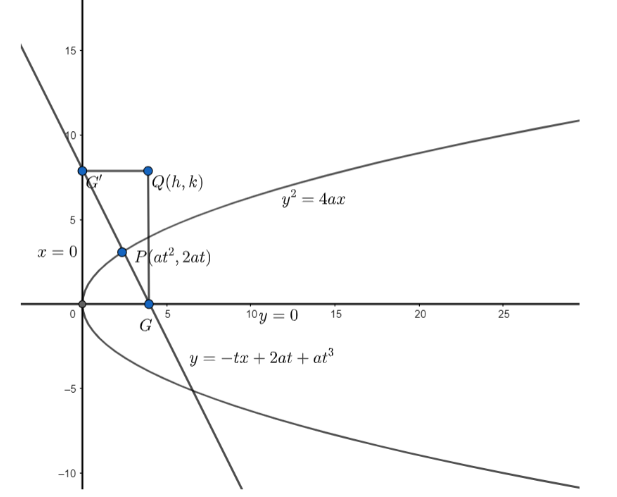
Now , we will find the equation of normal to the parabola at \[P\].
We know , the equation of the normal to the parabola in parametric form is given as
\[y=-tx+2at+a{{t}^{3}}\]
So, the equation of normal at \[P\] can be written as
\[y=-tx+2at+a{{t}^{3}}....\left( i \right)\]
Now , we know , the axis of the parabola is \[y=0\].
We need to find the point of intersection of the normal to the parabola and the axis of the parabola .
To find the point of intersection of normal and the axis , we will substitute \[y=0\] in equation \[\left( i \right)\].
On substituting \[y=0\] in equation \[\left( i \right)\] , we get
 \[0=-tx+2at+a{{t}^{3}}\]
\[\Rightarrow x=2a+a{{t}^{2}}\]
So , the coordinates of \[G\] are \[\left( 2a+a{{t}^{2}},0 \right)\].
Now , we know , tangent to the parabola at vertex is given by the equation \[x=0\].
So , the point of intersection of normal and tangent at vertex can be found by substituting \[x=0\]in \[\left( i \right)\].
On substituting \[x=0\]in \[\left( i \right)\], we get
\[y=2at+a{{t}^{3}}\]
So, \[{{G}^{'}}=\left( 0,2at+a{{t}^{3}} \right)\]
Now , since we need to find the locus of \[Q\], let \[Q=\left( h,k \right)\].
Now , it is given that \[AGQ{{G}^{'}}\]is a rectangle.
We know the diagonals of a rectangle bisect each other.
So , the midpoint of the diagonals \[AQ\] and \[G{{G}^{'}}\] is the same.
Now, we know that the coordinates of the midpoint of the line joining two points \[\left( {{x}_{1}},{{y}_{1}} \right)\] and \[\left( {{x}_{2}},{{y}_{2}} \right)\] is given as: \[\left( \dfrac{{{x}_{1}}+{{x}_{2}}}{2},\dfrac{{{y}_{1}}+{{y}_{2}}}{2} \right)\]
So, midpoint of \[AQ=\left( \dfrac{0+h}{2},\dfrac{0+k}{2} \right)\]
\[=\left( \dfrac{h}{2},\dfrac{k}{2} \right)....\left( ii \right)\]
Midpoint of \[G{{G}^{'}}=\left( \dfrac{2a+a{{t}^{2}}+0}{2},\dfrac{0+2at+a{{t}^{3}}}{2} \right)\]
\[=\left( \dfrac{2a+a{{t}^{2}}}{2},\dfrac{2at+a{{t}^{3}}}{2} \right)....\left( iii \right)\]
Comparing \[\left( ii \right)\] with \[\left( iii \right)\],
\[\dfrac{h}{2}=\dfrac{2a+a{{t}^{2}}}{2}\Rightarrow h=2a+a{{t}^{2}}....\left( iv \right)\]
\[\dfrac{k}{2}=\dfrac{2at+a{{t}^{3}}}{2}\Rightarrow k=2at+a{{t}^{3}}....\left( v \right)\]
On dividing \[\left( v \right)\]by \[\left( iv \right)\], we get
\[\dfrac{k}{h}=\dfrac{2at+a{{t}^{3}}}{2a+a{{t}^{2}}}\]
\[\Rightarrow \dfrac{k}{h}=t\]
Now, let’s substitute \[t=\dfrac{k}{h}\]in \[\left( iv \right)\]. We get ,
\[h=2a+a{{\left( \dfrac{k}{h} \right)}^{2}}\]
\[\Rightarrow h=\dfrac{2a{{h}^{2}}+a{{k}^{2}}}{{{h}^{2}}}\]
Or, \[{{h}^{3}}=2a{{h}^{2}}+a{{k}^{2}}........\]equation\[(vi)\]
Now, to find the locus of \[Q\left( h,k \right)\], we will substitute \[(x,y)\]in place of \[\left( h,k \right)\] in equation \[(vi)\]
So, the locus of \[Q\left( h,k \right)\] is \[{{x}^{3}}=2a{{x}^{2}}+a{{y}^{2}}\]
Note: Vertex of \[{{y}^{2}}=4ax\]is \[\left( 0,0 \right)\].
Tangent at vertex is \[x=0\]
Equation of the axis is \[y=0\].
Students generally get confused between the equation of tangent at vertex and the equation of axis.