Answer
329.9k+ views
HINT- Proceed the solution of this question by doing prime factor of given number then try to form triplets of prime numbers, if we get triplets of all prime numbers then it will be a perfect cube and multiplication of all prime numbers will be our cube root.
Complete step-by-step answer:
We know that,
The process of cubing is similar to squaring, only that the number is multiplied three times instead of two. The exponent used for cubes is 3. for examples are 8³ = 8*8*8 = 512.
In prime factorisation method-
we will find prime factors of 27000, if it is a perfect cube and then will pair them in a group of three.
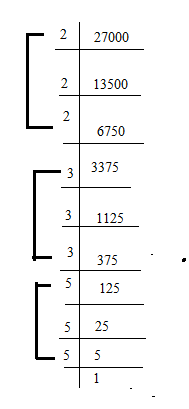
⇒27000=2×2×2×3×3×3×5×5×5
On forming pairs of three
⇒ 27000=${2^3} \times {3^3} \times {5^3}$
Since 2, 3 and 5 occurs in triplets
\[\therefore \] 27000 is a perfect cube
⇒27000=${2^3} \times {3^3} \times {5^3}$
It can be write like,
⇒27000=${\left( {2 \times 3 \times 5} \right)^3}$
On taking cube root on both side
$ \Rightarrow \sqrt[3]{{\left( {27000} \right)}} = \sqrt[3]{{{{\left( {2 \times 3 \times 5} \right)}^3}}}$
Cube root can be write raised to power $\frac{1}{3}$
\[ \Rightarrow \sqrt[3]{{27000}} = {\left( {2 \times 3 \times 5} \right)^{3 \times \frac{1}{3}}}\]
On cancelling exponential power
⇒ So cube root of 27000 = 2×3×5=30
Note- We should know that the cubic function is a one-to-one function. This is because cubing a negative number results in an answer different to that of cubing its positive counterpart. We can say this because when three negative numbers are multiplied together, two of the negatives are cancelled but one remains, so the result is also negative. 8³ = 8*8*8 = 512 and (-8) ³ = (-8) *(-8) *(-8) = -512.
Complete step-by-step answer:
We know that,
The process of cubing is similar to squaring, only that the number is multiplied three times instead of two. The exponent used for cubes is 3. for examples are 8³ = 8*8*8 = 512.
In prime factorisation method-
we will find prime factors of 27000, if it is a perfect cube and then will pair them in a group of three.
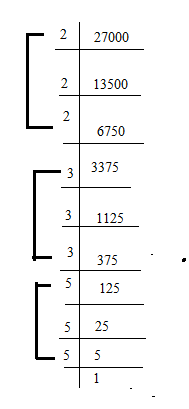
⇒27000=2×2×2×3×3×3×5×5×5
On forming pairs of three
⇒ 27000=${2^3} \times {3^3} \times {5^3}$
Since 2, 3 and 5 occurs in triplets
\[\therefore \] 27000 is a perfect cube
⇒27000=${2^3} \times {3^3} \times {5^3}$
It can be write like,
⇒27000=${\left( {2 \times 3 \times 5} \right)^3}$
On taking cube root on both side
$ \Rightarrow \sqrt[3]{{\left( {27000} \right)}} = \sqrt[3]{{{{\left( {2 \times 3 \times 5} \right)}^3}}}$
Cube root can be write raised to power $\frac{1}{3}$
\[ \Rightarrow \sqrt[3]{{27000}} = {\left( {2 \times 3 \times 5} \right)^{3 \times \frac{1}{3}}}\]
On cancelling exponential power
⇒ So cube root of 27000 = 2×3×5=30
Note- We should know that the cubic function is a one-to-one function. This is because cubing a negative number results in an answer different to that of cubing its positive counterpart. We can say this because when three negative numbers are multiplied together, two of the negatives are cancelled but one remains, so the result is also negative. 8³ = 8*8*8 = 512 and (-8) ³ = (-8) *(-8) *(-8) = -512.
Recently Updated Pages
The branch of science which deals with nature and natural class 10 physics CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Define absolute refractive index of a medium

Find out what do the algal bloom and redtides sign class 10 biology CBSE

Prove that the function fleft x right xn is continuous class 12 maths CBSE

Find the values of other five trigonometric functions class 10 maths CBSE

Trending doubts
Difference Between Plant Cell and Animal Cell

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

Write an application to the principal requesting five class 10 english CBSE

What organs are located on the left side of your body class 11 biology CBSE

What is the z value for a 90 95 and 99 percent confidence class 11 maths CBSE



