Projection

Our lives are made more ordered and less confusing by mathematics. Power of reasoning, creativity, logical thinking, critical thinking, problem-solving abilities, and even excellent communication skills are some of the attributes that mathematics generates.

It is believed that shadows produced by objects on the ground are where the concept of projection in mathematics first emerged. A geometric projection is like a shadow that an object casts onto another object. When a three-dimensional sphere is projected onto a plane, its projection will be a circle or an ellipse. In this article, we will learn about the projection of a sphere onto the plane.

What is Projection?

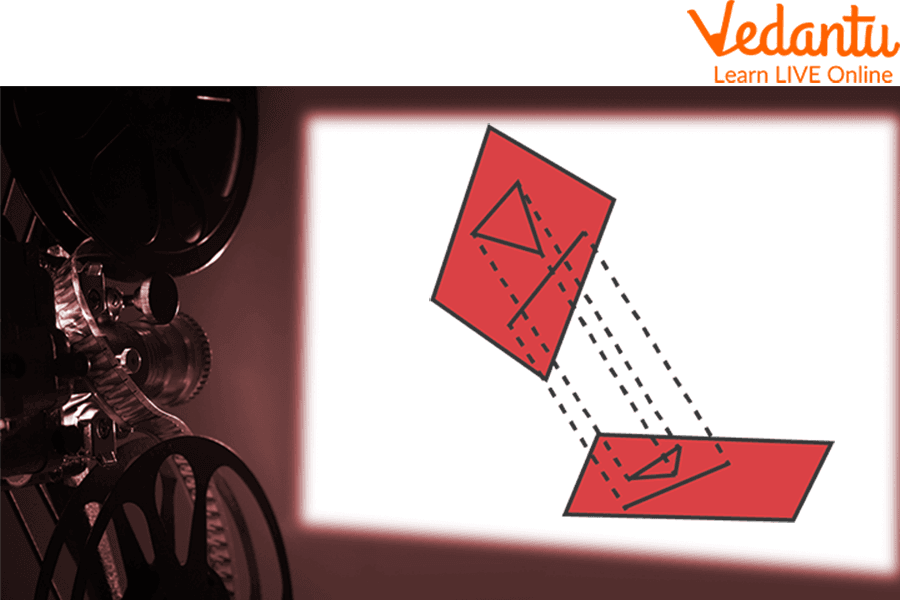
Projection Using Figures

Geometric projection is the area of geometry that deals with the characteristics and rules of geometric shapes when they are projected. By linking corresponding points on the two planes with parallel lines, a projection is the conversion of points and lines in one plane onto another. This can be seen as creating an image of whatever is drawn on one sheet of paper on another by shining a (point) light source (placed at infinity) through the translucent sheet of paper.

Projection Example in Real Life

Camera: The projective plane is extremely useful in image registration, especially in camera resectioning. To convert a 3D camera image into a 2D one, we need to convert it from 3D space to 2D space. By using projective geometry, this transformation can be described as a linear mapping from 4D to 3D.

Computer: In computer vision, projective geometry is widely used to transform a photograph (a 2D perspective view of a 3D world) into a projective transformation. Therefore, due to their projective limitations, planar images can only provide limited geographic information.

Projection of a Sphere onto the Plane

A mathematical method called sphere projection. Let us visualise the sphere's points on the plane.

There are many different projection types, and because the sphere and the plane are not locally identical, these projections are affected to different degrees. One selects a suitable projection type, considering its characteristics, depending on the goal.

Example: Globe Map on a Plane sheet

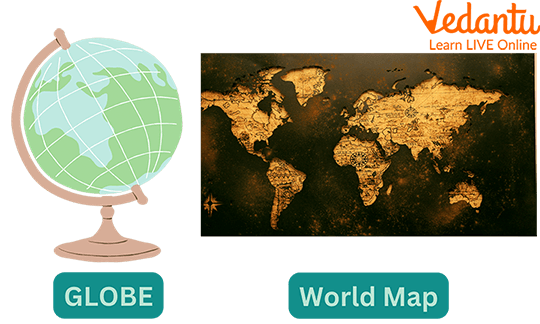
The Projection of the Content of a Spherical Globe on a Plane Surface

The magnitude of the area of a region on the map is identical to the area of the same region depicted on the sphere, which is an example of a map that maintains areas. The projection, however, is not uniform.

When two curves intersect at a single point and form an angle, their projections cross at that same point and form an angle with the same magnitude. When a projection possesses this property, it is said to be symmetric.

Map Projection Facts

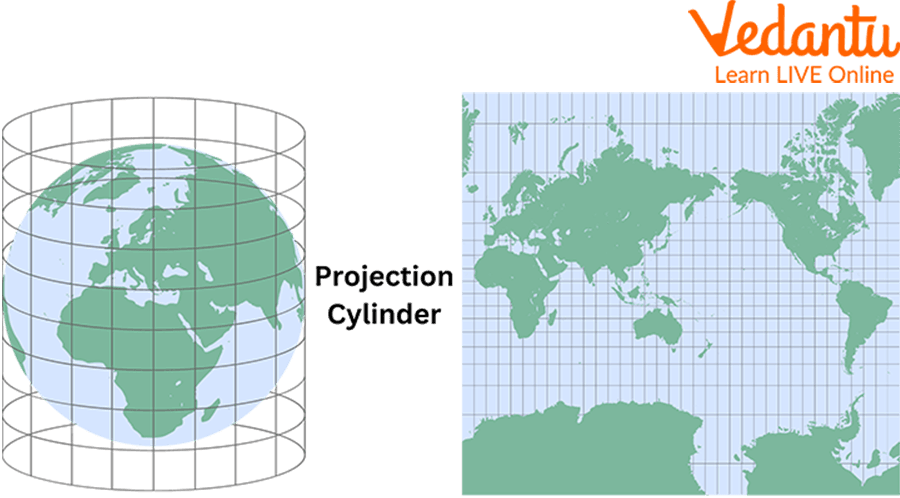
Miller Cylindrical Projection

• Miller's cylindrical projection is used to depict a cylinder on the globe.

• Despite being oriented around a different axis, this transverse Mercator projection is mathematically equivalent to a normal map.

• A sinusoidal projection depicts relative sizes accurately but severely distorts forms. The map can be "disrupted," reducing distortion.

• Shapes and sizes are distorted elsewhere, while distances and directions from the central point are precisely depicted.

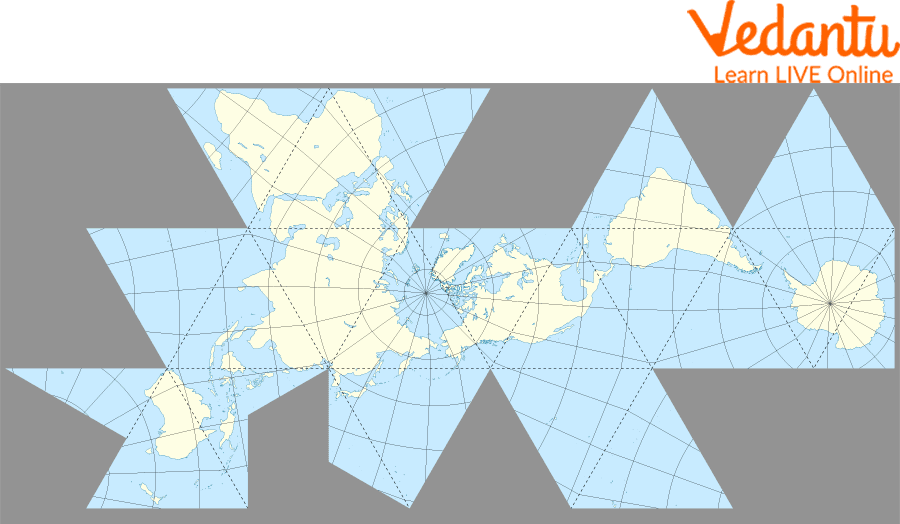
Dymaxion Map by Buckminster Fuller

• This is how the Dymaxion map by Buckminster Fuller looked. While conformal and perspective, a stereographic projection is neither equal-area nor equidistant.

• Thales created the Gnomonic projection in the sixth century BC, regarded as the oldest map projection.

Summary

Projective geometry makes understanding some geometric objects easier by neglecting measurements like distances and angles. Since then, many more complex fields have adopted these insights. Because capturing a photograph (a 2D perspective view of a 3D world) corresponds to a projective transformation, computer vision is widely employed. In a sphere, the term "projected area" refers to measuring a three-dimensional object's area in two dimensions.