Question

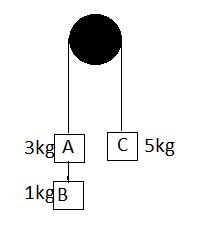
Three weights A, B and C are connected by string as shown in the figure. The system moves over a frictionless pulley. The tension in the string connecting A and B is (where $g$ is acceleration due to gravity)

(A) $g$
(B) ${\displaystyle \frac{g}{9}}$
(C) ${\displaystyle \frac{8g}{9}}$
(D) ${\displaystyle \frac{10g}{9}}$

Answer 1

Hint To make the problem simpler we first draw the free body diagram of the entire system. First, the acceleration with which the weights are moving is found by dividing net force with net mass. Then we find all the forces acting on weight B. Using the value of acceleration found earlier we find the net force acting on weight B and find the tension between weights A and B.

Complete step by step answer:
The free-body diagram of the system is as follows,

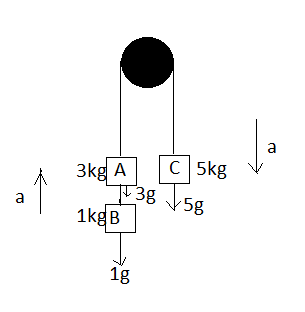
The weight on the right side is heavier than the left side. Due to this, the weights on the left side move up and weights on the right side move down with the same acceleration.
From newton’s second law of motion
$F=ma$
$F_{net}=m_{net}a$
The net force acting due to the weights will be,
$F_{net}=5g-3g-1g=1g$
The negative sign for the left side forces is due to the direction.
The net mass of the weights will be,
$m_{net}=5+3+1=9kg$
Substituting in newton’s second law
$F_{net}=m_{net}a$
$a={\displaystyle \frac{F_{net}}{m_{net}}}={\displaystyle \frac{1g}{9}}={\displaystyle \frac{g}{9}}m/s$
Now, isolating the weight B and making a separate free-body diagram of weight B,
The net forces acting on weight B are
$T-ma=mg$
 $\Rightarrow T=mg-ma$
Weight B is being accelerated with an acceleration of ${\displaystyle \frac{g}{9}}$
Therefore, the equation of net forces become
$T=(1\times g)+(1\times {\displaystyle \frac{g}{9}})={\displaystyle \frac{10g}{9}}$
Hence the tension force between weights (a) and (b) is equal to ${\displaystyle \frac{10g}{9}}$

Option (D) ${\displaystyle \frac{10g}{9}}$ is the correct answer.

Note We should be careful while finding the acceleration. The acceleration of all the bodies will be the same because the weights move by a common string. This is why we find the acceleration in the entire system and apply it to a particular weight.