
Answer
91.2k+ views
Hint: First we draw a square inscribed in a circle, then form a diameter in the square of ‘p’ cm then by using Pythagoras theorem calculate any side of the square. And finally find the area of square to get the final answer by using the formula : Area of square = ${\left( {{\text{side}}} \right)^2}$
Formula: Pythagoras Theorem
$A{B^2} + B{C^2} = A{C^2}$
And area of square = ${\left( {{\text{side}}} \right)^2}$
Complete step-by-step solution
Consider the diagram of the square inscribed circle with diameter of p cm.
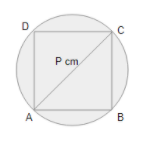
Given that the diameter of the circle is p cm. so the diagonal of the square is also p cm
Now by using the formula of Pythagoras theorem
Pythagoras theorem- A theorem attributed to the Pythagoras that the square on the hypotenuse of a right angled triangle is equal to the sum of squares on the other two sides.
$
A{B^2} + B{C^2} + A{C^2} \\
A{B^2} + A{B^2} = {P^2} \\
\Rightarrow 2A{B^2} = {P^2} \\
\Rightarrow AB = \dfrac{P}{{\sqrt 2 }} \\
$
Now the area of square is
Area= square of side
\[
\Rightarrow \dfrac{p}{{\sqrt 2 }} \times \dfrac{p}{{\sqrt 2 }} \\
\Rightarrow \dfrac{{{p^2}}}{2} \\
\]
So the area is $\dfrac{{{p^2}}}{2}{\text{cm sq}}$
Hence option C is the correct answer.
Note- First we draw a diagram according to the question and draw a diameter in it of P cm, after that using Pythagoras theorem find the side of square and then from this find the area of square and answer is $\dfrac{{{p^2}}}{2}{\text{cm sq}}$.
Formula: Pythagoras Theorem
$A{B^2} + B{C^2} = A{C^2}$
And area of square = ${\left( {{\text{side}}} \right)^2}$
Complete step-by-step solution
Consider the diagram of the square inscribed circle with diameter of p cm.

Given that the diameter of the circle is p cm. so the diagonal of the square is also p cm
Now by using the formula of Pythagoras theorem
Pythagoras theorem- A theorem attributed to the Pythagoras that the square on the hypotenuse of a right angled triangle is equal to the sum of squares on the other two sides.
$
A{B^2} + B{C^2} + A{C^2} \\
A{B^2} + A{B^2} = {P^2} \\
\Rightarrow 2A{B^2} = {P^2} \\
\Rightarrow AB = \dfrac{P}{{\sqrt 2 }} \\
$
Now the area of square is
Area= square of side
\[
\Rightarrow \dfrac{p}{{\sqrt 2 }} \times \dfrac{p}{{\sqrt 2 }} \\
\Rightarrow \dfrac{{{p^2}}}{2} \\
\]
So the area is $\dfrac{{{p^2}}}{2}{\text{cm sq}}$
Hence option C is the correct answer.
Note- First we draw a diagram according to the question and draw a diameter in it of P cm, after that using Pythagoras theorem find the side of square and then from this find the area of square and answer is $\dfrac{{{p^2}}}{2}{\text{cm sq}}$.
Recently Updated Pages
Name the scale on which the destructive energy of an class 11 physics JEE_Main

Write an article on the need and importance of sports class 10 english JEE_Main

Choose the exact meaning of the given idiomphrase The class 9 english JEE_Main

Choose the one which best expresses the meaning of class 9 english JEE_Main

What does a hydrometer consist of A A cylindrical stem class 9 physics JEE_Main

A motorcyclist of mass m is to negotiate a curve of class 9 physics JEE_Main

Other Pages
Velocity of car at t 0 is u moves with a constant acceleration class 11 physics JEE_Main

If a wire of resistance R is stretched to double of class 12 physics JEE_Main

Chloroform reacts with oxygen in the presence of light class 12 chemistry JEE_Main

A passenger in an aeroplane shall A Never see a rainbow class 12 physics JEE_Main

Formula for number of images formed by two plane mirrors class 12 physics JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main



