Question

Three forces ${F_1}$, ${F_2}$ and ${F_3}$ together keep a body is equilibrium if ${F_1} = 3N$ along the positive X-axis ${F_2} = 4N$ along the positive Y-axis, then the third force ${F_3}$ is:
A) $5N$ making an angle $\theta = {\tan ^{ - 1}}\left( {\dfrac{3}{4}} \right)$with negative Y-axis.
B) $5N$ making an angle $\theta = {\tan ^{ - 1}}\left( {\dfrac{4}{3}} \right)$with negative Y-axis.
C) $7N$ making an angle $\theta = {\tan ^{ - 1}}\left( {\dfrac{3}{4}} \right)$with negative Y-axis.
D) $7N$ making an angle $\theta = {\tan ^{ - 1}}\left( {\dfrac{4}{3}} \right)$with negative Y-axis.

Answer 1

hint: We can solve this question by using simple vector laws of addition if a body is in equilibrium its means that the net force on that body must be zero.

Complete step by step solution:
In question it is given force ${F_1} = 3N$ in positive x-axis direction we can write this force in vector form
${\vec F_1} = 3\hat i$
And in question ${F_2}$ is given $4N$ in the direction of positive y-axis in vector form it can written as
${\vec F_2} = 4\hat j$
We want to find ${\vec F_3}$
If the body is in equilibrium means the net force on the body must be zero
Or can say that the vector sum of all three forces is zero net force ${\vec F_{net}} = 0\hat i + 0\hat j + 0\hat k$
$ \Rightarrow {\vec F_1} + {\vec F_2} + {\vec F_3} = {\vec F_{net}}$
Put values of forces
$ \Rightarrow 3\hat i + 4\hat j + {\vec F_3} = 0\hat i + 0\hat j + 0\hat k$
Solving this
$ \Rightarrow {\vec F_3} = - 3\hat i - 4\hat j$ .......... (1)
Equation (1) gives the vector form of ${F_3}$
Magnitude of any force given as $\left| {\vec F} \right| = \sqrt {{{\left( {{F_x}} \right)}^2} + {{\left( {{F_y}} \right)}^2} + {{\left( {{F_z}} \right)}^2}} $
Magnitude of ${F_3}$ can be calculate by equation (1)
$ \Rightarrow \left| {{{\vec F}_3}} \right| = \sqrt {{{\left( { - 3} \right)}^2} + {{\left( { - 4} \right)}^2}} $
$ \Rightarrow {F_3} = \sqrt {9 + 16} $
$ \Rightarrow {F_3} = \sqrt {25} $
So ${F_3}$
${F_3} = 5N$
To find the direction we draw ${\vec F_3} = - 3\hat i - 4\hat j$ at origin as given


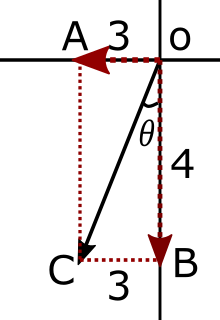
Let assume the force ${F_3}$ make angle $\theta $ with negative y-axis then
$ \Rightarrow \tan \theta = \dfrac{P}{B}$
From diagram it is clear that in triangle OBC perpendicular is $3$ and base is $4$
$ \Rightarrow \tan \theta = \left( {\dfrac{3}{4}} \right)$
So the value of $\theta $
$ \Rightarrow \theta = {\tan ^{ - 1}}\left( {\dfrac{3}{4}} \right)$
Hence the force ${F_3}$ is $7N$ at angle $\theta = {\tan ^{ - 1}}\left( {\dfrac{3}{4}} \right)$ with the negative y-axis.

Hence option (A) is correct.

Note: We can solve this question by another method first we calculate vector sum of ${F_1}$ and ${F_2}$ as given below
$ \Rightarrow {\vec F_{12}} = 3\hat i + 4\hat j$
We know body is in equilibrium so then net force is zero means the third force must be equal and opposite to the ${\vec F_{12}}$ so that this cancel the resultant of ${F_1}$ and ${F_2}$ means
$ \Rightarrow {\vec F_3} = - \left( {3\hat i + 4\hat j} \right)$
By this we can calculate magnitude and direction as calculated in the above solution.