Answer
64.8k+ views
Hint: The rate of change of the charge is represented in terms of time in exponential form. Find the charge by integration. Put the value of charge at time zero from the graph and from it find the value of integration constant and also the constant term $k$ .
The equation of charge and time put the value of times given in the problem and calculate the charges respectively.
Complete step by step answer:
The rate of change of charge s given in terms of time, $\dfrac{{dQ}}{{dt}} = - ak{e^{kt}}$
By integration we get,
$Q = - ak\dfrac{{{e^{kt}}}}{k} + c$
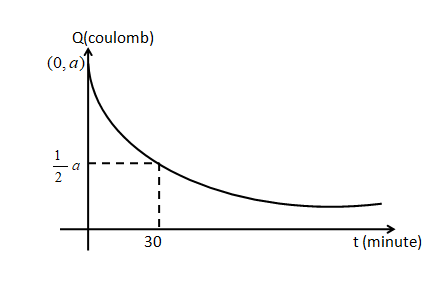
The graph shown below is the relation between the charge and the time. The charge \[Q\] is in the coulomb unit and the time \[t\] is in a minute.
To get the equation of the charge we have to integrate by separation of variables method, as follows
$\int {dQ} = - ak\int {{e^{kt}}dt} + c$ where, $c$ is the integrating constant.
$ \Rightarrow Q = - ak\dfrac{{{e^{kt}}}}{k} + c$
$ \Rightarrow Q = - a{e^{kt}} + c$…………….(1)
Now from the graph, we get, at $t = 0,{\text{ }}Q = a$
So, from eq. (1)
$ \Rightarrow a = - a{e^0} + c$
$ \Rightarrow a = - a \times 1 + c$
$ \Rightarrow c = 2a$
Hence the eq. (1) $ \Rightarrow Q = - a{e^{kt}} + 2a$ ……………..(2) [ putting the value of $c$]
From the graph, it is shown that, at $t = 30,{\text{ }}Q = \dfrac{a}{2}$
So, the eq.(2) $ \Rightarrow \dfrac{a}{2} = - a{e^{30k}} + 2a$
$ \Rightarrow a{e^{30k}} = 2a - \dfrac{a}{2}$
On taking LCM we get,
$ \Rightarrow a{e^{30k}} = \dfrac{{3a}}{2}$
On cancelling the term we get,
$ \Rightarrow {e^{30k}} = \dfrac{3}{2}$
Taking $\ln $ both sides of the above equation,
$ \Rightarrow 30k = \ln \dfrac{3}{2}$
Let us divide \[30\] on both sides we get,
$ \Rightarrow k = \dfrac{1}{{30}}\ln \dfrac{3}{2}$ .
Putting the value of $k$ in eq. (2) we get,
$ \Rightarrow Q = - a{e^{\dfrac{t}{{30}}\ln \dfrac{3}{2}}} + 2a$ ……….(3)
Now we have to calculate the charge at time $(a){\text{ }}1{\text{ hour}}$ and $(b){\text{ 80 minutes}}$
$(A) \Rightarrow 1{\text{ hour}} = 60{\text{ minutes}}$
So, eq.(3) $ \Rightarrow Q\left| {_{t = 60}} \right. = - a{e^{\dfrac{{60}}{{30}}\ln \dfrac{3}{2}}} + 2a$
$ \Rightarrow Q\left| {_{t = 60}} \right. = - a{e^{2\ln \dfrac{3}{2}}} + 2a$
$ \Rightarrow Q\left| {_{t = 60}} \right. = - a{e^{\ln {{\left( {\dfrac{3}{2}} \right)}^2}}} + 2a$
On squaring the term and we get
$ \Rightarrow Q\left| {_{t = 60}} \right. = - a{e^{\ln \dfrac{9}{4}}} + 2a$
On canceling the exponents and logarithm we get,
$ \Rightarrow Q\left| {_{t = 60}} \right. = - \dfrac{{9a}}{4} + 2a$
Taking LCM we get
$ \Rightarrow Q\left| {_{t = 60}} \right. = \dfrac{{8a - 9a}}{4}$
Let us subtract the numerator term we get,
$ \Rightarrow Q\left| {_{t = 60}} \right. = - \dfrac{a}{4}$
$(B) \Rightarrow 80{\text{ minutes}}$
So, eq.(3) $ \Rightarrow Q\left| {_{t = 80}} \right. = - a{e^{\dfrac{{80}}{{30}}\ln \dfrac{3}{2}}} + 2a$
\[ \Rightarrow Q\left| {_{t = 80}} \right. = - a{e^{2.66\ln \left( {\dfrac{3}{2}} \right)}} + 2a\]
\[ \Rightarrow Q\left| {_{t = 80}} \right. = - a{e^{\ln 2.94}} + 2a\]
[since, ${\left( {\dfrac{3}{2}} \right)^{2.66}} = 2.94$ ]
\[ \Rightarrow Q\left| {_{t = 80}} \right. = - 2.94a + 2a\]
On subtracting we get,
\[ \Rightarrow Q\left| {_{t = 80}} \right. = - 0.94a\].
Hence the answers for,
$(A){\text{ }} \Rightarrow Q\left| {_{t = 60}} \right. = - \dfrac{a}{4}$and $(B) \Rightarrow Q\left| {_{t = 80}} \right. = - 0.94a$.
Additional information:
If a capacitor is connected to a battery, then the charges are gathered in that capacitor. This is known as the charging of the capacitor. When the batteries are disconnected from the capacitor, the capacitor loses charges. This is called the discharging of the capacitor.
Note: The rate of change of the charge is given, $\dfrac{{dQ}}{{dt}} = - ak{e^{kt}}$
Here the negative sign implies that the charge decreases with the increase of time.
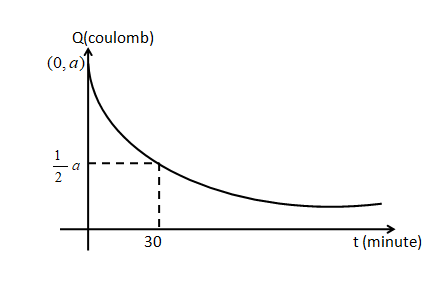
From the graph, we see that the charge decays exponentially. It takes infinite time to be fully discharged.
The equation of charge and time put the value of times given in the problem and calculate the charges respectively.
Complete step by step answer:
The rate of change of charge s given in terms of time, $\dfrac{{dQ}}{{dt}} = - ak{e^{kt}}$
By integration we get,
$Q = - ak\dfrac{{{e^{kt}}}}{k} + c$
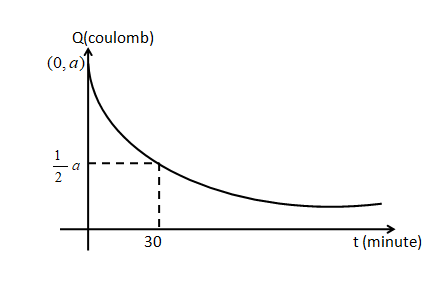
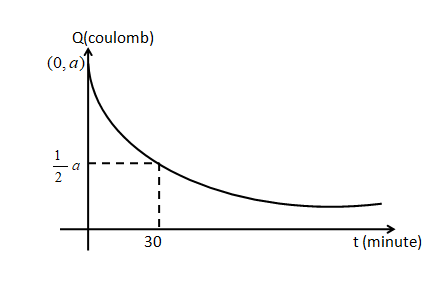
The graph shown below is the relation between the charge and the time. The charge \[Q\] is in the coulomb unit and the time \[t\] is in a minute.

To get the equation of the charge we have to integrate by separation of variables method, as follows
$\int {dQ} = - ak\int {{e^{kt}}dt} + c$ where, $c$ is the integrating constant.
$ \Rightarrow Q = - ak\dfrac{{{e^{kt}}}}{k} + c$
$ \Rightarrow Q = - a{e^{kt}} + c$…………….(1)
Now from the graph, we get, at $t = 0,{\text{ }}Q = a$

So, from eq. (1)
$ \Rightarrow a = - a{e^0} + c$
$ \Rightarrow a = - a \times 1 + c$
$ \Rightarrow c = 2a$
Hence the eq. (1) $ \Rightarrow Q = - a{e^{kt}} + 2a$ ……………..(2) [ putting the value of $c$]
From the graph, it is shown that, at $t = 30,{\text{ }}Q = \dfrac{a}{2}$
So, the eq.(2) $ \Rightarrow \dfrac{a}{2} = - a{e^{30k}} + 2a$
$ \Rightarrow a{e^{30k}} = 2a - \dfrac{a}{2}$
On taking LCM we get,
$ \Rightarrow a{e^{30k}} = \dfrac{{3a}}{2}$
On cancelling the term we get,
$ \Rightarrow {e^{30k}} = \dfrac{3}{2}$
Taking $\ln $ both sides of the above equation,
$ \Rightarrow 30k = \ln \dfrac{3}{2}$
Let us divide \[30\] on both sides we get,
$ \Rightarrow k = \dfrac{1}{{30}}\ln \dfrac{3}{2}$ .
Putting the value of $k$ in eq. (2) we get,
$ \Rightarrow Q = - a{e^{\dfrac{t}{{30}}\ln \dfrac{3}{2}}} + 2a$ ……….(3)
Now we have to calculate the charge at time $(a){\text{ }}1{\text{ hour}}$ and $(b){\text{ 80 minutes}}$
$(A) \Rightarrow 1{\text{ hour}} = 60{\text{ minutes}}$
So, eq.(3) $ \Rightarrow Q\left| {_{t = 60}} \right. = - a{e^{\dfrac{{60}}{{30}}\ln \dfrac{3}{2}}} + 2a$
$ \Rightarrow Q\left| {_{t = 60}} \right. = - a{e^{2\ln \dfrac{3}{2}}} + 2a$
$ \Rightarrow Q\left| {_{t = 60}} \right. = - a{e^{\ln {{\left( {\dfrac{3}{2}} \right)}^2}}} + 2a$
On squaring the term and we get
$ \Rightarrow Q\left| {_{t = 60}} \right. = - a{e^{\ln \dfrac{9}{4}}} + 2a$
On canceling the exponents and logarithm we get,
$ \Rightarrow Q\left| {_{t = 60}} \right. = - \dfrac{{9a}}{4} + 2a$
Taking LCM we get
$ \Rightarrow Q\left| {_{t = 60}} \right. = \dfrac{{8a - 9a}}{4}$
Let us subtract the numerator term we get,
$ \Rightarrow Q\left| {_{t = 60}} \right. = - \dfrac{a}{4}$
$(B) \Rightarrow 80{\text{ minutes}}$
So, eq.(3) $ \Rightarrow Q\left| {_{t = 80}} \right. = - a{e^{\dfrac{{80}}{{30}}\ln \dfrac{3}{2}}} + 2a$
\[ \Rightarrow Q\left| {_{t = 80}} \right. = - a{e^{2.66\ln \left( {\dfrac{3}{2}} \right)}} + 2a\]
\[ \Rightarrow Q\left| {_{t = 80}} \right. = - a{e^{\ln 2.94}} + 2a\]
[since, ${\left( {\dfrac{3}{2}} \right)^{2.66}} = 2.94$ ]
\[ \Rightarrow Q\left| {_{t = 80}} \right. = - 2.94a + 2a\]
On subtracting we get,
\[ \Rightarrow Q\left| {_{t = 80}} \right. = - 0.94a\].
Hence the answers for,
$(A){\text{ }} \Rightarrow Q\left| {_{t = 60}} \right. = - \dfrac{a}{4}$and $(B) \Rightarrow Q\left| {_{t = 80}} \right. = - 0.94a$.
Additional information:
If a capacitor is connected to a battery, then the charges are gathered in that capacitor. This is known as the charging of the capacitor. When the batteries are disconnected from the capacitor, the capacitor loses charges. This is called the discharging of the capacitor.
Note: The rate of change of the charge is given, $\dfrac{{dQ}}{{dt}} = - ak{e^{kt}}$
Here the negative sign implies that the charge decreases with the increase of time.

From the graph, we see that the charge decays exponentially. It takes infinite time to be fully discharged.
Recently Updated Pages
Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

What is the common property of the oxides CONO and class 10 chemistry JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

If four points A63B 35C4 2 and Dx3x are given in such class 10 maths JEE_Main

The area of square inscribed in a circle of diameter class 10 maths JEE_Main

Other Pages
A boat takes 2 hours to go 8 km and come back to a class 11 physics JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

In the ground state an element has 13 electrons in class 11 chemistry JEE_Main

According to classical free electron theory A There class 11 physics JEE_Main

Differentiate between homogeneous and heterogeneous class 12 chemistry JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main