
The equation
(a) No solution.
(b) Only one solution
(c) Two solutions
(d) Three solutions
Answer
156.6k+ views
Hint: Start by using the identity
Complete step-by-step answer:
Before starting with the solution to the above question, we will first talk about the required details of different inverse trigonometric ratios. So, we must remember that inverse trigonometric ratios are completely different from trigonometric ratios and have many constraints related to their range and domain.
Now let us draw the graph of
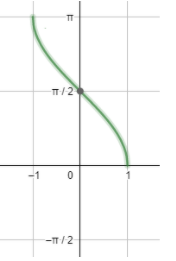
So, looking at the above graphs, we can draw the conclusion that
Now moving to the solution to the above question, we will start with the simplification of the expression given in the question.
We know that
Now as
Therefore, the answer to the above question is option (a).
Note: Students generally get confused in the range of different inverse trigonometric functions as they very much look the same but are far different. Also, it is important that you check the domain of each inverse trigonometric term in the equation separately before reporting a value of x that satisfies the equation.
Complete step-by-step answer:
Before starting with the solution to the above question, we will first talk about the required details of different inverse trigonometric ratios. So, we must remember that inverse trigonometric ratios are completely different from trigonometric ratios and have many constraints related to their range and domain.
Now let us draw the graph of

So, looking at the above graphs, we can draw the conclusion that
Now moving to the solution to the above question, we will start with the simplification of the expression given in the question.
We know that
Now as
Therefore, the answer to the above question is option (a).
Note: Students generally get confused in the range of different inverse trigonometric functions as they very much look the same but are far different. Also, it is important that you check the domain of each inverse trigonometric term in the equation separately before reporting a value of x that satisfies the equation.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

Chemical Properties of Hydrogen - Important Concepts for JEE Exam Preparation

JEE Energetics Important Concepts and Tips for Exam Preparation

JEE Isolation, Preparation and Properties of Non-metals Important Concepts and Tips for Exam Preparation

Trending doubts
Degree of Dissociation and Its Formula With Solved Example for JEE

Geostationary Satellites and Geosynchronous Satellites - JEE Important Topic

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

What is Hybridisation in Chemistry?

Photoelectric Effect and Stopping Potential with Work Function and Derivation for JEE

Best Books for JEE Main and Advanced 2025 - Comprehensive Study Materials




