Question

The engine of an unpowered toy train is rolling at a constant speed on a level track, as shown in fig. The engine collides with a stationary toy truck, and joins with it. Before the collision toy engine is travelling at $0.32m{s^{ - 1}}$. The mass of the engine is $0.5kg$
(A) Calculate the momentum of the toy engine before collision.
(B) The mass of the truck is $0.3kg$. Using the principle of conservation of momentum, calculate the speed of the joined engine and truck immediately after collision.

Answer 1

Hint: Momentum can be defined as the tendency of an object to remain in its state of motion. It is the product of mass and velocity. Since it depends on velocity as well as the direction of motion it is a vector quantity.
According to the Law of Conservation of Momentum, when two or more bodies in an isolated system collide upon each other the net momentum remains constant unless an external force is applied. Thus momentum can neither be created nor be destroyed. This is directly related to Newton's third law of motion.

Complete step by step solution:
We are given that,
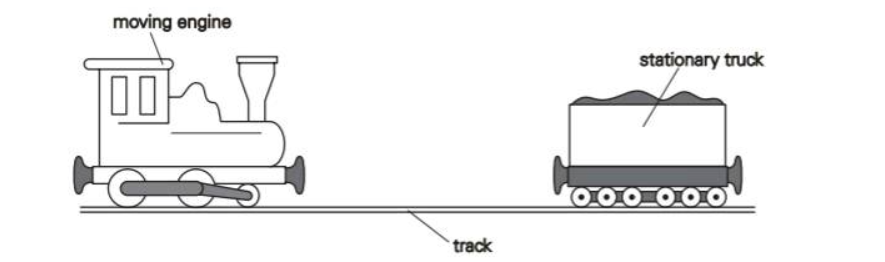
${v_1} = 0.32m{s^{ - 1}}$ ${m_1} = 0.5kg$
Where ${v_1}\& {m_1}$ are the velocity and mass of the toy engine.
We know that momentum is the product of mass and velocity.
${P_1} = {m_1}{v_1}$
$ \Rightarrow P = 0.5 \times 32$
$ \Rightarrow P = 0.16kgm{s^{ - 1}}$
So the answer for option A is $0.16kgm{s^{ - 1}}$
Moving on to the second part of the question, we are given that
${m_2} = 0.3kg$ ${v_2} = ?$
Where ${m_2}\& {v_2}$ are the mass and velocity of the truck respectively.
According to law of conservation of momentum,
${P_i} = {P_f}$
$ \Rightarrow {P_i} = \left( {{m_{engine}} + {m_{truck}}} \right)v$
$ \Rightarrow 0.16 = \left( {0.5 + 0.3} \right)v$
$ \Rightarrow {v_f} = 0.2m{s^{ - 1}}$
Additional Information:
Angular momentum is defined as the product of moment of inertia and angular velocity. It is property of a rotating body.
Law of conservation of angular momentum states that momentum is well conserved as long as there is no application of external torque on it. This principle is used in electric generators and aircrafts.

Note:
Moment of Inertia: It can be defined as the quantity expressed by body resisting angular acceleration which is the sum of product of mass of every particle with its square of a distance from the axis of rotation or in simple words it decides the amount of torque needed for angular acceleration in rotational axis.
Moment of inertia depends on density of material, shape and size of the material, axis of rotation of the body.