
The coordination number of an atom in a fcc lattice is:
(A) 12
(B) 8
(C) 4
(D) 6
Answer
444.4k+ views
1 likes
Hint:
In a fcc lattice there is one constituent particle at each corner and one at the centre of each face. The number of nearest neighbours is called coordination number.
Complete step by step answer:
A face centred cubic unit cell contains atoms at all the corners of the unit cell and at the centre of all the faces of the cube.
Coordination number is the number of other atoms that each atom in a crystalline solid contacts. In simple words the number of nearest neighbours of an atom forms the coordination number.
In an fcc unit cell the total number of atoms is:
(a) 8 corner atoms with each one contributing
(b) 6 face centre atoms with each one contributing
So, a total of 4 atoms in an fcc unit cell.
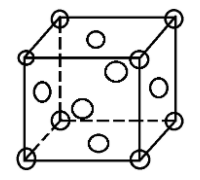
In an fcc structure atoms are packed as closely to each other as possible and the 4 atoms occupy 74% of the volume of the unit cell. It has unit cell vectors a = b = c and interaxial angles α = β = γ = 90
In an fcc lattice the face centre atoms are the nearest atoms and one corner atom is surrounded by 4 faces in the x-plane, 4 faces in the y-plane and 4 faces in the z-plane. So, every corner atom is surrounded by (4 × 3) = 12 face centre atoms. Since they are the nearest they form the coordination number.
Also if we consider the atom at the face centre, it is surrounded by 4 corner atoms of its own plane and the 8 adjacent face centred atoms (4 from above and 4 from below). This also makes the coordination number to be 12.
Hence, the coordination number of an fcc lattice is 12.
So, the correct option is (A).
Note:
While imagining the structure of the fcc unit cell, always be precise regarding the position of the atoms. Also remember that there are 4 faces associated with the 3 axes.
In a fcc lattice there is one constituent particle at each corner and one at the centre of each face. The number of nearest neighbours is called coordination number.
Complete step by step answer:
A face centred cubic unit cell contains atoms at all the corners of the unit cell and at the centre of all the faces of the cube.
Coordination number is the number of other atoms that each atom in a crystalline solid contacts. In simple words the number of nearest neighbours of an atom forms the coordination number.
In an fcc unit cell the total number of atoms is:
(a) 8 corner atoms with each one contributing
(b) 6 face centre atoms with each one contributing
So, a total of 4 atoms in an fcc unit cell.

In an fcc structure atoms are packed as closely to each other as possible and the 4 atoms occupy 74% of the volume of the unit cell. It has unit cell vectors a = b = c and interaxial angles α = β = γ = 90
In an fcc lattice the face centre atoms are the nearest atoms and one corner atom is surrounded by 4 faces in the x-plane, 4 faces in the y-plane and 4 faces in the z-plane. So, every corner atom is surrounded by (4 × 3) = 12 face centre atoms. Since they are the nearest they form the coordination number.
Also if we consider the atom at the face centre, it is surrounded by 4 corner atoms of its own plane and the 8 adjacent face centred atoms (4 from above and 4 from below). This also makes the coordination number to be 12.
Hence, the coordination number of an fcc lattice is 12.
So, the correct option is (A).
Note:
While imagining the structure of the fcc unit cell, always be precise regarding the position of the atoms. Also remember that there are 4 faces associated with the 3 axes.
Recently Updated Pages
Difference Between Alcohol and Phenol

Classification of Drugs Based on Pharmacological Effect, Drug Action

JEE Main Mock Test Series Class 12 Chemistry for FREE

JEE Atomic Structure and Chemical Bonding important Concepts and Tips

JEE Amino Acids and Peptides Important Concepts and Tips for Exam Preparation

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Degree of Dissociation and Its Formula With Solved Example for JEE

Displacement-Time Graph and Velocity-Time Graph for JEE

Electrical Field of Charged Spherical Shell - JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

NCERT Solutions for Class 12 Chemistry Chapter 1 Solutions

NCERT Solutions for Class 12 Chemistry Chapter 2 Electrochemistry

NCERT Solutions for Class 12 Chemistry Chapter 6 Haloalkanes and Haloarenes

Solutions Class 12 Notes: CBSE Chemistry Chapter 1




